Class IX Maths Solutions WBBSE Chapter 11 Statistics Exercise 11.1

Question 1. I have written the number of children belonging to each of 40 families in our locality below

1	2	6	5	1	5	1	3	2	6
2	3	4	2	0	4	4	3	2	2
0	0	1	2	2	4	3	2	1	0
5	1	2	4	3	4	1	6	2	2

 

I prepare a frequency distribution table of the above-given data whose classes are 0-2, 2-4,…….., etc.

Solution: Frequency distribution table

Class Interval	Class Size	Length of Class	Tally Mark	Frequency
0-2	0-2	2	I	11
2-4	2-4	2	II	17
4-6	4-6	2	llll	9
6-8	8-Jun	2	III	3
			Total	40

 

Read and Learn More WBBSE Solutions For Class 9 Maths

Question 2. Given below are the marks obtained by 40 students in a test of school:

34	27	45	21	30	40	11	47	1	15
3	40	12	47	48	18	30	24	25	28
32	31	25	22	27	41	12	13	2	44
43	7	9	49	13	19	32	39	24	3

 

I construct a frequency distribution table of these marks by taking classes 1-10, 11-20, ………, 41-50.

Solution: Frequency distribution table

Class	Tally mark	Frequency
1-10	I	6
11-20	III	8
21-30	I	11
31-40	II	7
41-50	III	8
	Total	40

 

Question 3. There are many oranges in a basket. From this basket, by aimlessly taking 40 oranges, I wrote below their weights (gm):

45, 35, 30, 55, 70, 100, 80, 110, 80, 75, 85, 70, 75, 85, 90, 75, 90, 30, 55, 45, 40, 65, 60, 50, 40, 100, 65, 60, 40, 100, 75, 110, 30, 45, 84, 70, 80, 95, 85, 70.

Now, I construct a frequency distribution table and a less-than-type cumulative frequency distribution table for the above-given data.

Solution: Maximum weight = 110 gm
Minimum weight = 30 gm
∴ Width = 110-30=80 gm
Size of class = 10

Class	Tally mark	Frequency	Cumulative Frequency
30-40	llll	4	4
40-50	I	6	4 + 6=10
50-60	III	3	10 + 3=13
60-70	llll	4	13 + 4=17
70-80	III	8	17 + 8 = 25
80-90	II	7	25 + 7 = 32
90-100	III	3	32 + 3 = 35
100-120	III	3	35 + 3 = 38
110-120	II	2	38 + 2 = 40
	Total	40

 

Note: If the same value occurs in two classes put the value in a higher class.

Class IX Maths Solutions WBBSE

Question 4. Mitali and Mohidul wrote below the amount of money of electricity bills of the 45 houses of their village for this month:

116, 127, 100, 82, 80, 101, 91, 65, 95, 89, 75, 92, 129, 78, 87, 101, 65, 52, 59, 65, 95, 108, 115, 121, 128, 63, 76, 130, 116, 108, 118, 61, 129, 127, 91, 130, 125, 101, 116, 105, 92, 75, 98, 65, 110.

I construct a frequency distribution table for the above data.

Solution: Frequency distribution table

Class	Tally Mark	Frequency
50-60	II	2
60-70	I	6
70-80	llll	4
80-90	llll	4
90-100	II	7
100-110	II	7
110-120	I	6
120-130	II	7
130-140	II	2
	Total frequency	45

 

Question 5. Maria has written the ages of 300 patients of a hospital in the table given below:

Ages (years)	10-20	20-30	30-40	40-50	50-60	60-70
The number of patients	80	40	50	70	40	20

 

I construct a more than type cumulative frequency distribution table for the above data.

Solution: More than type cumulative frequency distribution label

Age (years) Class	No. of patients Frequency	Cumulative frequency
10-20	80	220 + 80 = 300
20-30	40	180 + 40 = 220
30-40	50	130 + 50 = 180
40-50	70	60 + 70 = 130
50-60	40	20 + 40 = 60
60-70	20	20
Total	300

 

Question 6. Let us observe the following cumulative frequency distribution table and construct a frequency distribution table:

Classes	Below 10	Below 20	Below 30	Below 40	Below 50	Below 60
The number of students	17	22	29	37	50	60

 

Solution: Frequency distribution table

Class no. of students	Frequency	Cumulative frequency
10	17	17
10-20	22-17 = 5	22
20-30	29-22 = 7	29
30-40	37-29 =8	37
40-50	50-37 = 13	50
50-60	60-50 = 10	60

 

Question 7. Let us observe the following cumulative frequency distribution table and construct the frequency distribution table :

Marks obtained	The number of students
More than 60	0
More than 50	16
More than 40	40
More than 30	75
More than 20	87
More than 10	92
More than 0	100

 

Class IX Maths Solutions WBBSE

Solution: Frequency distribution table

Marks	Class	The no. of students	Frequency
More than 60	More than 60	0	0
More than 50	50-60	16	16-0 = 16
More than 40	40-50	40	40-16 = 24
More than 30	30-40	75	75-40 = 35
More than 20	20-30	87	87-75 = 12
More than 10	10-20	92	92-87 = 5
More than 0	0-10	100	100-92 = 8

 

Question 8.

1. Which one of the following is a graphical (pictorial) representation of statistical data?

1. Line graph
2. Raw data
3. Cumulative frequency
4. Frequency.

Solution: 1. Line graph

2. The range of the data 12, 25, 15, 18, 17, 20, 22, 26, 6, 16, 11, 8, 19, 10, 30, 20, 32 is

1. 10
2. 15
3. 18
4. 26

Solution: Greatest value = 32
Minimum value = 6
Range 326 = 26

∴ 4. 26

3. The class size of classes 1-5, 6-10 is

1. 4
2. 5
3. 4.5
4. 5.5

Solution: Class size=5-110-6=4
∴ 1. 4

Class 9 Maths WB Board

4. In a frequency distribution table, the mid-points of the classes are 15, 20, 25, 30 ……. respectively. The class having a mid-point as 20 is

1. 12.5-17.5
2. 17.5-22.5
3. 18.5 21.5
4. 19.5 20.5

Solution:  20-15-25-20-30-25=5
∴ Class size = 5
Class size of 1st class = 17.5-12.5 = 5

Mid value = \(\frac{12.5+17.5}{2}=\frac{30.0}{2}=15 \neq 20\)

In the class, 17.5-22.5, class size = 22.5 17.5 = 5

Mid value = \(\frac{12.5+17.5}{2}\) = 20

The mid value of the last two classes is 20, but the class length is not 5.

∴ 2. 17.5 22.5

5. In a frequency distribution table if the mid-point of a class is 10 and the class size of each class is 6; the lower limit of the class is

1. 6
2. 7
3. 8
4. 12

Solution: Let upper limit of = x and lower limit = y.

According to the problem, 1st conditions: \(\frac{x+y}{2}=10\)

or, x + y = 20 …..(1)

According to the problem, 2nd conditions:
or, x – y = 6 ….(2)

Subtracting (2) from (1), 2y = 14

or, \(y=\frac{14}{2}=7\)

∴ Lower limit of the class
∴ 2. 7

Question 9.

1. In a continuous frequency distribution table if the mid-point of a class is m and the upper-class boundary is u, then let us find out the lower-class- boundary.

Solution: Mid value of the class = m
Upper boundary of the class = u
∴ Mid value = m
∴ Lower class boundary= 2 x mid value =2 x m-u = 2m – u

2. In a continuous frequency distribution table, if the mid-point of a class is 42 and class size is 10, then let us write the upper and lower limits of the class.

Solution: Let the upper-class boundary be x and the lower boundary be y. According to the problem, 1st condition

According to the problem, 1st condition \(\frac{x+y}{2}=42\)

or, x + y = 84 ……(1)

According to the problem, 2nd condition 2x = 94

or, \(x=\frac{94}{2}=47\)

Putting the value of x in equation (1), 47 + y = 84
⇒ y= 84-47 = 37
∴ Upper limit = 47
Lower limit 37

Class 9 Maths WBBSE

3. Let us write the frequency density of the first class of the frequency distribution table.

Class- limit	70-74	75-79	80-84	85-89
Frequency	3	4	5	8

Solution: 1st class = 70-74
Class size the 1st class = 74-70 = 4
Frequency of the 1st class = 3

Frequency dencing of the 1st class \(=\frac{\text { Frequency }}{\text { Classsize }}\)

\(=\frac{3}{4}\)= 0.75

4. Let us write the frequency density of the last class

Class- limit	70-74	75-79	80-84	85-89
Frequency	3	4	5	8

 

Solution: Last class = 85-89
Frequency = 8
Total frequency=3+4+5+8=20

∴ Frequency of the class \(y=\frac{\text { Frequency of the class }}{\text { Total frequency }}\)

\(\begin{aligned}
& =\frac{8}{20} \\
& =\frac{2}{5}=0.4
\end{aligned}\)

 

5. Let us write from the following examples which one indicates attribute and which one indicates variable.

1. Population of the family.
2. Daily temperature.
3. Educational value.
4. Monthly income.
5. Grade obtained in Madhyamik Examination.

Solution:
1. Population of the family – Variable
2. Daily temperature – Variable
3. Educational – Attribute
4. Monthly income – Variable
5. Grade obtained in – Attribute

Class 9 Maths WBBSE Chapter 11 Statistics Exercise 11.2

 

Question 1. I construct the frequency polygon for the following marks obtained by 75 learners of Pritha’s school:

Marks obtained	30	40	50	60	70	80
Number of students	12	18	21	15	6	3

 

In the graph paper, taking suitable measures along horizontal and vertical lines, the points (20, 0), (30, 12), (40, 18), (50, 21), (60, 15), (70, 6), (80, 3) and (90, 0) are plotted on the graph paper and then I draw the frequency polygon by adding them.

Solution: Along x-axis one side of the smallest square = 1 mark & along y-axis two sides of the smallest square 1 student. And putting the points and joining then ABCDEFGH frequency polygon is obtained.

 

 

Question 2. I draw the frequency polygon for the following frequency distribution table:

Classes	0-5	5-10	10-15	15-20	20-25	25-30
Frequency	4	10	24	12	20	8

 

Solution:

Class	Mid value	Frequency
0-5	2.5	4
5-10	7.5	10
10-15	12.5	24
15-20	17.5	12
20-25	22.5	20
25-30	27.5	8

 

 

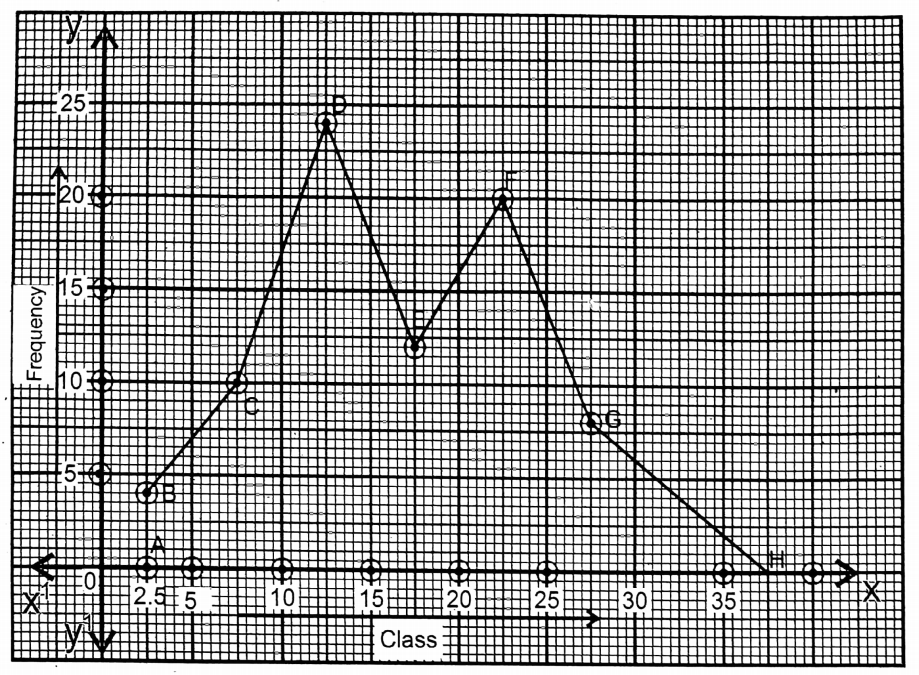
1. Class along x-axis & frequency along y-axis.
2. Taking the points of mid-value & frequency points are plotted, B(2.5, 4) C(7.5, 10), D(12.5, 24), E(17.5, 12), F(22.5, 20), G(27.5, 8)
3. Joining A, B, C, D, E, F, G, H with a scale we get, ABCDEFGH a frequency polygon.

Question 3. I write below in tabular form the daily profit of the 50 shops of the village of Bakultala:

Daily profit (Rs.)	0-50	50-100	100-150	150-200	200-250
Number of shops	8	15	10	12	5

 

 

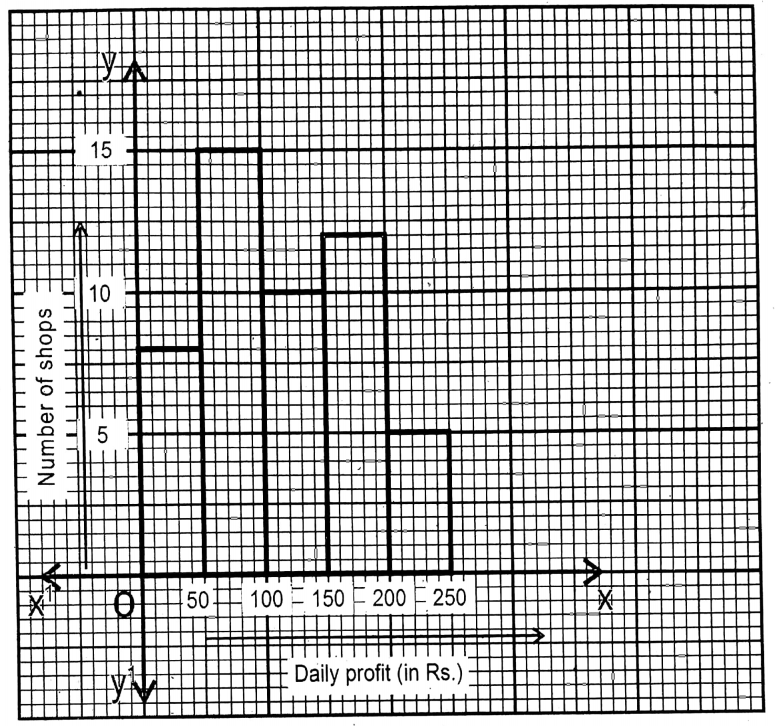
Solution: Along x-axis – Daily profit
Along y-axis No. of shops

One side of the smallest square along x-axis = Rs. 10 (Profit); two sides of the smallest square along y-axis = 1 shop.

I draw the histogram for the above data.

Question 4. By measuring, Mita wrote the heights of her 75 friends of their school in the table given below : I draw the histogram of the data collected by Mita.

Height (cm.)	136-142	142-148	148-154	154-160	160-166
Number of friends	12	18	26	14	5

 

Solution: Along x-axis – height (in cm) &
along y-axis No. of friends

unit:- 5 sides of the smallest square along x-axis = 6 cm & 1 side of the smallest square along y-axis = 1 friend. Putting the value, I draw the histogram for the given data

 

 

Question 5. In our locality, by collecting the number of Hindi-speaking people between the  ages of 10 years to 45 years, I write them in the table given below:

Age (in years)	10-15	16-21	22-27	28-33	34-39	40-45
Number of Hindi-speaking people	8	14	10	20	6	12

 

Solution: First make a frequency distribution table

Class (year)	Class boundary	Length of class	Frequency
10-15	9.5 – 15.5	6	8
16-21	15.5 – 21.5	6	14
22-27	21.5-27.5	6	10
28-33	27.5 – 33.5	0.6	20
34-39	33.5 – 39.5	6	6
40-45	39.5 – 45.5	6	12

 

Along the x-axis – Age (year) & Along the y-axis – No. of Hindi-speaking people Unit 5 sides of the smallest square along the x-axis & 2 sides of the smallest square along the y-axis = 1 people

Putting the value, I draw the histogram.

 

 

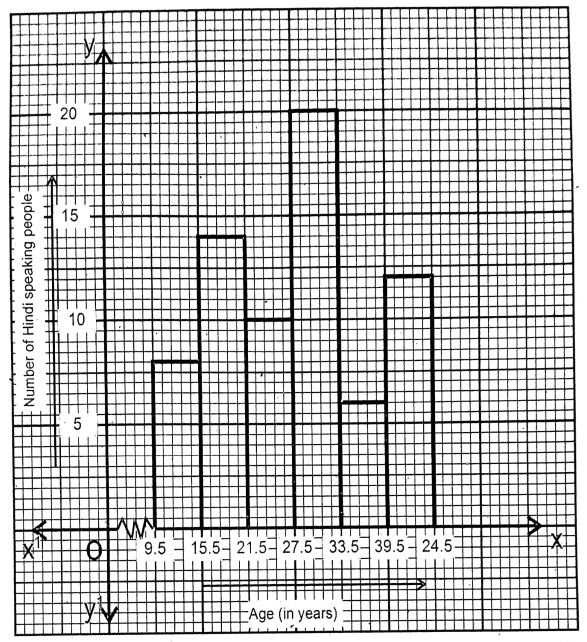
Question 6. I draw the histogram of the frequency distribution table given below:

Class	1-10	11-20	21-30	31-40	41-50	51-60
Frequency	8	3	6	12	2	7

 

Solution: Frequency distribution table

Class	Class-boundary	Length of Class	Frequency
1-10	0.5 – 10.5	10	8
11-20	10.5-20.5	10	3
21-30	20.5 – 30.5	10	6
31-40	30.5 – 40.5	10	12
41-50	40.5 – 50.5	10	2
51-60	50.5 – 60.5	10	7

 

Along x-axis-class boundary
& Along y axis – Frequency along x-axis
unit one side of the smallest square = 2 units
& Four side of the smallest square along y-axis = 1 unit
Putting the value, Histogram is obtained

 

 

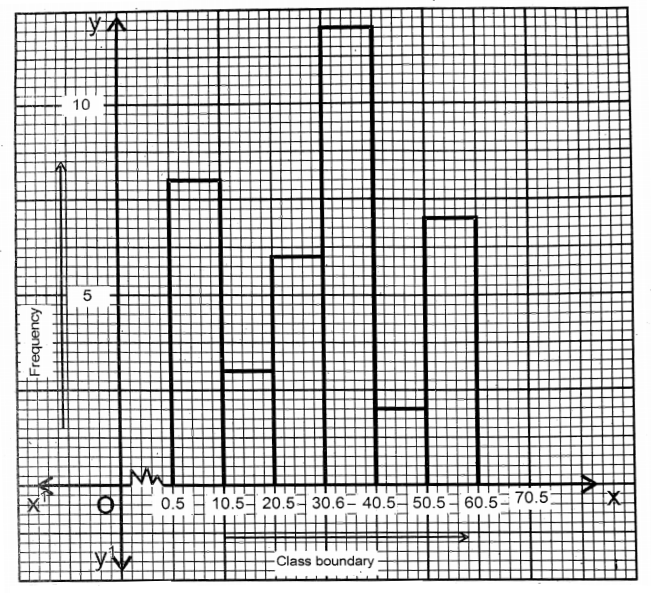
Question 7. By drawing the histogram, I draw the frequency polygon of the frequency distribution table given below:

Amount of subscriptions (Rs.)	20	25	30	35	40	45	50
Number of Members	20	26	16	10	418	6

 

Solution: Frequency distribution table

Class (Rs.)	Mid value	Frequency (no. of members)
17.5-22.5	20	20
22.5-27.5	25	26
27.5-32.5	30	16
32.5-37.5	35	10
37.5-42.5	40	4
42.5-47.5	45	18
47.5-52.5	50	6

 

Along x-axis Amount of Subscription (Rs.)
& Along y-axis Number of members (Frequency)

Unit 2 sides of the smallest square along the x-axis = Re. 1; 2 sides of the smallest square along y-axis = 1 member.

Now I draw the histogram.

Now for the drawing of frequency polygon just before the first class interval I take class interval 12.5 17.5 and just after class interval, I take class interval 52.5 –
57.5. The frequencies of these two class intervals are 0.

(30,16), (35,10), (40,4), (45,18), (50-6) & (55,0) successively with straight lines, I have drawn the frequency polygon ABCDEFGHI.

Class 9 Math Solution WBBSE In English

 

 

Question 8. I draw the histogram for the following frequency distribution table:

Number of children	0	1	2	3	4	5
Number of families		85	50	25	15	5

 

Hints: At first, by the exclusive class method the statistical data will be constructed as a frequency distribution table with class boundaries given below:

Number of children	0-1	1-2	2-3	3-4	4-5	5-6
Number of families	120	85	50	25	15	5

 

Solution: Frequency distribution table

Class (No. of children)	Frequency (No. of families)
0-1	120
1-2	85
2-3	50
3-4	25
4-5	15
5-6	5

 

Along x-axis – No of children & Along y-axis – Number of families

Unit: 5 sides of the smallest square along x-axis = 1 child. 2 sides of the smallest square along y-axis = 5 families

Putting the values Histogram is obtained.

 

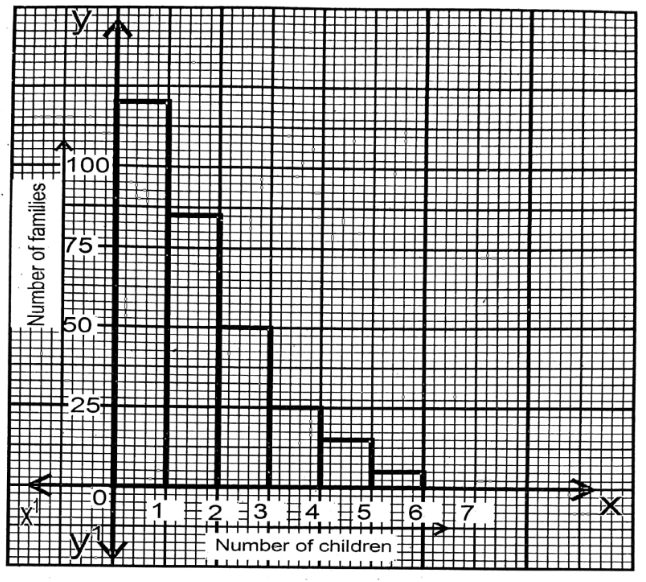
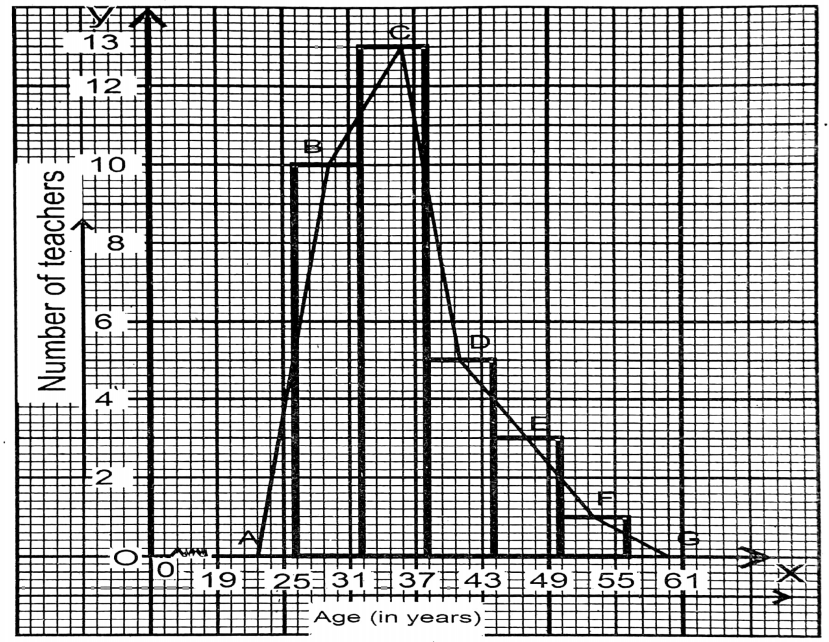
Question 9. I have written the ages of 32 teachers of Primary Schools in the village of Virsingha in a table given below:

Ages (years)	25-31	31-37	37-43	43-49	49-55
Number of teachers	10	13	5	3	1

 

Now, we have to draw a histogram and frequency polygon with the given data graphically:

Solution: Frequency distribution table

Class Age (years)	Class boundary	Mid value	Length	Frequency(No. of teachers)
25-31	25-31	28	6	10
31-37	31 -37	34	6	13
37-43	37-43	40	6	5
43-49	43-49	-46	6	3
49-55	49-55	52	6	1

 

Along x-axis Age (years) & Along y-axis – The number of teachers

Unit: 1 side of the smallest square along x – axis = 1 year and 5 sides of the smallest square along the y-axis = 1 teacher.

Now I draw the histogram.

Class 9 Math Solution WBBSE In English

Now for drawing frequency polygon just before 1st class interval, I take a class interval of 19-25, and just after the last class interval, I take another class interval of 55- 61. The frequencies of these two class intervals are 0 (Zero).

Then by joining the points (22,0), (28,10), (34,13), (40,5), (46,3), (52,1), and (58,0) successively with straight lines, frequency polygon ABCDEFG is obtained.

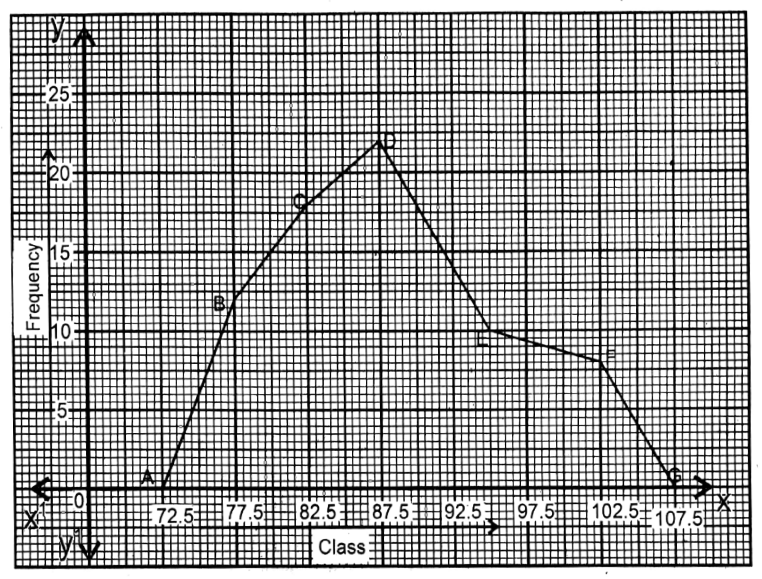
Question 10. I draw the frequency polygon for the following frequency distribution table:

Class	75-80	80-85 I	85-90	90-100	100-105
Frequency	12	18	22	10	8

Solution: Frequency distribution table

Class	Mid value	Frequency
75-80	77.5	12
80-85	82.5	18
85-90	87.5	22
90-100	95	10
100-105	102.5	8

 

Along x-axis Class & along y-axis Frequency

Unit: 2 sides of the smallest square along x-axis = 1 unit and 2 sides of the smallest square along the y-axis= 1 unit.

Now for drawing frequency polygon just before 1st class interval I take a class interval of 70-75 and just after the last class interval, I take another class interval of 105- 110. The frequencies of the two class intervals are 0 (Zero).

Then by doing the points (72.5,0) & (107.5,0) successively with a straight line the freguency polygon ABCDEFG is obtained.

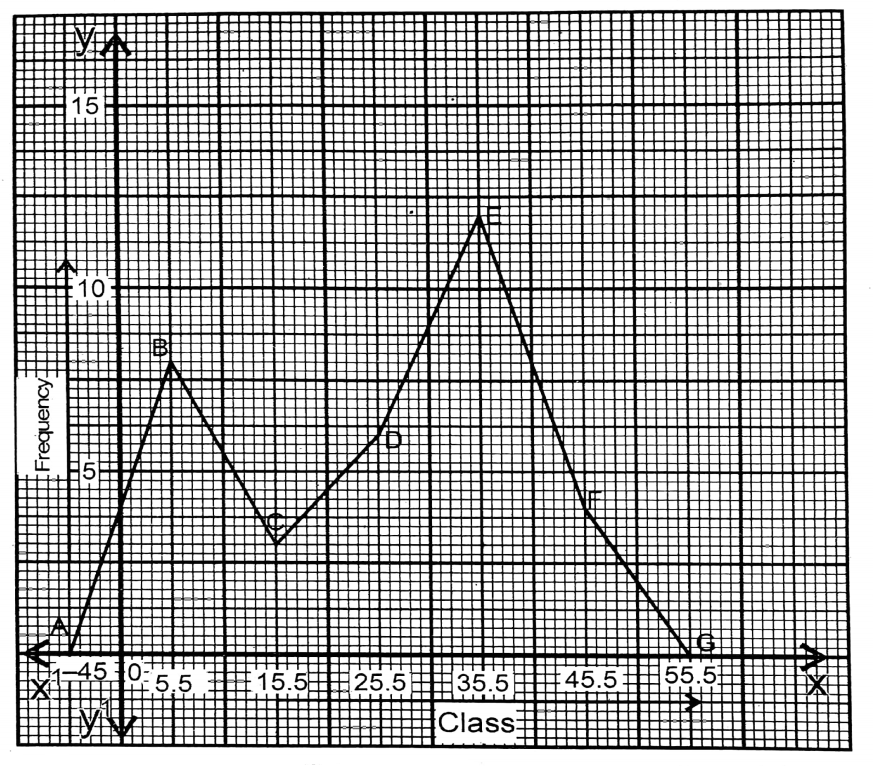
Question 11. I draw the frequency polygon for the following frequency distribution table.

Class	1-10	11-20	21-30	31-40	41-50
Frequency	8	3	6	12	4

 

Solution: Frequency distribution table

Class	Class boundary	Mid Value	Frequency
1-10	0.5-10.5	5.5	8
11-20	10.5-20.5	15.5	3
21-30	20.5-30.5	25.5	6
31-40	30.5 – 40.5	35.5	12
41-50	40.5 – 50.5	45.5	4

 

Along x-axis – Class & along y-axis – Frequency

Unit: 1 side of the smallest square along x-axis = 1 unit and 4 sides of the smallest square along y-axis = 1 unit

Now for drawing frequency polygon just before 1st class interval I take a class interval of -10,-0 and just after the last class interval, I take another class interval 51- 60.

The frequencies of these two class intervals are 0 (Zero). Then by joining the points (-4.5,0), (5.5,8), (15.5,3), (25.5,6), (35.5,12), (45.5,4), and (55.5,0) successively with straight lines we obtained ABCDEFG, frequency polygon.

 

 

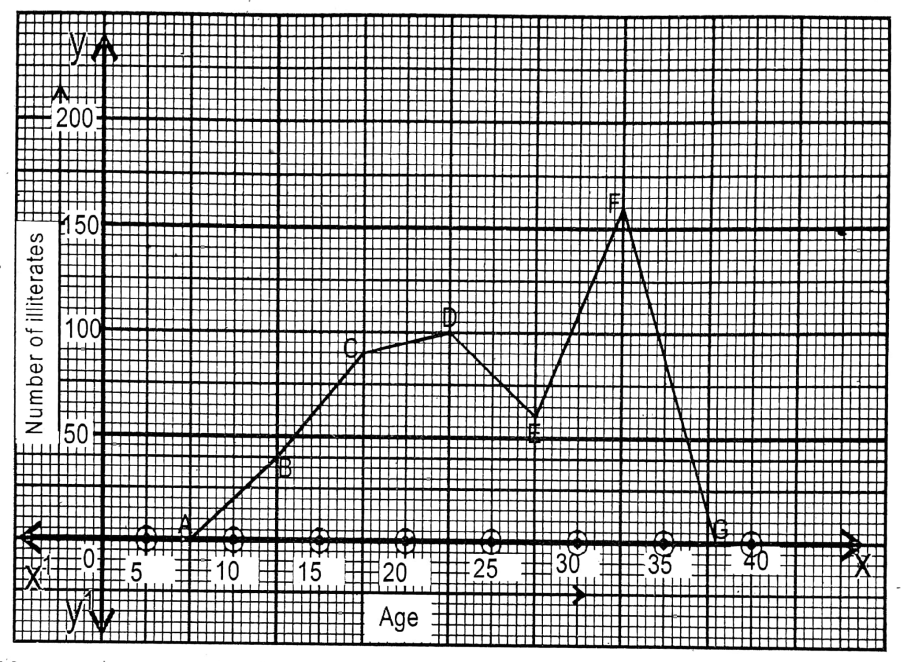
Question 12. A special drive will be taken for women’s literacy in total in our village. For this reason, we have collected the following data:

Age	10-15	15-20	20-25	25-30	30-35
Number of illiterates	40	90	too	60	160

 

Solution: To draw the frequency polygon

Frequency distribution table

Class Age (years)	Mid value	Frequency (No. of illiterates)
10-15	12.5	40
15-20	17.5	90
20-25	22.5	100
25-30	27.5	60
30-35	32.5	160

 

Along x-axis-Class (Age) & along y-axis is the Number of illiterates

Unit: 2 sides of the smallest square along x axis = 1 year & 1 side of the smallest square along the y-axis = 5 number of illiterates.

Now for drawing frequency polygon just before 1st class interval I take a class interval 5-10 and just after the last class interval, I take another ass interval of 35-40.

The frequencies of these two class intervals are 0 (Zero). Then by joining the points (7.5,0), (12.5,40), (17.5,90), (22.5,100), (27.5,60), (32.5,160), and (37.5,0) straight lines we get the required frequency polygon ABCDEFG.

 

Question 13. I have written in the following the frequency of the number of goals given by the teams in our Kolkata football league in the previous month. I draw the frequency polygon for the representation of the data.

Scores	0	1	2	3	4	5	6
Frequency	15	20	12	8	6	3	1

 

Solution: Frequency distribution table

Score	Frequency
0	15
1	20
2	12
3	8
4	6
5	3
6	1

 

Along x-axis-score (goal) & along y-axis – Frequency

Unit 10 sides of the smallest square along x-axis = 1 unit and 2 sides of the smallest square along y-axis = 1 unit.

Now for drawing frequency polygon just before 1st class interval I take a class interval of -1,-0 and just after the last class interval, I take another class interval of 7-0.

The frequencies of these two class intervals are 0 (Zero). Then by joining the points (1,0), (0,15), (1,20), (2,12), (3,0), (4,6) and (5,3), (6,1) & (7,0) successively with straight lines we obtained frequency polygon ABCDEFGHI,

 

 

Question 14.  Let us discuss

1. Each of the area of each of the rectangle of a histogram is proportional to

1. The mid-point of that class
2. The class size of that class
3. The frequency of that class
4. The cumulative frequency of that class

Solution: 3. The frequency of that class

2. A frequency polygon is drawn

1. Upper limit of the class
2. Lower limit of the class
3. Mid-value of the class
4. Any value of the class

Solution 3. Mid-value of the class

3. To draw a histogram, the class

1. Along y-axis
2. Along x-axis
3. Along x-axis and y-axis both
4. In between x-axis and y-axis

Solution: 2. Along x-axis

4. In the case of drawing a histogram,

1. Frequency
2. Class boundary
3. Range.
4. Class size.

Solution: 4. Class size.

5. A histogram is the graphical representation of grouped data whose class- boundary and frequency are taken respectively,
1. Along the vertical axis and horizontal axis,
2. Only along the vertical axis,
3. Only along horizontal axis,
4. Along the horizontal axis and vertical axis.

Solution: 4. Along the horizontal axis and vertical axis.

 

 

 

Chapter 17 Theorems On Concurrence Exercise 17

Question 1. The bisectors of ∠B and ∠C of ABC intersect each other at point I. Let us prove that \(\angle B I C=90^{\circ}+\frac{\angle B A C}{2}\)

Solution:

 

 

∵ Bl and Cl respectively are the bisectors of ∠ABC and ∠ACB.

∴ ∠IBC = \(\frac{1}{2}\) ∠ABC and ∠ICB = \(\frac{1}{2}\) ∠ACB

In ΔBIC, ∠BIC+∠IBC + ∠ICB = 180°

or, ∠BIC+ \(\frac{1}{2}\) ∠ABC + \(\frac{1}{2}\) ∠ACB=180°

or, ∠BIC+ \(\frac{1}{2}\) + (∠ABC + ∠ACB) = 180°

or, ∠BIC + \(\frac{1}{2}\) (∠ABC+∠ACB+ ∠BAC) = 180° + \(\frac{1}{2}\) ∠BAC

Adding \(\frac{1}{2}\) ∠BAC on both sides)

or, ∠BIC+ \(\frac{1}{2}\)  x 180° = 180° + \(\frac{1}{2}\) ∠BAC

or, ∠BIC = 90° + \(\frac{\angle B A C}{2}\) Proved

Read and Learn More WBBSE Solutions For Class 9 Maths

Question 2. If the lengths of the three medians of a triangle are equal, let us prove that the triangle is an equilateral triangle.

Solution: Let ABC is a triangle whose three medians AD, BE and CF cut each other at O and AD = BE = CF.
To prove ABC is an equilateral triangle

 

 

Proof: Centroid divides the median in the ratio of 2: 1.

∴ AO = \(\frac{2}{3}\), BO = \(\frac{2}{3}\) BE and CO = \(\frac{2}{3}\) CF

∵ AD = BE = CF (∵ Lengths of 3 medians of a triangle are equal.)

∴ \(\frac{2}{3}\) AD = \(\frac{2}{3}\) BE = \(\frac{2}{3}\) CF

∴ AO = BO=CO
∴ AD – AO = BE – BO = CF-CO
or, OD = OE = OF

In ΔAOF and ΔCOD,
OF = OD, AO = CO
∠AOF = VOA ∠COD

∴ ΔAOF ≅ ΔCOD (S-A-S congruency)
∴ AF = CD
or, 2AF 2CD

∴ AB = BC   ……(1)

Similarly, from ABOD and AAOE, it can be proved that AE = BD.
or, 2AE = 2BD

∴ AC = BC ….(2)

∴ In ΔABC AB = BC = CA
∴ ΔABC is an equilateral triangle. Proved

Question 3. Let us prove that in an equilateral triangle, circumcentre, incentre, centroid, and orthocentre will coincide.

Solution:

 

 

 

ABC is an equilateral triangle.
To prove cireum center, incentre, other center & centroid will coincide.

Proof: From A, B & C perpendiculars are drawn on BC, CA & AB. The perpendiculars AD, BE & CF meet at G

In ΔABD and ΔACD,
1. AB = AC
2. ∠ABD = ∠ACD
3. ∠ADB = ∠ADC

∴ ΔABD ≅ ΔACD
∴ ∠BAD = ∠CAD

∴ AD, ∠BAC is the bisector of ∠BAC. Similarly, BE & CF are the bisectors of ∠ABC & ∠ACB respectively. G is the centroid of the triangle. BD
∴ AD is the median

Similarly, BE & CF are two medians.
∴ G is the centroid.

Three medians are perpendicular on respective sides. C is the circumcentre of the triangle. (Proved)

Question 4. AD, BE and CF are three medians of a triangle ABC. Let us prove that the centroid of ABC and DEF are the same point.

Solution:

 

 

To prove the centroid of ΔABC and ΔDEF are the same point.

Proof: Three medians AD, BE & CF meet at G.
∴ G is the centroid of ΔABC

In ΕABC, F & E are the midpoints of AB & AC respectively.
∴ EF II BC, i.e., EF II BD.

Similarly, DE II BF
∴ BDEF is a parallelogram.

Its diagonals BE & DF intersect each other at N.
∴ N is the mid-point of DF.

Similarly, in parallelogram AFDE, M is the midpoint of EF. The two medians EN & DM meet each other at G.
∴ G is the centroid of ADEF.
∴The centroid of ΔABC & ΔDEF is the same point.

Question 5. Let us prove that the two medians of a triangle are together greater than the third median.

Solution:

 

 

 

In ΔABC, AD, BE & CF are the medians
To prove, BE+ CF > AD

Produce AD to H such that GD = DH
B, H and C, H are joined.

Proof: In □BHCG, BD = CD (∵ AD is median)
GD = DH (By construction)
BC & GH bisect each other at D.

∴ BHCG is a parallelogram.
∴ BH = CG

Now, ΔBGH in BG + BH > GH
or, BG+CG > 2GD ( ∵ BH = CG and GD = DH)

or, \(\frac{2}{3}\) BE +\(\frac{2}{3}\) CF > 2. \(\frac{1}{3}\) AD

or, \(\frac{2}{3}\) BE+ \(\frac{2}{3}\) CF > \(\frac{2}{3}\) AD

or, BE+ CF > AD Proved

Question 6. AD, BE and CF are the three medians of ΔABC. Let us prove that

1. 4(AD + BE + CF) > 3(AB+ BC + CA);
2. 3(AB+ BC + CA) > 2(AD + BE + CF).

Solution: In triangle ABC, 3 medians AD, BE and CF cut each other at G. Prove that

1. 4(AD+ BE + CF) > 3(AB + BC + CA);
2. 3(AB+ BC + CA) > 2(AD + BE + CF)

 

 

Proof: ∵Medians of a triangle cut each other in a ratio-2: of 1 at the centroid.

∴ AG = \(\frac{2}{3}\) AD, BG = \(\frac{2}{3}\) BE & CG = \(\frac{2}{3}\) CF

In ΔABG, AG + BG > AB

In ΔBCG, BG + CG > BC

In ΔACG, AG + CG > CA

∴ 2 (AG + BG+CG) > AB + BC + CA

or, \(2\left(\frac{2}{3} A D+\frac{2}{3} B E+\frac{2}{3} C F\right)>A B+B C+C A\)

or, \(\frac{4}{3}\)(AD+BE+CF) > AB + BC + CA

or, 4(AD + BE + CF) > 3 (AB+ BC + CA)

2.  In ΔABD, AB + BD > AD

or, AB + \(\frac{1}{2}\) BC > AD ( ∵ D, is the midpoint of BC)

In ΔBCE, BC + CE > BE

or, BC+ \(\frac{1}{2}\) CA> BE ( ∵ E, is the midpoint of CA)

In ΔCAF, CA + AF > CF

or, CA + \(\frac{1}{2}\) AB > CF (∵ F is the midpoint of AB)

∴ AB + \(\frac{1}{2}\) BC+BC+ \(\frac{1}{2}\) CA + CA + \(\frac{1}{2}\) AB > AD+ BE + CF

or, \(\frac{3}{2}\) (AB+BC+ CA)> (AD + BE+CF)

or, 3(AB+ BC + CA) > 2(AD + BE + CF)

Question 7. Three medians AD, BE and CF of ΔABC intersect each other at point G. If the area of AABC is 36 sq cm, let us calculate

1. Area of ΔAGB;
2. Area of ΔCGE;
3. Area of quadrilateral ΔDGF.

 

 

Solution: AD is the median of ΔABC
∴ ΔABD = ΔACD
∴ ΔGBD = ΔGCD  [GD is the median of AGBC]

ΔABD- ΔGBD = ΔACD- ΔGCD
∴ ΔAGB = ΔAGC

Similarly,
ΔAGB = ΔBGC

∴ ΔAGB = ΔBGC = ΔAGC = \(\frac{1}{3}\) (ΔAGB+ΔBGC + ΔAGC)

= \(\frac{1}{3}\) ΔABC

∴ ΔAGB = \(\frac{1}{3}\) x 36 sq. cm = 12 sq. cm

2. ΔCGE = \(\frac{1}{2}\) ΔAGC

= \(\frac{1}{2}\).\(\frac{1}{3}\) ΔABC

= \(\frac{1}{6}\)ΔΑΒC

= \(\frac{1}{6}\) x 36 sq. cm = 6 cm

3. Area of quadrilateral BDGF

= \(\frac{1}{6}\) x area of ΔABC + \(\frac{1}{6}\) x area of ΔABC

= \(\frac{1}{3}\) x 36 sq. cm + \(\frac{1}{6}\) x 36 sq. cm

= (3+5) sq. cm = 12 sq. cm

Question 8. AD, BE and CF are the medians of ΔABC. If \(\frac{2}{3}\) AD = BC, then let us prove that the angle between two medians is 90°.

Solution:

 

 

Produce GD to point H, such that GD = DH. As G is the centroid of ΓABC,
∴ AG: GD = 2:1

∴ GD = \(\frac{1}{3}\) AD = DH (∵ GD = DH)

∴ GD = GD + DH

= \(\frac{1}{3}\) AD + \(\frac{1}{3}\) AD

= AD \(\frac{2}{3}\) = BC (given)

∵ AD is the median.
∴ BD = DC & GD = DH

∴ In quadrilateral BHCG, diagonals bisect each other at D.
∴ BHCG is a parallelagram.

∵ The other diagonals bisect equally  ∴BC= GH
∴ BHCG is a rectangle.

∴ ∠BGC = 90° = ∠EGF

Question 9. P and Q are the mid-points of sides BC and CD respectively of a parallelogram ABCD; the diagonals AP and AQ cut BD at the points K and L. Let us prove that, BK = KL = LD.

Solution:

 

 

Diagonal AC bisects BD at O.
Two medians of AADC, DO & AQ intersect at L.

∴ DL: LO = 2:1

∴ DL = \(\frac{2}{3}\) DO and LO = \(\frac{1}{3}\) DO

KO = \(\frac{1}{3}\) BO = \(\frac{1}{3}\) DO and BK = \(\frac{2}{3}\) BO = \(\frac{2}{3}\) DO

KL = LO+KO = \(\frac{1}{3}\) DO + \(\frac{1}{3}\) DO

= \(\frac{\mathrm{DO}+\mathrm{DO}}{3}=\frac{2}{3} \mathrm{DC}\)

∴ BK = KL = LD = \(\frac{2}{3}\) DO Proved

Question 10. Multiple choice questions

1. O is the circumcentre of ABC; if ∠BOC = 80°, the measure of ∠BAC is

1. 40°
2. 160°
3. 130°
4. 110°

 

 

 

Solution: ∠BOC = 2 ∠BAC

∴ ∠BAC = \(\frac{1}{2}\) ∠BOC

= \(\frac{1}{2}\) × 80° = 40°

Solution: 1. 40°

2. O is the orthocentre of ABC; if ∠BAC = 40°, the measure of ∠BOC is

1.  80°
2. 140°
3. 110°
4. 40°

 

 

Solution: Let in ΔABC AD, BE and CF on sides BC, CA, and AB are perpendicular; the orthocentre is O.

In □AFOE,

∠FOE = 360°-(∠OFA + ∠OEA + ∠FAE)
= 360° (90° + 90° + 40°) = 360° – 220° = 140°

∴ ∠BOC = VOA  ∠FOE = 140°

∴ 2. 140°

3. O is the orthocentre of ABC; if ∠BAC 40° then

1. 80°
2. 110°
3. 140°
4. 40°

 

 

Solution: ΔABC
∠A+∠B+∠C = 180°
or, 40°+ ∠B+∠C = 180°
or, ∠B+∠C= 180° – 40° = 140°

In ΔBOC,
∠BOC+∠OBC+∠OCB = 180°

or, ∠BOC + \(\frac{1}{2}\) ∠B+ \(\frac{1}{2}\) ∠C=180°

or, ∠BOC + \(\frac{1}{2}\)(∠B + ∠C) = 180°

or, ∠BOC+ \(\frac{1}{2}\) x 140° = 180°

or, ∠BOC+70° = 180°
or, ∠BOC = 180° – 70° = 110°

∴ 2.110°

4. G is the centroid of triangle ABC; if the area of GBC is 20 sq cm, then the area of ABC is

1.  24 sq. cm
2. 6 sq. cm
3.  36 sq. cm
4. none of them

 

 

Solution: In a triangle, ABC G is the centroid.
∴ ΔAGB = ΔGBC = ΔCGA

∴ \(\frac{1}{3}\) ΔABC = ΔGBC

or, ΔABC = 12 sq. cm

or, ΔABC = 3 x 12 sq. cm 36 sq. cm

∴ 3. 36 sq. cm

5. If the length of the circumradius of a right-angled triangle is 5 cm, then the length of its hypotenuse is

1. 2.5 cm
2. 5 cm
3. 10 cm
4. none of this

 

 

Solution: In a right-angled triangle, the circumcentre lies at the mid-point of the hypotenuse.

∵ Length of circumradius = 5 cm
∴ Lengths of hypotenuse 2 x 5 cm = 10 cm.

∴ 3. 10 cm

Question 11. Short answer type questions:

1. If the lengths of the sides of a triangle are 6 cm, 8 cm, and 10 cm, then let us write where the circumcentre of this triangle lies.

Solution: ∵ (10)² = (6)² + (8)²

∴ The triangle whose three sides are 6 cm, 8 cm, and 10 cm is a right-angled triangle. And we know that the circumcentre is at the midpoint of the hypotenuse.
∴ Circumcentre lies at the midpoint of the side of 10 cm.

2. AD is the median and G is the centroid of an equilateral triangle. If the length of side is 3√3 cm, then let us write the length of AG.

Solution: ∵ Median of an equilateral triangle = Height of the triangle

∴ AD = \(\frac{\sqrt{3}}{2}\) x side 3√3 = \(\frac{9}{2}\)

∴ AG = \(\frac{2}{3}\) AD= \(\frac{2}{3}\) x \(\frac{9}{2}\) cm = 3 cm.

3. Let us write how many points are equidistant from the sides of a triangle.

Solution: One point is equidistance from the sides of the triangle.

4. DEF is a pedal triangle of an equilateral triangle ABC. Let us write the measure of ∠FDA.

Solution: The triangle formed by the foot of perpendiculars from the vertices to the opposite sides in a triangle is called Pedal Triangle.
‘O’ is the orthocentre of ΔABC.

 

 

D, E, and F are the foot of perpendiculars of AD, BE & CF.
The triangle joining the points D, E, and F is a pedal triangle.
Δ DEF is a pedal triangle.

∵ ΔABC is an equilateral triangle.
∴ DA is the bisector of ∠FDE

∴ ∠FDA = \(\frac{1}{2}\) x 60° = 30°

5. ABC is an isosceles triangle in which ∠ABC = ∠ACB and median AD =  BC. If AB = √2 cm, let us write the length of the circumradius of this triangle.

Solution: ABC is an isosceles triangle whose ∠ABC = ∠ACB.
∴ AB AC = √2 cm

 

 

∵ AD is median
∴BD = CD

∵ AD = \(\frac{1}{2}\) BC

∴ BD = CD = AD

The height of an isosceles triangle is the median of that triangle.
∴ AD ⊥ BC

∴ In rt. angled ΔADB,
AD²+ BD² = AB²

or, AD² + AD² =   (√2)² [∵ BD = AD]

or, 2 AD²=2

or, AD² = \(\frac{2}{2}\) = 1

or, AD = √1
or, AD = 1

∴ Circumradius of the triangle = 1 cm.

 

 

Chapter 16 Circumference Of Circle

Wbbse Class 9 Maths Chapter 16 Definitions:

1. Circle: When a curved line rotates around a point keeping a fixed distance, then the figure formed by its rotation is called a circle.
2. Circumference: The curved line which forms the circle is called the circumference.
3. Centre of a circle: The point inside a circle from which the distance between any point on the circumference is equal, is called the center of the circle.

4. Radius: The distance between the center and the circumference is called the radius.
5. Diameter: The straight line passing through the center which touches the circumference on both sides is called the diameter.
6. Arc: A part of the circumference is called arc.

7. Chord: The straight line joining any two points on the circumference is called a chord. The longest chord is the diameter.
8. Cyclic Quadrilateral: The quadrilateral whose vertices lie on the circumference of a circle is called a cyclic quadrilateral.
9. Segment: A figure formed by an arc and a chord of a circle is called a segment.

Read and Learn More WBBSE Solutions For Class 9 Maths

Wbbse Class 9 Maths Chapter 16 Memorable Facts:

1. Infinite number of circles can be drawn from a point.
2. Infinite number of circles can be drawn from two points.
3. Only one circle can be drawn from three non-collinear points.

4. It is not possible to draw a circle from more than three points. If it is, then those points are called the same circle points.
5. There are an infinite number of diameters in a circle and all diameters are equal in length.
6. There are an infinite number of radii in a circle and all radii are equal in length.”

7. The part of a circle that is enclosed on one side by diameter and on the other by circumference is called a semi-circle.
8. Angle in a semi-circle is a right angle.
9. The greatest chord of a circle is its diameter.
10. All angles of the same segment of a circle are equal.

Wbbse Class 9 Maths Chapter 16  Formulae:

1. Circumference of a circle = 2πr=πd units
2. Perimeter of a semicircle = (x+2) units
3. Circumference of a semi-circle = πr units

 

Chapter 16 Circumference Of Circle Exercise 16

Question1. Let us calculate the perimeter of each of the following Images

 

 

 

 

Solution: DE = (CE-CD) = (8-5) m = 31m

In right-angled ΔADE

\(\begin{aligned}
& A E=\sqrt{A D^2+D E^2} \\
& =\sqrt{(4)^2+(3)^2} \mathrm{~m} \\
& =\sqrt{16+9} \mathrm{~m} \\
& =\sqrt{25} \mathrm{~m} \\
& =5 \mathrm{~m}
\end{aligned}\)

 

 

 

The perimeter of the semi-circle = πr²

\(\begin{aligned}
& =\frac{22}{7} \times \frac{4}{2} \mathrm{~m} \\
& =\frac{44}{7} \mathrm{~m} \\
& =6 \frac{2}{7} \mathrm{~m}
\end{aligned}\)

 

∴ Perimeter of 1st Image = (5+5+8+\(6 \frac{2}{7}\))m = \(24 \frac{2}{7}\) m

2. Perimeter of a semi-circle = πr

= \(\frac{22}{7} \times 7\) cm = 22 cm

∴ Perimeter of 2nd Image = (14+14 +14 +22) cm = 64 cm.

Question 2. Let us calculate how long the wire will be taken to make a circular ring of radius 35 meters.

Solution: Circular ring of radius = 35 m
∴ Circumference = 2πr

= \(2 \times \frac{22}{7} \times 35 \mathrm{~m}\)= 220 m

∴ 220 m is the required length of the wire.

Wbbse Class 9 Maths Chapter 16  Question 3.

The radius of a wheel of a train is 35 meters. If It makes 450 revolutions per minute, let us calculate the velocity of the train per hour.

Solution: The radius of the wheel of the train is = 0.35 m.
∴ Circumference of wheel = 2πr

= \(2 \times \frac{22}{7} \times \frac{35}{100} \mathrm{~m}\) = 2.2 m

∵ The wheel of the train moves in 1 minute = 450 revolution
∴ The wheel moves in 60 minutes = 60 x 450 revolutions.
∴ Wheel moves in 1 hr = 27000 revolution

∵ Wheel moves in 1 revolution = 2.2 m
∴ Wheel moves in 27000 revolution = 27000 × 2.2 m = 59400 m = 59.4 km

∴ Speed = 59.4 km/hr.

Question 4. The radius of the circular field of the village Amadpur is 280 meters. Chaltall wants to go around the field by walking with a speed 5.5 km/hour, let us calculate how long time will be taken by Chaltall to complete one revolution.

Solution: Radius of a circular field = 280 m.

∴ Circumference = 2πr = \(2 \times \frac{22}{7} \times \frac{35}{100} \mathrm{~m}\) = 1760 m

5.5 km 5.5 x 1000 m = 5500 m
1 hr = 60 minute = 60 x 60 sec = 3600 sec

∵ Chaitali goes 5500 m in 3600 sec

∴ She goes 1 m in \(\frac{3600}{5500}\) sec

∴ She goes 1760 m in \(\frac{3600}{5500} \times 1760\) sec

∴ She goes 1760 m in 1152 sec = 19 minutes 12 sec.

Wbbse Class 9 Maths Chapter 16 Question 5.

Tathagata bent a copper wire in the form of a rectangle whose length is 18 cm and breadth is 15 cm. I made a circle by bending this copper wire. Let us calculate the length of the radius of the circular copper wire.

Solution: Perimeter of the rectangle = 2(18+15) cm = 66 cm

If the wire is bent in form of a circle, its circumference is 66 cm.
Let the radius of the circle be r cm.

∴ Circumference of the circle = 2πr cm

\(\begin{aligned}
& =2 \times \frac{22}{7} r \mathrm{~cm} \\
& =\frac{44 r}{7} \mathrm{~cm}
\end{aligned}\)

 

 

 

As for questions,

 

 

 

\(
\frac{44 r}{7}=66
or, r=\frac{66 \times 7}{44}
or, r=\frac{21}{2}=10.5\)

∴ Radius = 10.5 cm.

Question 6. The perimeter of a semi-circular field is 108 meters. Let us calculate the diameter of the field.

Solution: Let the radius of the semi-circular field be r m.

∴ Perimeter of semi-circle = (πr+2r) m

\(\begin{aligned}
& =r(\pi+2) m \\
& =r\left(\frac{22}{7}+2\right) m \\
& =r\left(\frac{22+14}{7}\right) m \\
& =\frac{36 r}{7} m
\end{aligned}\)

As for question,

\(
\frac{36 r}{7}=108
or, r=\frac{108 \times 7}{36}\)

or, r = 21

∴ Diameter of the field = 2r m = 2 × 21 m = 42 m

Question 7. The difference between the circumference and the diameter of a wheel is 75 cm, let us calculate the length of the radius of this wheel.

Solution: Let the radius of the wheel be r cm.
∴ Circumference of the wheel 2πr cm
Diameter of the wheel = 2r cm

∴ Difference between the circumference and diameter = (2πr-2г) cm

\(\begin{aligned}
& =2 r(\pi-1) \mathrm{cm} \\
& =2 r\left(\frac{22}{7}-1\right) \mathrm{cm} \\
& =2 r\left(\frac{22-7}{7}\right) \mathrm{cm} \\
& =2 r \times \frac{15}{7} \mathrm{~cm}
\end{aligned}\)

 

As for question,

\(
2 r \times \frac{15}{7}=75
or, r=\frac{7-\times 7}{2 \times 15}
or, r=\frac{35}{2}\)

or, r = 17.5
∴ Radius = 17.5 cm.

Question 8. In a race, Puja and Jakir start to compete from the same point and same time on a circular track of a length of diameter 56 meter. When Puja finishes the race at the competition by 10 revolutions, Jakir is one revolution behind. Let us calculate how many meters is the length of the race and by how many meters Puja beats Jakir.

Solution: Radius of track = \(\frac{56}{2} \mathrm{~m}\) = 28 m

∴ Circumference = 2πr = \(2 \times \frac{22}{7} \times 28 \mathrm{~m}\) = 176 m

∴ Length of the race = 10 x 176 m = 1760 m
& Puja beats Jakir by 176 m.

Ganit Prakash Class 9 Solutions Question 9.

The perimeter of the borewell of our village is 440 cm. There is an equally wide stone parapet around this borewell. If the perimeter of the borewell with parapet is 616 cm, let us write by calculating how much is the width of the stone parapet.

Solution: Let the radius of the (borewell) be r₁ cm and the radius including the stone parapet be r₂ cm.

∴ Circumference of the borewell = 2πr₁ cm
∴ 2πr₁ = 440  ……(1)

∴ Circumference of the borewell including the stone parapet = 2πr₂ cm
∴ 2πr₂ =616   ……(2)

 

 

Subtracting (1) from (2),
2πr₂-2πr₁ = 616-440
or, 2π(r₂-r₁) =176

\(or, 2 \times \frac{22}{7}\left(r_2-r_1\right)=176
or, r_2-r_1=\frac{176 \times 7}{2 \times 22}\)

 

or, r₂ -r₁ = 28
∴ Width of stone parapet is 28 cm.

Question 10. Niyamat chacha of the village attaches the motor’s wheel with a machine’s wheel with a belt. The length of diameter of the motor wheel is 14 cm and the machine wheel is 94.5 cm. If the motor’s wheel revolves 27 times in a second then let us calculate how many times the machine’s wheel will revolve in an hour.

Solution: Radius of the wheel of motor (r) = \(\frac{14}{2}\) = 7cm

∴ Circumference of motor’s wheel = 2πr

= \(2 \times \frac{22}{7} \times 7 \mathrm{~cm}\) = 44cm

∴ In 1 revolution motor wheel moves 44 cm.
∵ In 27 revolutions it moves = 44 x 27 cm
∴ In 1 hour it moves = 3600 x 27 x 44 cm

Radius of the motor wheel = \(\frac{94.5}{2} \mathrm{~cm}\) = 47.25 cm

Circumference of the motor wheel = 2πr

= \(2 \times \frac{22}{7} \times \frac{4725}{100} \mathrm{~cm}\) = 297 cm

∴ The motor wheel can traverse a 297 cm distance in 1 revolution.

∴ The motor wheel can traverse 1 cm distance in \(\frac{1}{297}\) revolution

∴ The motor wheel can traverse a 3600×27 x 44 cm distance in \(\frac{1 \times 3600 \times 27 \times 44}{297}\) revolution.

∴ The motor wheel can traverse 3600 x 27 x 44 cm distance in 14400 revolution.
∴ In an hour the motor wheel will revolve 14400 times.

Question 11. The lengths of hour’s hand and minute’s hand are 8.4 cm and 14 cm respectively of our club clock. Let us calculate how much distance will be covered by each hand in a day.

Hints: Hour’s hand goes in 12 hours = \(2 \times \frac{22}{7} \times 8.4 \mathrm{~cm}\)

Minute’s hand goes in one hours = \(2 \times \frac{22}{7} \times 14 \mathrm{~cm}\)

Solution: Radius of hour hand (r) = 8.4 cm.

∴Hour’s hand will traverse distance in 12 hours = \(2 \times \frac{22}{7} \times 8.4 \mathrm{~cm}[/latex = 52.8 cm

∴ Hour’s hand will traverse distance in 1 hours = [latex]\frac{52.8}{12} \mathrm{~cm}\)

∴ Hour hand will move in a day (= 24 hours) twice = \(\frac{52.8}{12} \times 24 \mathrm{~cm}\)

 

 

∵ Circumference = \(2 \times \frac{22}{7} \times 14 \mathrm{~cm}\) = 88 cm.

∴ The minute hand will moves in a day (= 24 here) 24 x 88 cm = 2112 cm.
∴ In a day the hour’s hand and minute’s hand will move 105.6 cm and 2112 cm respectively.

Question 12. The ratio of diameters of two circles which are drawn by me and my friend Mihir is □:□ It was found by calculating that the ratio of perimeters of two circles is □:□

Solution: If the ratio of diameter = x:y of two circles then the ratio of their perimeter (circumference) is also x:y.

Ganit Prakash Class 9 Solutions Question 13.

The time that Rahim takes to cover up by running a circular field is 40 seconds less when he goes from one end to another end diametrically. The velocity of Rahim is 90 meters per minute. Let us calculate the length of the diameter of the field.

Solution: Let the radius of the circular field be r m.

∴ Circumference of the circular field = 2πr m.
Diameter of the circular field = 2r m.

∵ Rahim can travel a 1m distance in \(\frac{60}{90}\)

∴ Rahim can travel 2πr m distance in \(\frac{2}{3} \times 2 \pi r \text { sec }\)

∴ Rahim can travel 2 r m distance in \(\frac{2}{3} \times 2 r\)

By the problem,

\(
\frac{2}{3} \times 2 \pi r-\frac{2}{3} \times 2 r=40
or, \frac{2}{3} \times 2 r(\pi-1)=40
or, \frac{2}{3} \times 2 r\left(\frac{22}{7}-1\right)=40\)

 

\(or, \frac{2}{3} \times 2 r\left(\frac{22-7}{7}\right)=40
or, \frac{2}{3} \times 2 r \times \frac{15}{7}=40
or, r=\frac{40 \times 7 \times 3}{2 \times 2 \times 15}\)

or, r = 14
∴ Diameter of the field = 2r m = 2 x 14 m = 28 m.

Question 14. The ratio of perimeters of two circles is 2 : 3 and the difference of their length of radii is 2 cm. Let us calculate the lengths of diameters of the two circles.

Solution: The radius of the smaller circle = r cm
∴ Radius of the larger circle = (r + 2) cm
∴ Radius of the smaller circle circle = 2πr cm and circumference of the larger circle = 2π(r+2) cm

B.T.P.,
2πг: 2π(г+2)=2:3

\(or,$\frac{2 \pi r}{2 \pi(r+2)}=\frac{2}{3}
or, \frac{r}{r+2}=\frac{2}{3}\)

or, 3r = 2r+4

or, 3r – 2r=4
or, r = 4

∴ Diameter of the first circle = 2r cm
= 2 x 4 cm = 8 cm.

∴ Diameter of the second circle = 2(r + 2) cm
= 2(4+2) cm = 12 cm.

Ganit Prakash Class 9 Solutions Question 15.

The four maximum-sized circular plates are cut out of a brass plate of square size. Having an area of 196 sq. cm; let us calculate the circumference of each circular plate.

Solution: The area of the square plate is 196 sq. cm

∴ Length of each side = √196 cm = 14.cm

Diameter of each circle = \(=\frac{14}{2} \mathrm{~cm}\) = 7 cm

∴ The radius of the maximum-sized circular plate cut out of a square plate of side 7 cm will be 7 cm.

 

 

∴ Radius of each circular plate (r) = latex]\frac{7}{\dot{2}} \mathrm{~cm}[/latex]

∴ Circumference of each circular plate p = 2πr cm

= \(2 \times \frac{22}{7} \times \frac{7}{2} \mathrm{~cm}\) = 22 cm

Question 16. The time that Nashifer takes to cover up a circular field from one end to other end is 45 seconds less when he goes diametrically. Let us calculate the length of the diameter of this field.

Solution:

 

Let the radius of the circular field r m
∴ Semi-circumference of the circular field = r m
Diameter of the circular field = 2r m

∴ Nashifer covers up 80 m distance in 60 sec

∴ Nashifer covers up 1 m distance in \(\frac{60}{80} \mathrm{sec}\)

∴ Nashifer covers up r m distance in \(\frac{3}{4} \times \pi r \sec\)

∴ Nashifer covers up 2r m distance in \(\frac{3}{4} \times 2 r\)

B.T.P.,

\(
\frac{3}{4} \times \pi r-\frac{3}{4} \times 2 r=45
or, \frac{3}{4} r(\pi-2)=45\) \(\text { or, } \frac{3}{4} r\left(\frac{22}{7}-2\right)=45\) \(\text { or, } \frac{3}{4} r\left(\frac{22-14}{7}\right)=45\) \(\text { or, , } \frac{3}{4} r \times \frac{8}{7}=45\) \(\text { or, } r=\frac{45 \times 7 \times 4}{8 \times 3}\) \(\text { or, } r=\frac{105}{2}\)

 

Diameter of the field = 2r m

\(\begin{aligned}
& =2 \times \frac{105}{2} \mathrm{~m} \\
& =105 \mathrm{~m}
\end{aligned}\)

Ganit Prakash Class 9 Solutions Question 17.

Mohim takes 46 seconds and 44 seconds respectively to go around along the outer and the inner edges of a circular path with 7 meters 5 dcm width by a cycle. Let us calculate the diameter of the circle along the inner edge of the path.

Solution: Let the inner radius of the circular path = r m
Width of the path = 7 m 5 dcm = 7.5 m

∴ The other radius of the circular path = (r+7.5) m
∴ Inner circumference = 2πr m
And other circumference = 2(r+7.5) m

To cover up 2π(r+7.5) m distance it takes 46 seconds

∴ To cover up 1 m distance it takes \(\frac{46}{2 \pi(r+7.5)} \text { seconds }\)

∴ To cover up 2πr m distance it takes \(\frac{46 \times 2 \pi r}{2 \pi(r+7.5)} \text { seconds }\)

B.T.P..

 

 

 

\(\begin{aligned}
& \frac{46 \times 2 \pi r}{2 \pi(r+7.5)}=44 \\
& \text { or, } \frac{46 r}{r+7.5}=44
\end{aligned}\)

or, 46r = 44r + 330
or, 46r- 44r330
or, 2r=330

∴ Diameter of the circle along the inner edge of the path = is 330 m. Ans.

Class 9 Ganit Prakash Solutions

Question 18. The ratio of time taken by a cyclist to go around the outer and inner circumference of a circular path is 20: 19; if the path is 15 meters wide, let us calculate the length of the diameter of the inner circle.

Solution: Width of the path = 5 m.
Let the inner radius of a circular path be r m and
the speed of the cycle is v m/second.

∴ The outer radius of the circular path = (r + 5) m
∴ The inner and outer circumferences of the path is 2r m and 2π(r+5) m respectively.

 

 

∴ Cycle covers up v m distance in 1 sec

∴ Cycle covers up 1 m distance in \(\frac{1}{v} \sec\)

∴ Cycle covers up 2πr m distance in \(\frac{2 \pi r}{v} \sec\)

∴ Cycle covers up, 2π(r+5) m distance in \(\frac{2 \pi(r+5)}{v} \sec\)

B. T. P.,

\(\frac{2 \pi\left(r+5\right)}{v}: \frac{2 \pi r}{v}=20: 19\) \(\text { or, } \frac{\frac{2 \pi(r+5)}{v}}{\frac{2 \pi r}{v}}=\frac{20}{19}\) \(\text { or, } \frac{2 \pi(r+5)}{v} \times \frac{v}{2 \pi r}=\frac{20}{19}\) \(\text { or, } \frac{r+5}{r}=\frac{20}{19}\)

or, 20r = 19r+ 95
or, 20r – 19r = 95
or, r = 95

∴Length of the inner diameter of the path = 2r m
= 2 x 95 m 190 m.

Class 9 Maths Chapter 16 Circumference Of Circle Multiple Choice Questions

 

1. The ratio of the velocity of the hour’s hand and minute’s hand at a clock is

1. 1: 12
2. 12:1
3. 1:24
4. 24: 1

Solution: Let the radius of the clock be r unit.
∴ Circumference of the clock = 2πr unit.

∵ The hour’s hand in 12 hours covers up 2πr unit distance.

∴ The hour’s hand in 1 hours covers up \(\frac{2 \pi r}{12}\)

∵ The minute’s hand in 1 hour covers up 2πr unit distance.

∴ Rotio of the velocity of hour’s hand and minute’s hand = \(\frac{2 \pi r}{12}: 2 \pi r\)

\(\begin{aligned}
& =\frac{1}{12}: 1 \\
& =\frac{1}{12} \times 12: 1 \times 12 \\
& =1: 12
\end{aligned}\)

∴ 1. 1: 12

Class 9 Ganit Prakash Solutions

2. Soma takes \(\frac{\pi x}{100}\) minutes to go one complete round of a circular path. Soma will take how much time for going around the park diametrically?

1. \(\frac{x}{200}\) minute

2. \(\frac{x}{100}\) minute

3. \(\frac{\pi}{100}\) minute

4. \(\frac{\pi}{200}\) minute

Solution: Let the radius of the circular park be r m.
∴ Circumference of the circular park = 2πr m and
Diameter of the circular park = 2r m

∴ Soma will take to cover up 2πr m distance \(\frac{\pi x}{100}\) minute

∴ Soma will take to cover up 1 m distance \(\frac{\pi \mathrm{x}}{100.2 \pi \mathrm{r}}\) minute

∴ Soma will take to cover up 2r m distance \(\frac{\pi x .2 r}{100.2 \pi r}\) minute

∴ Soma will take to cover up 2r m distance \(\frac{x}{100}\) minut

∴ Soma will take time for going round the park diametrically \(\frac{x}{100}\) minute

∴ 2. \(\frac{x}{100}\) minute

3.  A circle is inscribed by a square. The length of a side of the square is 10 cm. The length of diameter of the circle is

1. 10 cm
2. 5 cm
3. 20 cm
4. 10√2 cm

 

 

Solution: Let ABCD is a square in which
AB = BC= CD = DA = 10 cm

∵ A circle is inscribed by the square.
∴ Length of the diameter of the circle Length of each side of the square

∴ 1. 10 cm

Class 9 Ganit Prakash Solutions

4. The minute’s hand of a clock is 7 cm. How much length does the minute’s hand move in 15 minutes?

1. 5√2 cm
2. 10√2 cm
3. 5 cm
4. 10 cm

 

 

Solution: Length of each side of the square 5 cm.

∴ Length of diagonal of the square = √2x side
= √2×5 cm =5√2 cm

∵ A square is inscribed in a circle.
∴ Diameter of the circle = 5√2 cm

Solution: 1. 5√2 cm

Wbbse Class 9 Ex 16

5. A circular ring is 5 cm wide. The difference between the outer and inner radii is

1. 5 cm
2. 2.5 cm
3. 10 cm
4. none of these.

 

 

Solution: Width of the circular ring = 5 cm.
Let the inner radius of the circular ring be r cm.

∴ The outer radius of the ring = (r+ 5) cm.
∴ Difference between outer and inner radius of the ring = {(r+5)-r} cm = (r+5-r) cm = 5 cm

Answer: 1. 5 cm

Class 9 Maths Chapter 16 Circumference Of Circle Short Answer Type Questions

1. Perimeter of a semi-circle is 36 cm. What is the length of the diameter?

Solution: Let the radius of the semi-circle be r cm.
∴ Perimeter of the semi-circle = (r+2r) cm
Diameter of the semi-circle = 2r cm.

 

 

B. T. P., πг+2r=36
or, r(x+2)=36

\(\text { or, } r\left(\frac{22}{7}+2\right)=36\) \(\text { or, } r\left(\frac{22+14}{7}\right)=36\) \(or, r \times \frac{36}{7}=36
or, r=\frac{36 \times 7}{36}\)

or, r = 7
∴ Length of the diameter of the semi-circle = 2r cm = 2 x 7 cm = 14 cm.

Class 9 Ganit Prakash Solutions 

2. The length of the minute’s hand is 7 cm. How much length will a minute’s hand go to rotate 90°?

Solution: Length of the minute’s hand of the watch = 7 cm

∴ Circumference of the clock = 2πr

\(2 \times \frac{22}{7} \times 7 \mathrm{~cm}\) = 44 cm

 

 

∵ To rotate 360° degrees the minute’s hand will cover 44 cm distance.

∴ To rotate 1° degrees the minute’s hand will cover \(\frac{44}{360} \mathrm{~cm}\) distance

∴ To rotate 90° degrees the minute’s hand will cover \(\frac{44}{360} \times 90\) distance.

∴ To rotate 90° degrees the minute’s hand will cover \(\frac{44}{360} \times 90\) cm distance.

 Wbbse Class 9 Ex 16 

3. What is the ratio of radii of the inscribed and circumscribed circles of a square?

Solution: Let each side of the square = a unit.

∴ Diameter of the inner circle = a unit.

∴ Radius of the inner circle = \(\frac{a}{2}\) unit.

 

 

Diameter of the circumcircle = Length of the diagonal of the square = √2a unit.

∴ Radices of the circumcircle = \(\frac{\sqrt{2} a}{2} \text { unit. }\)

∴ Ratio of radii of inscribed circle and circumscribed circle = \(=\frac{a}{2}: \frac{\sqrt{2} a}{2}=1: \sqrt{2}\)

4. The minute’s hand of a clock is 7 cm. How much longer does the minute’s hand move in 15 minutes?

Solution: Radius of the circle = 7 cm.

∴ Circumference of the clock = 2πr

= \(2 \times \frac{22}{7} \times 7 \mathrm{~cm}=44 \mathrm{~cm}\)

∴ The minute hand moves in 60 minutes = 44 cm.

∴ It will move in 15 minute =\(\frac{44}{60}\)

∴ The minute’s hand in 15 minutes will cover = \(\frac{44}{60} \times 15 \mathrm{~cm}\) = 11 cm.

Wbbse Class 9 Ex 16

5. What is the ratio of the side of a square and the perimeter of a circle when the length of the diameter of the circle is equal to the length of the side of the square?

Solution: Let the radius of the circle = r cm.
∴ One side of the square = 2r unit.

 

 

∴ Circumference 2πr cm.
∴ Length of one side of the square = 2r cm
= 4 × side
= 4 x 2r cm = 8r cm.

∴ Ratio of the side of the square & the circumference
= 2πг: 8r
= π: 4

\(\begin{aligned}
& =\frac{22}{7}: 4 \\
& =\frac{22}{7} \times 7: 4 \times 7=22: 28
\end{aligned}\)

∴ 11:14

Chapter 14 Construction Of A Triangle Exercise 14

Question 1. Pritam drew a quadrilateral ABCD of which AB = 5 cm, BC = 6 cm, CD = 4 cm, DA = 3 cm, and ∠ABC = 60°. I drew a triangle with an equal area of that quadrilateral.

Solution:

 

Construction: First, a straight line BX is drawn. From BX, BC is cut off equal to 6cm. On point B equal to a 60° angle ∠CBY is drawn. From BY equal to AB 5 is cut off Taking A as the center and taking a radius equal to 3 cm a radius is drawn. Again, taking C as a center and taking a radius equal to 4 cm, an arc is drawn.

Read and Learn More WBBSE Solutions For Class 9 Maths

Both arcs intersect each other at the D point. A, D, and C, D are joined. Consequently, ABCD, a quadrilateral whose sides AB = 5 cm, BC = 6 cm, CD = 4 cm, AD = 3 cm, and ∠ABC 60°, is formed.

Diagonal AC is drawn D From point parallel to AC a st. line is drawn which cuts BX at point E. A, and E is joined. ABE is the required triangle whose area is equal to quadrilateral ABCD.

Question 2. Sahana drew a quadrilateral ABCD of which AB = 4 cm, BC = 5 cm, CD = 4.8 cm, DA = 4.2 cm and diagonal AC = 6 cm. I draw a triangle with an equal area of the quadrilateral.

Solution:

 

 

Equal in area to quadrilateral ABCD, triangle ABE is drawn.

Question 3. Sahana drew a rectangle ABCD, of which AB = 4 cm and BC = 6 cm. I draw a triangle with an equal area of that rectangle.

Solution:

 

 

Equal in the area to rectangle ABCD, triangle ADE is drawn.

Question 4. I draw a quadrilateral ABCD of which BC = 6 cm, AB = 4 cm, CD = 3 cm, ∠ABC 60°, ∠BCD = 55°. I draw a triangle with an equal area of that quadrilateral of which one side is alongside AB and another side is alongside BC.

Solution:

 

 

Equal in area to quadrilateral ABCD, triangle ABE is drawn.

Question 5. I draw a square with side of 5 cm. I draw a parallelogram of which one angle is 60°, equal area to the square.

Solution:

 

 

Equal in area to ABCD a square of side 5 cm ΔABE is drawn and equal in area to ΔABE a parallelogram CEGF is drawn whose angle ∠FCE = 60°.

Question 6. I draw a square with a side 6 cm and I draw a triangle with an equal area to that square.

Solution:

 

 

A square of side 6 cm, ABCD is drawn and equal in area to this square, a triangle ABE is drawn.

Question 7. I draw a quadrilateral ABCD, of which AD and BC are perpendicular on side AB and AB = 5 cm, AD = 7 cm, and BC = 4 cm. I draw a triangle with an equal area of that quadrilateral of which one angle is 30°.

Hints: I draw a ΔABQ with equal area of quadrilateral ABCD. Taking BQ as base of ΔABC I draw another triangle with same base and between two parallels of which one angle is 30°.

Solution:

 

 

Construction: From AY straight line a 5 cm long segment, AB is cut off. On A and B. points respectively perpendiculars AX and BM are drawn. From AX a 7 cm long segment AD and from BM a 4 cm long segment BC are cut. C and D are joined. Consequently, quadrilateral ABCD is formed.

Equal in area to □ABCD ΔADP is drawn. From point D parallel to AP a straight line DN is drawn. On point A, ∠PAF = 30° is drawn. AF cuts DN at point E.

Joining E, P, ΔAPE is drawn whose area is equal to □ABCD and one angle is 30°.

Proof: ΔAPD = ΔBCD

Again, ΔAPD and ΔAPE lie on same base AP and in between parallel lines AP and DE.

∴ ΔAPD = ΔAPE
∴ ΔAPE = □ABCD (Both are equal to ΔAPD)

Question 8. I draw any pentagon ABCDE and draw a triangle equal in area to it, of which one vertex is C.

Solution:

 

 

ABCDE, a pentagon is drawn. AC and CE diagonals are drawn. B From the point parallel to CA a straight line is drawn which cuts extended EA at point P.

Again, from point D parallel to CE a straight line is drawn which cuts extended AE at point Q C, P and C, Q are joined. ΔCPQ is the required triangle equal in area to pentagon ABCDE.

 

 

Chapter 13 Construction Exercise 13

Question 1. We draw a line segment PQ of length 5 cm. We take an external point A on the line segment. Let us draw a parallel line through point A to line segment PQ. [Let us draw three alternative processes].

Solution:
First method:

 

 

1. First draw a 5 cm long straight line PQ with the help of scale and outside PQ take any point.
2. On PQ straight line take any point R. A and R joined by scale, consequently is formed ∠ARQ. Now on point A on the opposite side of ∠ARQ ∠RAS joining is formed S and A by the scale and extending on both sides, got straight line XY

∴ ∠SAR = ∠ARQ, Alternate angles. XY II PQ
∴ XY is the required line through A and parallel to PQ.

Read and Learn More WBBSE Solutions For Class 9 Maths

Second Method:

 

 

1. First draw a 5 cm long straight line PQ with help of scale and outside PQ take any point.
2. On PQ straight line takes any point D.
3. D and A are joined by scale.

DA is extended to point E, consequently, ∠ADQ is formed.

4. On the same side of AD, equal to ∠ADQ on straight line EA at point A, ∠EAB is formed.
5. BA straight line is extended to point R.

∴ ∠EAB = ∠ADQ (corresponding angles). ∵RB II PQ
RB is the required straight line through A and parallel to PQ.

Third Method:

 

 

1. First draw a 5 cm long straight line PQ with the help of scale and outside PQ take any point
2. On PQ straight line take any point B. B and A are joined.
3. From BQ a segment BO is cut off.
4. Taking O as the centre, and taking a radius equal to BA, an arc is drawn.

5. Now taking A as a centre and taking a radius equal to BO another arc is drawn which cuts the former at point R. Joining A and R by the scale and extending on both sides we get MN straight line.

∴MN is the required straight line through A and parallel to PQ.

Question 2. We draw a triangle with lengths of sides 5 cm, 8 cm and 11 cm and draw a parallelogram equal in area to that triangle and having an angle of 60°. [Let us write instruction process and proof].

Solution:
1. By scale, an 11 cm straight line BC is drawn. Taking B and C as centres, and taking radii equal to 8 cm and 5 cm respectively, two arcs are drawn which cut each other at point A. A B and AC are joined. ABC is a triangle whose sides AB = 8 cm, AC 5 cm and BC= 11 cm.
2. In the ΔABC side BC is bisected at point D.
3. In ΔABC from A to BC a parallel straight line PQ is drawn.
4. In ΔABC on side BC at point D an angle of 60°, ∠GDC is drawn which cuts PQ at E.

5. From EQ, DC equal to EF is cut and a joining C, F, and CDEF parallelogram is formed.

 

 

 

 

6. Proof: A, and D are joined.

In □EDCF, DC II EF (By construction) and DC EF (By construction)
∴ EDCF is a parattelogram.

ΔADC and parallelogram □EDCF are on the same base DC and between parallel lines DC and EF.

∴ ΔADC = \(\frac{1}{2}\) parallelogram □EDCF …..(1)

Again, in □ABC, AD is the median

∴ ΔADC = \(\frac{1}{2}\) ΔABC

From (1) and (2), ΔABC = parallelogram □EDCF
∴ Equal to ΔAABC in the area a parallelogram EDCF is formed whose one angle is 60°.

Question 3. We draw a triangle in which AB = 6cm, BC =9 cm, ∠ABC= 55°, let us draw a parallelogram equal in area to that triangle having an angle of 60° and length of one side is \(\frac{1}{2}\) of AC.

Solution:
1. A straight line AP is drawn in which segment AB equal to 6 cm is cut off. On point angle, ∠ABQ= 55° is drawn. From segment BQ, BC equal to 9 cm is cut off. A and C are joined; consequently ∠ABC is formed whose side AB = 6 cm, BC = 9 cm and <ABC = 55°

 

 

2. In ΔABC side AC is bisected at point D.
3. In ΔABC from point B parallel to AC an st. line XY is drawn.
4. In ΔABC on side AC at point D equal to 60° angle GDC is drawn which cuts XY at point E.
5. DC equal to EF is cut off from EY and joining C and F a parallelogram CDEF is formed.

Proof: B and D are joined.

In □CDEF, DC II EF (By construction) and DC = EF (By construction)
∴ CDEF is a parallelogram.

ΔBDC and parallelogram CDEF lie on same base DC and in between parallel lines DC and XY.

∴ ΔBDC = \(\frac{1}{2}\) parallelogram □CDEF

Again, in ΔABC, BD is the median

∴ ΔBDC = \(\frac{1}{2}\) ΔABC

∴ \(\frac{1}{2}\) ΔΑΒC = \(\frac{1}{2}\) parallelogram □CDEF

∴ ΔABC = parallelogram □ CDEF

Question 4. In ΔPQR, ∠PQR = 30°, ∠PRQ = 75° and QR = 8 cm. Let us draw a rectangle equal in area to that triangle.

Solution:
1. PQR is a triangle whose ∠PQR = 30°, ∠PRQ = 75° and QR = 8 cm.
2. In ΔPQR from point P parallel to RQ a straight line MN is drawn.
3. RQ is bisected at S which cuts MN at T.
4. From TN equal to SQ segment TO is cut and O, and Q are joined. TSQO is the required rectangle.

 

 

 

 

 

Proof: P and S are joined.
In □TSQO, SQ II TO (By construction)
and SQ = TO (By construction)
and ∠TSQ = 90°

∴TSQO is a rectangle.
ΔPSQ and rectangle TSQO lie on the same base SQ and in between parallel lines SQ and PO.

∴ ΔPSQ= \(\frac{1}{2}\) rectangle TSQO

Again, in ΔPRQ, PS is the median

∴ ΔPSQ =\(\frac{1}{2}\) ΔPRQ

∴ \(\frac{1}{2}\) ΔPRQ= \(\frac{1}{2}\) rectangle TSQO

∴ PRQ rectangle TSQO Proved

Question 5. Draw an equilateral triangle with a length of each side of 6.5 cm and let us draw a parallelogram equal in area to that triangle and having an angle of 45°.

Solution:
Construction process:
1. An equilateral triangle AABC having a side 6 cm is drawn.
2. In ΔABC side BC is bisected at point D and from point A parallel to BC a straight line PQ is drawn.
3. In ΔABC at point D on side BC angle ∠EDC equal to 45° is drawn which cuts side PQ at point E.
4. Equal to DC EF is cut from the EQ segment. Joining C and F, the parallelogram CDEF is formed.

 

 

Proof: In □CDEF, DC II EF (By construction) and CD = EF (By construction)
∴ CDEF is a parallelogram.

ΔADC and parallelogram CDEF lie on the same base CD and in between parallel lines CD and AF.

∴ ΔADC = \(\frac{1}{2}\) parallelogram □CDEF

Again, in ΔABC, AD is the median

∴ΔADC = \(\frac{1}{2}\) ΔABC

∴ \(\frac{1}{2}\) ΔABC= \(\frac{1}{2}\) parallelogram □CDEF

∴ ΔABC = parallelogram □CDEF Proved

Question 6. The length of each equal side of an isosceles triangle is 8 cm and the length of the base is 5 cm. Let us draw a parallelogram equal in area to that circle and having one angle of a parallelogram is equal to one of the equal angles of an isosceles triangle and one side is \(\frac{1}{2}\) of equal side.

Solution: ABC, an isosceles triangle is formed whose base BC = 5 cm and equal sides AB = AC = 8 cm.

 

 

Equal to this triangle in area, a parallelogram ADEF is formed whose ∠D is equal to ∠C of the isosceles triangle.

Question 7. Construct an isosceles triangle whose equal sides are each equal to 8 cm and the vertical angle is 30°. Construct a parallelogram equal in area to this triangle.

Solution: ΔABC, an isosceles triangle is formed whose sides AB = AC = 8 cm and ΔBAC = 30°.

 

Equal to this triangle in the area is the parallelogram BEGH is formed.

 

Chapter 12 Theorems On Area Exercise 12

Question 1. P and Q are the midpoints of sides AB and DC of parallelogram ABCD, let’s prove that the area of quadrilateral field = \(\frac{1}{2}\) x area of parallelogram field.

Solution:

 

 

Join: P, Q.

Proof: ∵ P is the midpoint of AB

∴ AP = \(\frac{1}{2}\) AB

= \(\frac{1}{2}\) DC [∵ opposite sides of parallelogram]

= QC (∵ Q is the midpoint of CD)

Read and Learn More WBBSE Solutions For Class 9 Maths

In □PCQ, AP = QC & AP II QC (∵ AB II DC)
∴ APCQ is a parallelogram.

In □PQD, AP II DQ & AP = DC
∴  APQD is a parallelogram.

In □PBCQ, PB = QC & PB II QC
∴ PBCQ is a parallelogram.
∴ Area of quadrilateral APCQ = ΔAPQ+ΔPCQ

= \(\frac{1}{2}\) parallelogram □APQD + \(\frac{1}{2}\) parallelogram □PBCQ

= \(\frac{1}{2}\) (parallelogram □APQD+ parallelogram □PBCQ)

= \(\frac{1}{2}\) Area of parallelogram ABCD  Proved

Question 2. The distance between two sides AB and DC of a rhombus ABCD is PQ and the distance between sides AD and BC is RS; let’s prove that PQ = RS.

Solution:

 

 

To prove PQ = RS.

Proof: ∵  Area of rhombus = base x height
∴ Area of rhombus ABCD = AB.PQ

Again, the area of rhombus ABCD = BC.RS
∴ AB.PQ=BC.RS
∴ As AB = BC (Side of the rhombus)
∴ PQ = RS Proved

Question 3. P and Q are the mid-points of sides AB and DC of parallelogram ABCD respectively. Let’s prove that PBQD is a parallelogram and ΔPBC= \(\frac{1}{2}\) parallelogram PBQD.

Solution:

 

 

 

To prove
1. PBQD is a parallelogram

2. ΔPBC =  \(\frac{1}{2}\) Parallelogam PBQD

Join: P & C.

Proof: ∵ ABCD is a parallelogram.
∴ AB = DC

or, \(\frac{1}{2}\) AB= \(\frac{1}{2}\) DC

or, PB = DQ (∵ P & Q are the mid-points of the sides AB & DC respectively)

In parallelogram □PBQD, PB = DQ & PB II DQ (∵ AB II DC)
∴ PBQD is a parallelogram  …… (1)

∴ ΔPBC & parallelogram PBQD are on the same base PB & between the same parallel straight lines PB & DC.

∴ ΔPBC = \(\frac{1}{2}\) parallelogram □PBQD ….(2) Proved

Question 4. In an isosceles triangle, ABC, AB = AC, and P is any point on produced side BC. PQ and PR are perpendicular on sides AB and AC from the point P respectively. BS is perpendicular on side AC from point B; let’s prove that PQ-PR = BS.

Solution:

 

 

 

To prove, PQ-PR = BS
Join: P, Q.

Proof: Area ΔABP = area of ΔABC+ area of ΔAPC

or, \(\frac{1}{2}\) . AB.CQ = \(\frac{1}{2}\) AC.BS+ \(\frac{1}{2}\) .AC.PR

,or, AB.PQ = AC(BS +PR)
or, AC. PQ AC (BS+ PR) (∵ AB = AC)

or, PQ=BS + PR
or, PQ-PR = BS Proved

Question 5. O is any point outside the equilateral triangle ABC and within the angular region on ABC; OP, OQ and OR are the perpendicular distances of AB, BC, and CA respectively from the point O. Let us prove that the altitude of the triangle = OP + OQ – OR.

Solution:

 

 

To prove the Height of the triangle = OP + OQ – OR.

Join: O, A; O, B and O, C & AS is the perpendicular from A on BC.

Proof : ΔABC = ΔAOB + ΔBOC – ΔAOC

or, \(\frac{1}{2}\).BC.AS = \(\frac{1}{2}\).AB.OP+\(\frac{1}{2}\).BC.OQ – \(\frac{1}{2}\) AC.OR

or, BC.AS = BC. OP+ BC. OQ – BC. OR [∵ AB = BC = AC]
or, AS OP + OQ – OR
or, Height of the triangle = OP+OQ-OR Proved

Question 6. A straight line parallel to AB of parallelogram ABCD intersects sides AB, AC, and BC or their produced parts at the points E, F, and G respectively. Let’s prove that ΔAEG = ΔAFD.

Solution:

 

 

To prove  ΔAEG = ΔAFD

Join: A, G & D, F.

Proof: ΔACG & parallelogram CDEG are on the same base CG & in between two parallelogram lines CG & AD.

∴ ΔACG = \(\frac{1}{2}\) parallelogram □CDEG

Again, ΔCDF & parallelogram □CDEG are on the same base & in between two parallel lines CD & EG.

∴ ΔCDF = \(\frac{1}{2}\) parallelogram □CDEG

∴ ΔACG = ΔCDF …..(1)

∵ AC is the diagonal of parallelogram ABCD.
∴ ΔABC = ΔADC ……(2)

Subtracting (1) from (2), ΔABC – ΔACG = ΔADC – ΔCDF
or, ΔABG = ΔAFD
or, ΔAEG = ΔAFD ( ∵ AG is the diagonal of the parallelogam ABGE)
∴ ΔABG = ΔAEG Proved

Question 7. E is any point on side DC of parallelogram ABCD, produced AE meets produced BC at the point F. D, F are joined. Let’s prove that (1) ΔADF= ΔABE; (2)ΔDEF = ΔBEC.

Solution:

 

 

Join: D, F. To prove (1) ΔADF = ΔABE (2) ΔDEF = ΔBEC.

Proof: ΔADF & parallelogram ABCD are on the same base AD & in between two parallelograms AD & BF

∴ ΔADF = \(\frac{1}{2}\) parallelogram □ABCD

Again, ΔABE & parallelogram □ABCD are on the same base AB & between two parallel straight lines AB & DC.

∴ ΔABE = \(\frac{1}{2}\) parallelogram □ABCD

∴ ΔADF = ΔABE
∴ ΔADE+ ΔBEC = \(\frac{1}{2}\) □ABCD

or, ΔADE+ ΔBEC = ΔADF
or, ΔADE + ΔBEC = ΔADE + ΔDEF
or, ΔBEC = ΔDEF
or, ΔDEF = ΔBEC

Question 8. Two triangles ABC and ABD with equal area stand on the opposite side of AB. Let’s prove that AB bisects CD.

Solution:

 

 

To prove AB bisects CD.
CP & DQ are two perpendiculars drawn on AB from points C & D respectively. CD cuts AB at O.

Proof: ∵ΔABC= ΔABD (given)

∴ \(\frac{1}{2}\).AB.OP = \(\frac{1}{2}\).AB.DQ

or, CP = DQ
In ΔCOP & ΔDOQ,
CP = DQ (proved)

∠CPO = ∠DQO (= 90°)
∠COP = vertically opposite ∠DOQ

∴ ΔCPO = ΔDOQ (A-A-S condition)
∴ CO = OD
∴ AB bisects CD. Proved

Question 9. D is midpoint of side BC of triangle ABC. Parallelogram CDEF stands between side BC and parallels to BC through point A. Let’s prove that ΔABC = parallelogram □CDEF.
Solve: To prove AABC = parallelogram CDEF.

Solution:

 

 

 

Join: A, D.

Proof: ΔADC & parallelogram CDEF are on the same base CD & in between two parallel straight lines CD & AF.

∴ ΔADC = \(\frac{1}{2}\) parallelogram □CDEF

∵ AD is the median of ΔABC

∴ ΔADC = \(\frac{1}{2}\) ΔABC

∴ \(\frac{1}{2}\) ABC = parallelogram □CDEF

or, ΔABC = parallelogram □CDEF Proved

Question 10. P is any a point on diagonal BD of parallelogram ABCD. Let’s prove that ΔAPD = ΔCPD.

Solution:

 

 

To prove ΔAPD = ΔCPD
AE & CF are two perpendiculars on BD from points A & C respectively.

Proof: In ΔADE ΔBCF
∠ADE = alternate ∠CBF
∠AED = ∠CFB
& AD = BC

∴ ΔADE ≅ ΔBCF
∴ AE = CF

(∵ AD II BC & BD is the transversal)
[each angle is 90°]
(∵ opposite side of parallelogram)
(A-A-S condition)

As ΔAPD & ΔCPD are on the same base DP
∴ Their heights are equal.
∴ AE = CF
∴ ΔAPD = ΔCPD Proved

Question 11. AD and BE are the medians of triangle ABC. Let’s prove that ΔACD = ΔBCE

Solution:

 

 

In ΔABC, AD & BE are the medians.

To prove: ΔACD = ΔBCE

Proof: In ΔABC is the median.

∴ ΔACD = ΔABD = \(\frac{1}{2}\) ΔABC

Again, In ΔABC, BE is the median.

∴ ΔBCE = \(\frac{1}{2}\) ΔABC

∴ ΔACD = ΔBCE Proved

Question 12. A line parallel to BC of triangle ABC intersects sides AB and AC at the points P and Q respectively. CQ and BQ intersect each other at the point X. Let’s prove that:

1. ΔBPQ= ΔCPQ
2. ΔBCP = ΔBCQ
3. ΔACP= ΔABQ
4. ΔBXP = ΔCXQ

 

 

Solution: To prove

1. ΔBPQ= ΔCPQ
2. ΔBCP = ΔBCQ
3. ΔACP= ΔABQ
4. ΔBXP = ΔCXQ

Proof: ΔBPQ & ΔCPQ are on the same base PQ & in between two parallel lines PQ & BC.
∴ ΔBPQ = ΔCPQ ….(1)

ΔBCP & ΔBCQ are on the same base BC & in between two parallel lines PQ & BC.
∴ ΔBCP = ΔBCQ …(2)

∵ ΔBPQ = ΔCPQ (Proved)
∴ ΔBPQ+ΔAPQ = ΔCPQ + ΔAPQ

∴ ΔABQ = ΔACP
∴ ΔACP = ΔABQ

∵ ΔBPQ = ΔCPQ
∴ ΔBPQ – ΔPQX = ΔCPQ = ΔPQX

∴ ΔBXP = ΔCXQ …..(4) Proved

Question 13. D is the mid-point of BC of triangle ABC and P is any point on BC. Join P,A. Through the point D a straight line parallel to line segment PA meets AB at point Q. Let’s prove that:

1. ΔADQ= ΔPDQ
2. ΔBPQ= \(\frac{1}{2}\) ΔABC.

 

 

Solution: D is the mid-point of BC of triangle ABC and P is any point on BC. P and A are joined. Through the point D a straight line parallel to line segment PA meets AB at point Q.

To prove :
1. ΔADQ= ΔPDQ
2. ΔBPQ= \(\frac{1}{2}\) ΔABC.

Proof: ΔADQ and ΔPDQ lie on the same base DQ and between the same parallel straight lines DQ and AP.

∴ ΔADQ ΔPDQ
∴ ΔADQ = ΔPDQ ….(1)

∴ ΔADQ+ΔBDQ = ΔPDQ + ΔBDQ
∴ ΔABD = ΔBPQ

∵ AD is the median of ΔABC.

∴ ΔΑΒD = \(\frac{1}{2}\) ΔΑΒC

∴ ΔBPQ = \(\frac{1}{2}\) ΔABC ….(2)

Question 14. In triangle ABC of which AB = AC; perpendiculars through the points B and Con sides AC and AB intersect sides AC and AB at the points E and F. Let’s prove that FE II BC.

 

 

Solution: To prove FE II BC
Join E, F.

Proof: In ΔABC, AB = AC

∴ ∠ABC = ∠ACB
∴ ∠FBC= ∠ECB

In ΔBCF & ΔBCE
∠FBC=∠ECB Proved
∠BFC = ∠BEC (= 90°)& BC is the common base

∴ ΔBCF ≅ ΔBCE (A-A-S condition)
∴ Area of ΔBCF = Area of ΔBCE

∴ ΔBCF & ΔBCE are on the same base BC & their areas are equal.
∴ The triangles are in between two parallel lines.
∴ FE II BC Proved

Question 15. In triangle ABC, ∠ABC= ∠ACB; bisectors of an angle ∠ABC and ∠ACB intersect the sides AC and AB at the points E and F respectively. Let’s prove that FE II BC.

 

 

Solution: To prove FE II BC

Join: E, F

Proof: In ΔABC, ΔABC ΔACB

∴ \(\frac{1}{2}\) ∠ABC = \(\frac{1}{2}\) ∠ACB

∴ ∠EBC = ∠FCB

In ΔBCF & ΔBCE
∠FBC =∠ECB  (∵∠ABC = ∠ACB)
∠FCB = ∠EBC (Proved) & BC is the common side.

∴ ΔBCF ≅ ΔBCE (A-A-S Condition)
∴ Area of ΔBCF = Area ΔBCE

∴ ΔBCF & ΔBCE on the same BC & their areas are equal.
∴ The triangles are in between two parallel lines.
∴ FE II BC

Question 16. The shape of two parallelograms ABCD and AEFG, of which ∠A is common, are equal in area and E lies on AB. Let’s prove that DE II FC.

 

 

 

Solution: To prove DE II FC.

Join: D, E; E, C & C, F.

Proof: ΔDEC and parallelogram ABCD are on the same base & in between two parallel lines AB & CD.

∴ ΔDEC = \(\frac{1}{2}\) parallelogram □ABCD

Again, ΔDEF & parallelogram AEFG are on the same base & in between two parallel lines EF & AG.

∴ ΔDEF = \(\frac{1}{2}\) parallelogram □AEFG

∵ parallelogram □ABCD = parallelogram □AEFG (given)

∴ \(\frac{1}{2}\) parallelogram □ABCD = \(\frac{1}{2}\) parallelogram □AEFG

∴ ΔDEC = ΔDEF
ΔDEC & ΔDEF are on the same base DE & their areas are equal.
∴ DE II FC Proved

Question 17. ABCD is a parallelogram and ABCE is a quadrilateral. Diagonal AC divides the quadrilateral field ABCE into two equal parts. Let’s prove that AC II DE.

 

 

Solution: To prove  AC II DE.
Join D, E.

Proof: Diagonal AC bisects quadrilateral ABCD in two equal parts.
∴ Area of AABC = Area of AACE

Again, in the triangle ABC,
∴ Area of ΔABC = Area of ΔADC
∴ Area of ΔADC = Area of ΔACE

∴ ΔADC & ΔACE are of equal area & on the same base.
∴ Triangles are in between two parallel lines.
∴ AC II DC Proved

Question 18. D is the mid-point of side BC of triangle ABC; P and Q lie on sides BC and BA in such a way that ΔBPQ = \(\frac{1}{2}\) ΔABC. Let’s prove that, DQ II PA.

 

 

Solution: To prove  DQ II PA.
Join A, D.

Proof: In ΔABC, D is the mid-point of BC.
∴ AD is the median.

∴ ΔABD = \(\frac{1}{2}\) ΔABC

But ΔBPQ = \(\frac{1}{2}\) ΔABC (given)

∴  ΔABD = ΔBPQ
∴  ΔABD-ΔBDQ= ΔBPQ-ΔBDQ
∴ ΔADQ = ΔDPQ

∴  ΔADQ & ΔDPQ are of equal area and on the same base DQ.
∴  DQ II PA Proved

Question 19. Parallelogram ABCD, of which mid-points of sides AB, BC, CD are E, F, G and H, and DA respectively. Let’s prove that:

1. EFGH is a parallelogram.
2. Area of the shape of parallelogram EFGH is half of the area of the shape of parallelogram ABCD.

 

 

Solution: To prove EFGH is a parallelogram.

Join: A, C

Proof:

1. In ΔABC, E & F are the mid-points of AB & AC.

∴ EF II AC & EF = \(\frac{1}{2}\) AC …….(1)

Again, in ΔADC, the mid-points of AD & CD are H & G.

∴ HG II AC & HG=\(\frac{1}{2}\) AC …….(2)

From (1) & (2), EF II HG & EF = HG
∴ EFGH is a parallelogram.

2. O is the midpoint of AC. Join H, O & G, O. HE & GF cut AC at P & Q. In ΔDAC joining the mid-points of the sides AD, AC & CD, AHOG is formed.

∴ ΔHOG = \(\frac{1}{4}\) ΔABC

∵ HG II PQ

∴ ΔHOG = \(\frac{1}{2}\) parallelogram □HPQG

[ ∵ ΔHOG & parallelogram □HPQG are on the same base HG and in between two parallel lines HG & PQ]

∴ parallelogram □HPQG = 2ΔHOG = 2 x \(\frac{1}{4}\) ΔACD = \(\frac{1}{2}\) ΔACD

Similarly, parallelogram □EPQF = \(\frac{1}{2}\) ΔABC

∴ parallelogram □HPQG + parallelogram □EPQF = \(\frac{1}{2}\) ΔACD + \(\frac{1}{2}\) ΔABC

∴ parallelogram □EFGH = \(\frac{1}{2}\) ( ΔACD+ ΔABC) = \(\frac{1}{2}\) parallelogram □ABCD ….(2) Proved

Question 20. In of a trapezium ABCD, AB II DC and E is mid-point of BC. Let’s prove that area of triangular field AED = \(\frac{1}{2}\) Χ area of the shape of the trapezium field ABCD.

 

 

Solution: In trapezium ABCD, AB II CD and E is the mid-point of BC.

Let’s prove that in the triangular field = area \(\frac{1}{2}\)  x ABCD of the trapezium field.

Construction: From point E parallel to AD a straight line is drawn which cuts DC at P and extended AB at Q. A, and E are joined.

Proof: ΔAED & parallelogram □ADPQ are on the same base AD & in between two parallel lines AD and PQ.

∴ Area of ΔAED = \(\frac{1}{2}\) parallelogram □ADPQ

In ΔPEC & ΔBEQ,
∠PCE = alternate ∠EBQ

∠PEC (vertically opposite angle) ∠BEQ & CE = BE (∵ E is the midpoint of BC)

∴ ΔPEC ≅ ΔBEQ (A-A-S Condition)
∴ Area of ΔPEC = Area ΔBEQ

Area of ABCD trapezium = Area ADPEB + Area ΔPEC
= Area of ADPEB + Area of ABEQ
= Area □ADPQ
= Area 2x ΔAED

∴ 2 x area ΔAED = Area of ABCD trapezium

∴ Area ΔAED = \(\frac{1}{2}\) Χ Area of ABCD trapezium

Question 21. Multiple choice questions

1. D, E, and F are mid-points of sides BC, CA, and AB respectively of a triangle ABC. If AABC= 16 sq. cm, then the area of the shape of trapezium FBCE is

 

 

1. 40 sq. cm
2. 8 sq. cm
3. 12 sq. cm
4. 100 sq. cm

Solution: Join B, E.

∵ BE is the median of ΔABC.

∴ Area ΔABE = Area ΔBEC = Area \(\frac{1}{2}\) ΔABC

= \(\frac{1}{2}\) x 16 sq cm= 8 sq cm

∵ EF is the median of ΔABE

∴ Area ΔBEF= Area \(\frac{1}{2}\) ΔABE

= \(\frac{1}{2}\) x 8 sq cm = 4 sq cm
Area FBCE = Area ΔBEF + Area ΔBCE = (4+8) sq. cm = 12 sq. cm

Solution: 3.12 sq. cm

2.  A, B, C, and D are the mid points of sides PQ, QR, RS, and SP respectively of parallelogram PQRS. If the area of the shape of the parallelogram PQRS = 36 sq. cm then the area of the ABCD field is

 

 

1. 24 sq. cm
2. 18 sq. cm
3. 30 sq. cm
4. 36 sq. cm

Solution: We know the area of the parallelogram formed by joining the midpoints of a parallelogram is half of that parallelogram.

∴ Area of ABCD = \(\frac{1}{2}\) Χ Area of parallelogram □PQRS

= \(\frac{1}{2}\) x 36 sq. cm = 18 sq. cm

Solution: 2. 18 sq. cm

3. O is any point inside parallelogram ABCD. If AAOB +ACOD = 16 sq. cm, then area of the shape of parallelogram ABCD is

 

 

1. 8 sq. cm
2. 4 sq. cm
3. 32 sq. cm
4. 64 sq. cm

Solution: The straight line through O & parallel to AB intersects AD & BC at R & S points respectively.

∴ ΔΑΟΒ = \(\frac{1}{2}\) parallelogram □ARSB

∴ ΔCOD = \(\frac{1}{2}\) parallelogram □CSRD

∴ ΔAOB + ΔCOD = \(\frac{1}{2}\) (parallelogram □ARSB+ parallelogram □CSRD)

∴ ΔAOB+ ΔCOD = \(\frac{1}{2}\) Χ parallelogram □ABCD

or, 16 sq. cm = \(\frac{1}{2}\) parallelogram □ABCD
∴ Area of parallelogram ABCD = 2 x 16 sqcm = 32 sqcm

Solution: 3. 32 sq. cm

4. D is the mid-point of side BC of triangle ABC. E is the mid-point of side BD and O is the mid-point of AE; the area of triangular field BOE is

 

 

1. \(\frac{1}{3}\) Χ Area of ΔABC

2. \(\frac{1}{4}\) Χ Area of ΔABC

3. \(\frac{1}{6}\) Χ Area of ΔABC

4. \(\frac{1}{8}\) Χ Area of ΔABC

Solution:

Area of the triangular area BOE = \(\frac{1}{2}\)  x area ΔABE [ ∵BO is the median of ΔABE]

\(\frac{1}{2}\) x \(\frac{1}{2}\) x area ABC (∵ In ΔABE, BO is the median)

\(\frac{1}{2}\) x \(\frac{1}{2}\) x area ABD (∵ In ΔABD, AE is the median)

=\(\frac{1}{2}\) X \(\frac{1}{2}\) X \(\frac{1}{2}\) x area ABC (∵ In ΔABC, AD is the median)

= \(\frac{1}{8}\) X ABC

Solution: 4. \(\frac{1}{8}\) X Area of ΔABC

5. A parallelogram, a rectangle, and a triangle stand on the same base and between the same parallel, and if their areas are P, Q, and T respectively then

 

 

1. P=R=2T
2. P=R= \(\frac{T}{2}{/latex]
3. 2P = 2R = T
4. P=R=T

Solve: Let parallelogram ABCD, rectangle ABEF and AABG are situated on same base AB and in between parallel straight lines AB and FC.

Area of parallelogram □ABCD = Area of rectangle ABEF =2 x area ΔAGB
∴ P = R = 2T

Solution: 1. P=R=2T

Question 22. Short answer type questions :

1. DE is perpendicular on side AB from point D of parallelogram ABCD and BF is perpendicular on side AD from the point B; if AB = 10 cm, AD = 8 cm, and DE = 6 cm, let us write how much is length of BF.

 

 

Solution: Area of parallelogram ABCD = Base x height = 10 x 6 sq. cm
= 60 sq. cm

∴ BF is the height of parallelogram □ABCD
∴ ADX BF = Area of parallelogram □ABCD
or, 8 x BF 60 cm

or, BF = [latex]\frac{60}{8}\) sq. cm

or, BF = 7.5 cm
∴ Length of BF = 7.5 cm

2. The area of the shape of parallelogram ABCD is 100 sq units. P is the mid-point of side BC; let us write how much is the area of the triangular field ABP.

 

 

Solution: Join, A, P, and A, C.

Area of ΔABP = Area of \(\frac{1}{2}\)× ΔABC (: AP is the median of ΔABC)

\(\frac{1}{2}\) x \(\frac{1}{2}\) Χ ABCD Area of parallelogram

= \(\frac{1}{4}\) x 100 sq.cm = 25 sq.cm

3. AD is the median of triangle ABC and P is any point on side AC in such a way that area of ΔADP area of ΔABD = 2: 3. Let us write the ratio, area of ΔPDC area of ΔABC.

 

 

Solution: Let the area of ΔADP & ΔABD be 2x sq. unit & 3x sq. unit respectively.

∵ AD is the median.

∴ Area of ΔACD = Area of ΔABD = 3x sq. cm
∴  Area of ΔPDC = Area of ΔACD = Area of ΔADP
=(3x-2x) sq.cm = x sq. cm

Area of ΔABC = Area of ΔABD + Area of ΔACD =(3x + 3x) sq.cm = 6x sq. cm
∴ Area of ΔPDC: Area of ΔABC =x:6x = 1:6

4. ABDE is a parallelogram. F is the midpoint of side ED. If the area of the triangular field ABD is 20 sq. unit, then let us write how much is an area of the triangular field AEF.

 

 

Solution: ∵ AD is the diagonal of the parallelogram ABDE.
∴ Area of ΔAED = Area of ΔABD = 20 cm

∴ In ΔAED, is the median AF,

∴  Area of ΔAEF = Area of \(\frac{1}{2}\) ΔAED

= \(\frac{1}{2}\) x 20 sq.cm = 10 sq.cm.

5. PQRS is parallelogram. X and Y are the mid-points of sides PQ and SR respectively. Construct diagonal SQ. Let us write the area of the shape of parallelogram field XQRY: area of triangular field QSR.

 

 

Solution: X & Y are the mid-points of PQ & SR respectively.

∴ Area of parallelogram □XQRY = \(\frac{1}{2}\) of Area of parallelogram PQRS

∵ SQ is the diagonal of the parallelogram PQRS.

∴ Area of ΔQSR = \(\frac{1}{2}\) x Area of the parallelogram PQRS

∴ Area of parallelogram □XQRY = Area of ΔQSR
∴ Area of the parallelogram XQRY: Area of ΔQRS = 1:1

 

 

Class IX Maths Solutions WBBSE Chapter 4 Co-ordinate Geometry: Distance Formula Let Us Work Out

Question 1. I measure the length of the straight line joining the following pairs of points

1. (18, 0); (8, 0)

Solution: Let P and Q be two points whose coordinates are (18,0) and (8,0). Points P and Q are situated in the positive direction of the x-axis.

∴ \(\overline{\mathrm{PQ}}=(\overline{\mathrm{OP}}-\overline{\mathrm{OQ}})\) = (18 – 8) unit = 10 units.

Read and Learn More WBBSE Solutions For Class 9 Maths

 

 

∴ The length of the straight line \(\overline{\mathrm{PQ}}\) = 10 units.

∴ Length of the line segment by joining two points is 10 units.

2. (0, 15); (0, 4)

Soltion: Let P and Q are two points whose coordinates are (0, 15) and (0, 4). P and Q are situated in the positive direction of the y-axis.

∴ \(\overline{\mathrm{OP}}\) =15 unit

∴ \(\overline{\mathrm{OQ}}\)= 4 unit

 

 

∴ \(\overline{\mathrm{PQ}}=(\overline{\mathrm{OP}}-\overline{\mathrm{OQ}}))\) = (15 – 4) units = 11 units.

∴ The length of the straight line PQ is 11 units.
∴ The length of the line segment formed by joining the two points is 11 units.

3. (-7, 0) (-2, 0)

Solution: Let P and Q are two points whose coordinates are (-7, 0) and (-2, 0). Points P and Q are situated in the negative direction of the x-axis.

∴ \(\overline{\mathrm{OP}}\) = 7 units

∴\(\overline{\mathrm{OQ}}\)= 2 units

 

 

∴ \(\overline{\mathrm{PQ}}=\overline{\mathrm{QP}}=\overline{\mathrm{OP}}-\overline{\mathrm{OQ}}\) = (7-2) units = 5 units

∴ Length of straight line PQ is 5 units.
∴ The length of the straight line formed by joining the two points is 5 units.

Class 9 Mathematics West Bengal Board

4. (0, -10) (0, -3)

Solution: Let P and Q are two points whose coordinates are: (0, -10) and (0, -3).

∴ \(\overline{\mathrm{OP}}\) = 10 units

∴ \(\overline{\mathrm{OQ}}OQ\) = 3 units

 

 

 

\(\overline{\dot{\mathrm{PQ}}}=\overline{\mathrm{QP}}=\overline{\mathrm{OP}}-\overline{\mathrm{OQ}}\) = (10 – 3) units = 7 units.
∴ Length of straight line PQ is 7 units.
∴ The length of the line segment formed by joining the two points is 7 units.

5. (6, 0) (-2, 0)

Solution: Let P and Q are two points whose coordinates are: (6, 0) and (-2, 0). Point P is situated in the positive direction and point Q is situated in the negative direction of x-axis.

∴ \(\overline{\mathrm{OP}}\) = 6 units

∴ \(\overline{\mathrm{OQ}}\) = 2 units

 

 

\(\overline{\mathrm{PQ}}=\overline{\mathrm{QP}}=(\overline{\mathrm{OP}}+\overline{\mathrm{OQ}})\) = (6 + 2) units = 8 units.

∴ Length of straight line PQ is 8 units.
∴ The length of the line segment formed by joining the two points is 8 units.

Class 9 Mathematics West Bengal Board

6. (0,-5) (0, 9)

Solution: Let P and Q are two points whose co-ordinates are: (0, -5) and (0, 9). Point P is situated in the negative direction and point Q is situated in the positive direction of y-axis.

∴ \(\overline{\mathrm{OP}}\) = 5 units

∴ \(\overline{\mathrm{OQ}}OQ\)=9 units

 

 

∴ \(\overline{\mathrm{PQ}}=\overline{\mathrm{QP}}=(\overline{\mathrm{OP}}+\overline{\mathrm{OQ}})\) = (5+9) units = 14 units.

∴ Length of the straight line PQ is 14 units.
∴ The length of the line segment formed by joining the two points is 14 units.

7. (5, 0): (0, 10)

Solution: Let P and Q are two points whose coordinates are (5, 0) and (0, 10)
∴OP = 5 units
OQ = 10 units

 

 

In right angled ΔPOQ from Pythagoras’ theorem,

PQ² = OP² + OQ²
or, PQ² = (5)² + (10)² sq.units
or, PQ² = 25+ 100 sq.units
or, PQ² = 125 sq.units

∴ PQ= √125 units = 5√5 units
∴ Distance between points P and Q is 5√5 units.
∴ The length of the line segment formed by joining the two points is 5√5 units.

Class 9 Mathematics West Bengal Board

8. (3,0): (0, 4)

Solution: Let P and Q be two points whose co-ordinates are (3, 0) and (0, 4).

∴ OP = 3 units
OQ = 4 units

 

 

In right-angled APOQ we get by Pythagoras’ theorem,

PQ² = OP²+OQ²
or, PQ² = (3)² + (4)² sq.units
or, PQ² = 9 + 16 sq.units
or, PQ² = 25 sq.units

∴ PQ= √25 units = 5 units
∴ Distance between points P and Q is 5 units.
Length of the straight line formed by joining the two points is 5 units.

Class 9 Maths WB Board

9. (4,3); (2, 1)

Solution: Let A and B are two points whose co-ordinates are (2, 1) and (4, 3).
In Image, OM = 2 units and AM = 1 units.
ON 4 units and BN = 3 units.

∴ AP = MN = ON-OM = (4-2) units = 2 units
Again, BP= BN-PN = (3-1) units = 2 units

 

 

In right angled Δ APB we get by Pythagoras’ theorem,

AB² = AP² + BP²
or, AB² = (2)² + (2)² sq.units
or, AB² = 4+4 sq.units
or, AB² = 8 sq.units

∴ AB = √8 units = 2√2 units
∴ Distance between the points A and B, i.e., length of the straight line formed by lining the two points is 2√2 units.

Class 9 Maths WB Board

10. (-2,-2) (2, 2)

Solution: Let A and B are two points whose co-ordinates are (-2, -2) and (2, 2).
∴ A point lies in third quadrant. B lies in first quadrant. From points A and B on axis respectively AP and BQ perpendiculars are drawn which cut x-axis at points p and Q respectively.
∴ OP 2 units and OQ = 2 units.
BQ 2 units and AP 2 units

 

 

From point A on extended-BQ a perpendicular is drawn which cuts extended BQ at point D.
∴ AD = PQ = OP + OQ
(2+2) units = 4 units
BD = BQ + QD = BQ + PA (2+2) units = 4 units

In right angled AADB we get by Pythagoras’ theorem, AB2

AD²+ BD²
or, AB² = (4)²+(4)² sq.units
or, AB² = 16+ 16 sq.units
or, AB² = 32 sq.units

∴ AB = √32 units = 4√√2 units
∴ Length of the straight line AB is 4√2 units, i.e., the length of the straight line formed by joining the two points is 4√2 units.

 

Class 9 Mathematics West Bengal Board Chapter 4 Co-ordinate Geometry: Distance Formula Exercise 4.1

 

Question 1. Let us calculate the distances of the following points from the origin

Here, distance formula = \(\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2}\)unit.

1. (7, -24)

Solution: The distance of the point (7,-24) from the origin is

\(\begin{aligned}
D & =\sqrt{(7-0)^2+(-24-0)^2} \text { unit } \\
& =\sqrt{49+576} \text { unit } \\
& =\sqrt{625} \text { unit } \\
& =25 \text { unit }
\end{aligned}\)

 

2. (3,-4)

Solution:The distance of the point (3, 4) from the origin (0,0) is

\(\begin{aligned}
D & =\sqrt{(3-0)^2+(-4-0)^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

3. (a+b, a-b)

Solution: The distance of the point (a+b, a-b) from the origin (0,0) is

\(\begin{aligned}
& D=\sqrt{(a+b-0)^2+(a-b-0)^2} \text { unit } \\
& =\sqrt{(a+b)^2+(a-b)^2} \text { unit } \\
& =\sqrt{2\left(a^2+b^2\right)} \text { unit }
\end{aligned}\)

 

Question 2. Let us calculate the distances between the pairs of points given below:

1. (5, 7) and (8, 3)

Solution: The distance between (5, 7) and (8, 3) is

\(\begin{aligned}
& =\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2} \text { unit } \\
& D=\sqrt{(5-8)^2+(7-3)^2} \text { unit } \\
& =\sqrt{-3^2+4^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

2. (7,0) and (2,-12)

Solution: Distance between (7, 0) and (2,-12) is

\(\begin{aligned}
& =\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2} \text { unit } \\
& D=\sqrt{(5-8)^2+(7-3)^2} \text { unit } \\
& =\sqrt{-3^2+4^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

 

3. \(\left(-\frac{3}{2}, 0\right)\) and (0, -2)

Solution: Distance between \(\left(-\frac{3}{2}, 0\right)\) and (0, -2)

 

\(=\sqrt{\left(\frac{-3}{2}-0\right)^2+(0+2)^2} \text { unit }\)

 

\(\begin{aligned}
& =\sqrt{\frac{9}{4}+4} \text { unit } \\
& =\sqrt{\frac{9+16}{4}} \text { unit } \\
& =\sqrt{\frac{25}{4}} \text { unit } \\
& =\frac{5}{2} \text { unit } \\
& =2.5 \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

4. (3, 6) and (-2, -6)

Solution: Distance between (3, 6) and (-2, -6) is

\(\begin{aligned}
& D=\sqrt{(3+2)^2+(6+6)^2} \text { unit } \\
& =\sqrt{25+144} \text { unit } \\
& =\sqrt{169} \text { unit } \\
& =13 \text { unit }
\end{aligned}\)

 

5. (1,-3) and (8,3)

Solution: Distance between (1,-3) and (8,3) is

\(\begin{aligned}
& =\sqrt{(1-8)^2+(-3-3)^2} \text { unit } \\
& =\sqrt{7^2+6^2} \text { unit } \\
& =\sqrt{49+36} \text { unit } \\
& =\sqrt{85} \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

6. (5,7) and (8,3)

Solution: The distance between the points (5, 7) and (8, 3) is

\(\begin{aligned}
D & =\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2} \text { unit } \\
& =\sqrt{(5-8)^2+(7-3)^2} \text { unit } \\
& =\sqrt{3^2+4^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

 

Question 3. Let us prove that the point (-2, -11) is equidistant from the two points (3,7) and (4,6).

Solution: The distance (D₁) between the points (-2, -11) and (-3, 7) is

\(\begin{aligned}
& =\sqrt{(-2+3)^2+(-11-7)^2} \text { unit } \\
& =\sqrt{1^2+(-18)^2} \text { unit } \\
& =\sqrt{1+324} \text { unit } \\
& =\sqrt{325} \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

And the distance (D) between the points (-2, -11) and (4, 6) is

\(\begin{aligned}
& =\sqrt{(-2-4)^2+(-11-6)^2} \text { unit } \\
& =\sqrt{(-6)^2+(-17)^2} \text { unit } \\
& =\sqrt{36+289} \text { unit } \\
& =\sqrt{325} \text { unit }
\end{aligned}\)

 

Here, D₁ = D₂
∴ The point (-2, -11) is equidistant to the two points (-3, 7) and (4, 6). Proved

Question 4. By calculation let us show that the points (7, 9), (3, -7), and C (-3, 3) are the vertices of a right-angled triangle.

Solution: Let the points be A = (7, 9), B = (3, -7), and C = (-3, 3).

 

 

\(\begin{aligned}
\text { Distance } A B & =\sqrt{(7-3)^2+(9+7)^2} \text { unit } \\
& =\sqrt{16+256} \text { unit } \\
& =\sqrt{272} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

\(\begin{aligned}
\text { Distance } B C & =\sqrt{(3+3)^2+(-7-3)^2} \text { unit } \\
& =\sqrt{36+100} \text { unit } \\
& =\sqrt{136} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance } A C & =\sqrt{(7+3)^2+(9-3)^2} \text { unit } \\
& =\sqrt{100+36} \text { unit } \\
& =\sqrt{136} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

Here, BC²+ CA² = 136+ 136 = 272 & AB² = 272
∴ BC²+ CA²= AB²
Δ ABC is a right angled triangle, right angle being at ∠C.

Question 5. Let us prove that in both of the following cases, the three points are the vertices of an isosceles triangle:

1. (1, 4), (4, 1), and (8, 8)
   
   Solution: Let A = (1,4); B = (4,1) & C = (8,8)

\(\begin{aligned}
\text { Distance } A B & =\sqrt{(1-4)^2+(4-1)^2} \text { unit } \\
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance AC } & =\sqrt{(1-8)^2+(4-8)^2} \text { unit } \\
& =\sqrt{49+16} \text { unit } \\
& =\sqrt{65} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

\(\begin{aligned}
\text { Distance } B C & =\sqrt{(4-8)^2+(1-8)^2} \text { unit } \\
& =\sqrt{16+49} \text { unit }
\end{aligned}\)

∴ In ΔABC AC= BC
∴ ABC is an isosceles triangle. Proved

2. (-2,-2), (2, 2) and (4,-4)

Solution:Let P= (-2,-2), Q=2, 2) and R = (4,-4)

\(\begin{aligned}
\text { Distance } P Q & =\sqrt{(-2-2)^2+(-2-2)^2} \text { unit } \\
& =\sqrt{4^2+4^2} \text { unit } \\
& =\sqrt{16+16} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance } Q R & =\sqrt{(2-4)^2+(2+4)^2} \text { unit } \\
& =\sqrt{(2)^2+(6)^2} \text { unit } \\
& =\sqrt{4+36} \text { unit } \\
& =\sqrt{40} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance PR } & =\sqrt{(-2-4)^2+(-2+4)^2} \text { unit } \\
& =\sqrt{(-6)^2+(2)^2} \text { unit } \\
& =\sqrt{36+4} \text { unit } \\
& =\sqrt{40} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

∴ In triangle PQR, QR = PR= √40
∴ ΔPQR is an isosceles triangle. Proved

Question 6. Let us prove that the three points A (3, 3), B (8,-2), and C (-2,-2) are the vertices of a right-angled triangle. Let us calculate the length of the hypotenuse of ΔABC.

Solution: Given, A (3,3); B = (8,-2); C = (-2,-2)

AB² = (38)² + (3 + 2)² = 5² +5² = 25+ 25 = 50

AC² = (3 + 2)² + (3 + 2)² = 5² +5² = 25+ 25 =50

BC² = (8+ 2)² + (-2 + 2)² = 10² +0 =100+ 0 = 100

∴ AB²+ AC² = 50+50 = 100∴ Δ ABC is right angled triangle and Hypotenuse BC= √100 = 10 units.

Question 7. Let us show by calculation that the points (2, 1), (0, 0), (-1, 2), and (1, 3) are the angular points of a square.

Solution: A = (2, 1), B = (0, 0), C (-1, 2), and D = (1, 3).

 

 

\(Distance A B=\sqrt{(2-0)^2+(1-0)^2}  units =\sqrt{4+1} units =\sqrt{5} units\)

 

\(Distance B C=\sqrt{(0+1)^2+(0-2)^2}  units =\sqrt{1+4} units =\sqrt{5} units\)

Class 10 Math Solution WBBSE Chapter 4

\(\begin{aligned}
\text { Distance CD } & =\sqrt{(-1-1)^2+(2-3)^2} \text { units } \\
& =\sqrt{4+1} \text { units } \\
& =\sqrt{5} \text { units }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance DA } & =\sqrt{(1-2)^2+(3-1)^2} \text { units } \\
& =\sqrt{1+4} \text { units } \\
& =\sqrt{5} \text { units }
\end{aligned}\)

 

\(Again, Diagonal A C=\sqrt{(2+1)^2+(1-2)^2} units =\sqrt{9+1} units =\sqrt{10} units\)

Class 10 Math Solution WBBSE Chapter 4

\(\begin{aligned}
\text { and Diagonal BD } & =\sqrt{(0-1)^2+(0-3)^2} \text { units } \\
& =\sqrt{1+9} \text { units } \\
& =\sqrt{10} \text { units }
\end{aligned}\)

 

∴ In the quadrilateral ABCD all the sides are equal and diagonals AC = BD.
∴ ABCD is a square. Proved

Question 8. Let us calculate and see that for what value of y, the distance between the two points (2,y) and (10, -9) will be 10 units.

Solution: Distance of the points (2, y) from the point (10, -9)= 10 units.

∴ \(\begin{aligned}
& \sqrt{(2-10)^2+(y+9)^2}=10 \text { (given) } \\
& \sqrt{(-8)^2+(y+9)^2}=10
\end{aligned}\)

Class 10 Math Solution WBBSE Chapter 4

or, 64 +(y+9)²= 100 (Squaring both sides)
or, (y+9) = 100 -64 =36
∴ y+9 = ±6

(1) y+9=6
y=6-9=-3 and

(2) y+9=-6
∴ y-6-9=-15

Question 9. Let us find a point on x-axis which is equidistant from the two points (3,5) and (1,3).

Hints the required point on x-axis is (x,0) = (x-3)² + (0-5)² = (x-1)² + (0-3)²

Solution: Let the point on the x-axis is (x, 0).
∴ Distance of the point (3, 5) from the point (x,0) is

\(\begin{aligned}
& =\sqrt{(x-3)^2+(0-5)^2} \text { unit } \\
& =\sqrt{(x-3)^2+5^2} \text { unit }
\end{aligned}\)

Class 10 Math Solution WBBSE Chapter 4

and the distance of the point (1,3) from the point (x, 0) is

\(\begin{aligned}
& =\sqrt{(x-1)^2+(0-3)^2} \text { unit } \\
& =\sqrt{(x-1)^2+3^2} \text { unit }
\end{aligned}\)

According to the condition, \(\sqrt{(x-3)^2+25}=\sqrt{(x-1)^2+9}\)

or,(x -3)² + 25 = (x -1 )² +9
or, (3 – x)² – (1 – x) ² = 9 – 25
or, (3-x+1-x) (3-x-1+x)=-16
or, (4-2x) x 2 = -16
or, 2(2 – x) x2 = -16
or, 2 – x = -4
or, 2 + 4 = x
∴ X = 6
∴ The required point is (6,0).

Class 10 Math Solution WBBSE Chapter 4

Question 10. Let us write by calculation whether the three points O(0, 0), A(4, 3), and B (8, 6) are collinear.

Hints: If OA + AB = OB, then they are collinear.

Solution:

 

 

\(\begin{aligned}
\text { Diștance } & O A=\sqrt{(0-4)^2+(0-3)^2} \text { unit } \\
= & \sqrt{16+9} \text { unit } \\
= & \sqrt{25} \text { unit } \\
= & 5 \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance } O B & =\sqrt{(0-8)^2+(0-6)^2} \text { unit } \\
& =\sqrt{64+36} \text { unit } \\
& =\sqrt{100} \text { unit } \\
& =10 \text { unit }
\end{aligned}\)

Class 9 Maths WB Board

\(\begin{aligned}
\text { Distance } A B & =\sqrt{(4-8)^2+(3-6)^2} \cdot \text { unit } \\
& =\sqrt{16+9} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Here OA+ AB OB, hence they are collinear.

Question 11. Let us show that the three points (2,2), (-2,-2), and (-2√3,2√3) vertices of an equilateral triangle.

Solution: Let A (2,2); B (-2,-2) and C (-2√3, 2√3).

\(\begin{aligned}
Distance A B & =\sqrt{(2+2)^2+(2+2)^2} \text { unit } \\
& =\sqrt{16+16} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

 

\(\text { Distance } B C=\sqrt{(-2+2 \sqrt{3})^2+(-2-2 \sqrt{3})^2} \text { unit }\)

 

\(\begin{aligned}
& =\sqrt{(2 \sqrt{3}-2)^2+(2+2 \sqrt{3})^2} \text { unit } \\
& =\sqrt{2\left\{(2)^2+(2 \sqrt{3})^2\right\}} \text { unit } \\
& =\sqrt{2(4+12)} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

 

\(\text { Distance } B C=\sqrt{(-2+2 \sqrt{3})^2+(-2-2 \sqrt{3})^2} \text { unit }\) \(\begin{aligned}
& =\sqrt{(2 \sqrt{3}-2)^2+(2+2 \sqrt{3})^2 \text { unit }} \\
& =\sqrt{2\left\{(2)^2+(2 \sqrt{3})^2\right\}} \text { unit } \\
& =\sqrt{2(4+12)} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

∴ AB = BC = CA
∴ A, B, & C are the vertices of an equilateral triangle.

Question 12. Let us show that the points (-7, 2), (19, 8), (15, -6), and (-11, -12) form a parallelogram when they are joined orderly.

Solution: Let A = (-7,2); B = (19,8); C = (15,-6); D = (-11, -12).

 

 

\(The length of A B=\sqrt{(-7-19)^2+(2-8)^2} unit
\begin{aligned}
& =\sqrt{(-26)^2+(-6)^2} \text { unit } \\
& =\sqrt{676+36} \text { unit } \\
& =\sqrt{712} \text { unit }
\end{aligned}
\)

 

\(\begin{aligned}
\text { Length of } B C & =\sqrt{(19-15)^2+(8+6)^2} \text { unit } \\
& =\sqrt{(4)^2+(14)^2} \text { unit } \\
& =\sqrt{16+196} \text { unit } \\
& =\sqrt{212} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Length of } C D & =\sqrt{(15+11)^2+(-6+12)^2} \text { unit } \\
& =\sqrt{(26)^2+(6)^2} \text { unit } \\
& =\sqrt{26^2+6^2} \text { unit } \\
& =\sqrt{676+36} \text { unit }
& = \sqrt{712} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Length of } D A & =\sqrt{(-11+7)^2+(-12-2)^2} \text { unit } \\
& =\sqrt{(-4)^2+(14)^2} \text { unit } \\
& =\sqrt{4^2+14^2} \text { unit } \\
& =\sqrt{16+196} \text { unit } \\
& =\sqrt{212} \text { unit }
\end{aligned}\)

 

\(Length of Diagonal A C=\sqrt{(-7-15)^2+(2+6)^2} unit
\begin{aligned}
& =\sqrt{(-22)^2+8^2} \text { unit } \\
& =\sqrt{22^2+8^2} \text { unit } \\
& =\sqrt{484+64} \text { unit }
\end{aligned}\)

Class 9 Maths WB Board

\(\begin{aligned}
\text { Length of Diagonal } B D & =\sqrt{(19+11)^2+(8+12)^2}=\sqrt{(30)^2+(20)^2} \\
& =\sqrt{900+400} \text { unit } \\
& =\sqrt{1300} \text { unit }
\end{aligned}\)

 

In the quadrilateral ABCD, ABCD and BC = DA [opposite sides are equations]
Diagonal AC ≠ Diagonal BD
∴ ABCD is a parallelogram.

Question 13. Let us show that the points (2,-2), (8, 4), (5, 7), and (-1, 1) are the vertices of a rectangle.

Solution: Let P = (2,-2), Q = (8,4); R = (5,7) and S = (-1,1)
To prove the PQRS is a rectangle.

 

 

 

Here, Diagonal PR = Diagonal QS.
∴ In quadrilateral PQRS, opposite sides are equal, and diagonal PR diagonal
∴ PQRS is a rectangle.

Question 14. Let us show that the points (2,5), (5,9), (9,12), and (6,8) form a rhombus when they are joined orderly.

Solution: Let the points A (2, 5); B (5, 9), C (9, 12), and D (6, 8).

 

 

 

\(\text { Here, } \begin{aligned}
A B= & \sqrt{(2-5)^2+(5-9)^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Class 9 Maths WB Board

\(\begin{aligned}
& B C=\sqrt{(5-9)^2+(9-12)^2} \text { unit } \\
&=\sqrt{16+9} \text { unit } \\
&=\sqrt{25} \text { unit } \\
&=5 \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
& C D=\sqrt{(9-6)^2+(12-8)^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit } \\
&
\end{aligned}\)

 

\(\begin{aligned}
& D A=\sqrt{(6-2)^2+(8-5)^2} \\
&=\sqrt{16+9} \text { unit } \\
&=\sqrt{25} \text { unit } \\
&=5 \text { unit }
\end{aligned}\)

 

∴ AB BC CD = DA

\(Again, Diagonal A C=\sqrt{(2-9)^2+(5-12)^2} unit
\begin{aligned}
& =\sqrt{49+49} \text { unit } \\
& =\sqrt{98} \text { unit }
\end{aligned}
\)

 

\(\begin{aligned}
\text { Diagonal BD } & =\sqrt{(5-6)^2+(9-8)^2} \text { unit } \\
& =\sqrt{1+1} \text { unit } \\
& =\sqrt{2} \text { unit }
\end{aligned}\)

∴ Diagonals are not equal.
∴ In quadrilateral ABCD, all the sides are equal but the diagonals are not equal.
∴  ABCD is a rhombus.

Chapter 4 Co-ordinate Geometry Distance Formula  Multiple Choice Questions

1. The distance between the two points (a + b, c-d) and (a-b, c + d) is

(1)\(2 \sqrt{a^2+c^2}\)
(2)\(2 \sqrt{b^2+d^2}\)
(3)\(\sqrt{a^2+c^2}\)
(4)\(\sqrt{b^2+d^2}\)

Solution: The distance between (a + b, c-d) and (a-b, c + d)

\(\begin{aligned}
& =\sqrt{(a+b-a+b)^2+(c-d-c-d)^2} \text { unit } \\
& =\sqrt{(2 b)^2+(-2 d)^2} \text { unit } \\
& =\sqrt{4 b^2+4 d^2} \text { unit } \\
& =2 \sqrt{b^2+d^2} \text { unit }
\end{aligned}\)

∴(2)\(2 \sqrt{b^2+d^2}\)

2. If the distance between the two points (x, -7) and (3, -3) is 5 units, then the values of x are
(1)0 or 6
(2)2 or 3
(3)5 or 1
(4)-6 or 0

Solution: The distance between (x, -7) and (3,-3)

\(=\sqrt{(x-3)^2+(-7+3)^2} \text { unit }\) \(=\sqrt{(x-3)^2+16} \text { unit }\)

 

By the problem, \(\sqrt{(x-3)^2+16}=5\)

or, (x-3)²+16= (5)² (Squaring both sides)
or, (x-3)²=25-16
or, (x-3)² = 9
or, x-3 = ± 3
or, x = 3+3 or x = 3-3
or, x = 6 or x = 0

∴ (1)0 or 6

3. If the distance of the point (x, 4) from the origin is 5 units, then the values of x are
(1)±4
(2)±5
(3)±3
(4)None of these

Solution: The distance of the point (x, 4) from (0,0)

\(\begin{aligned}
& =\sqrt{\mathrm{x}^2+4^2} \text { unit } \\
& =\sqrt{\mathrm{x}^2+16} \text { unit }
\end{aligned}\)

 

By the problem, \(\sqrt{x^2+16}=5\)
or, x²+16= (5)² (Squaring both sides)
or, x²+16=25
or, x² = 25-16
or, x²= 9
or, x = +3

∴ (3)±3

4. The triangle formed by the points (3, 0), (-3, 0), and (0,3) is
(1)Equilateral
(2)Isosceles
(3)Scalene
(4)Isosceles right-angled.

Solution:  Let the co-ordinates of A, B & C be (3,0) (3,0); & (0,3) respectively.

\(\text { Here, } A B=\sqrt{(2-5)^2+(5-9)^2} \text { unit }\) \(=\sqrt{9+16} \text { unit }\) \(=\sqrt{25} \text { unit }\) \(=5 \text { unit }\)

 

\(\begin{aligned}
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit } \\
& =3 \sqrt{2} \text { unit }
\end{aligned}\)

 

\(\text { Length of } B C=\sqrt{(-3-0)^2+(0-3)^2} \text { unit }
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit } \\
& =3 \sqrt{2}\text { unit }
\end{aligned}\)

 

\(\begin{aligned}
A C & =\sqrt{(3-0)^2+(0-3)^2} \text { unit } \\
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit } \\
& =3 \sqrt{2} \text { unit }
\end{aligned}\)

 

∴ \(B C^2+A C^2=(\sqrt{18})^2+(\sqrt{18})^2\) = 18+ 18 = 36 = 6² = AB²

∴AC = BC and AB² = BC² + AC²
∴ The ΔABC formed by the points (3,0), (-3,0) & (0,3) is an isosceles right-angled triangle.

∴(4)Isosceles right-angled.

5. The co-ordinates of the centre of a circle are (0,0) and the co-ordinates of a point on the circumference are (3,4); the length of the radius of the circle is
(1)5 units
(2)4 units
(3)3 units
(4)None of these.

Solution: Distance between the points (0, 0) and (3, 4) is

\(\begin{aligned}
& =\sqrt{(0-3)^2+(0-4)^2} \text { units } \\
& =\sqrt{9+16} \text { units } \\
& =\sqrt{25} \text { units } \\
& =5 \text { units }
\end{aligned}\)

∴ (1)5 units

Question 16. Short answer type questions:

1. Let us write the value of y if the distance of the point (-4, y) from the origin is 5 units.

Solution:

\(Distance =\sqrt{(-4-0)^2+(0-y)^2} units
\sqrt{16+\mathrm{y}^2}=5 \text { (given) }
\)

 

 or, 16 + y² = 25
∴ y² = 9
∴ y =±3

2. Let us write the co-ordinates of a point on y-axis which is equidistant from the two points (2,3) and (-1,2).

Solution: Let the point on y-axis is (0,b).

\(\begin{aligned}
& =\sqrt{(2-0)^2+(3-b)^2} \\
& =\sqrt{(-1-0)^2+(2-b)^2} \\
& =4+(3-6)^2 \\
& =1+(2-b)^2
\end{aligned}\)

 

∴ b = 4
∴ The point is (0,4).

 

 

Class 9 Math Solution WBBSE Chapter 7 Polynomial Exercise 7

Question 1. If f(x) = x⁵ + 3x³ – 7x² + 6,h(x)=3x³-8x² + 7,g(x) = x + 1,p(x) = x⁴ – x² + 2 and q (y) = 7y³-y+ 10 then let us calculate and write what would be the following polynomials:

1. f(x)+g(x)

Solution: f(x)+g(x) =x⁵+3x³-7x²+6+x+1 =x⁵+3x³-7x²+x+7

2. f(x)-h(x)

Solution: f(x)-h(x) =x⁵+3x³-7x²+6-(3x³-8x²+7) =x⁵+3x³-7x²+6-3x³+8x²-7 = x⁵+x²-1

3. f(x) -p(x)

Solution: f(x)-p(x) =x⁵+3x³-7x²+6-(x⁴-x²+2) =x⁵+3x³-7x²+6-x⁴+x²-2 =x⁵-x⁴+3x³-6x²+4

4. f(x)+p(x)

Answer: f(x)+p(x) =x⁵+3x³-7x²+6+x⁴-x²+2 =x⁵+x⁴+3x³-8x²+8

5. p(x)+g(x)+f(x)

Solution: p(x)+g(x)+f(x) = x⁴-x²+2+x+1+x⁵+3x³-7x²+6 =x⁵+x⁴-3x³-8x²+x+7

Read and Learn More WBBSE Solutions For Class 9 Maths

6. p(x)-q(y)

Solution: p(x)-q(y) =x⁴-x²+2-(7y³-y+10) =x⁴-x²+2-7y³+y-10 =x⁴-7y³-x²+y-8

7. f(x).g(x)

Solution: f(x).g(x) = (x⁵+3x³-7x²+6)(x+1) =x⁶+3x⁴-7x³+6x+x⁵+3x³-7x²+6 =x+x⁵+3x⁴-4x³-7x²+6x+6

8. p(x).g(x)

Solution: p(x).g(x) = (x⁴-x²+2)(x+1) =x⁵-x³+2x+x⁴-x²+2 = x⁵+x⁴-x³-x²+2x+2

Ganit Prakash Class 9 Solutions Chapter 7 Polynomial Exercise 7.1

Question 1. Let us write which are the polynomials in the following algebraic expressions. Let us write the degree of each of the polynomials.

1. 2x⁶ – 4x⁵ +7x² +3

Solution: 2x⁶ – 4x⁵ +7x² +3 is a polynomial because the index of the variable is a whole number and the highest index of x is 6. So the degree of 2x⁶ – 4x⁵ +7x² +3 is 6.

2. x² + 2x^-1 +4

Solution: x² + 2x^-1 +4 is not a polynomial as the index of the variable is not a whole number.

3. \(y^3-\frac{3}{4} y+\sqrt{7}\)

Solution: \(y^3-\frac{3}{4} y+\sqrt{7}\) is a polynomial as the index of the variable is a whole number, and the highest index of y is 3, so the degree of \(y^3-\frac{3}{4} y+\sqrt{7}\) is 3.

4. \(\frac{1}{x}-x+2\)

Solution: \(\frac{1}{x}-x+2\)  is not polynomial as the index of the variable is not a whole number.

5. \(x^{51}-1\)

Solution: \(x^{51}-1\) is a polynomial as the index of the variable is a whole number, and the highest index of x is 51 so the degree of \(x^{51}-1\)  is 51.

6. \(3 \sqrt{t}+\frac{t}{27}\)

Solution: \(3 \sqrt{t}+\frac{t}{27}=t^{\frac{1}{3}}+\frac{t}{27}\) is not a polynomial as the index of the variable is not a whole number.

7. 15

Solution: 15 15.1 15.x° It is a monomial of degree 0.

8. 0

Solution: 0 Power of the polynomial is undefined.

9. \(z+\frac{3}{z}+2\)

Solution: \(z+\frac{3}{z}+2=z+3 z^{-1}+2\) is not a polynomial as the index of the variable is not a whole number.

10. y³+ 4

Solution: y3+4 is not a polynomial as the index of the variable is a whole number. So the degree of y³+ 4 is 3.

11. \(\frac{1}{\sqrt{2}} x^2-\sqrt{2} x+2\)

Solution: \(\frac{1}{\sqrt{2}} x^2-\sqrt{2} x+2\) is not a polynomial as the index of the variable is a whole number. So the degree of \(\frac{1}{\sqrt{2}} x^2-\sqrt{2} x+2\) is 2.

Question 2. In the following polynomials, let us write which are first-degree polynomials in one variable, which are second-degree polynomials in one variable, and which are third-degree polynomials in one variable.

1. 2x + 17

Solution: 2x + 17 One variable – Degree – 1

2. x³ + x² + x + 1

Solution: x³ + x² + x + 1 One variable – Degree – 3

3. – 3+2y²+5xy

Solution: – 3+2y²+5xy is Not one variable here; x & y are two variables.

4. 5-x-x³

Solution: 5-x-x³ One variable – Degree – 3

5. √2+t- t²

Solution: √2+t- t² One variable – Degree – 2

6. √5x

Solution: √5x One variable – Degree – 1

Class 9 Mathematics West Bengal Board

Question 3. Let us write the coefficients of the following polynomials according to the guidelines:

1. The co-efficient of x³ in 5x³ – 13x² + 2

Solution: In 5x³ – 13x² + 2, co-efficient of x3 is 5.

2. The coefficient of x in x²-x+2.

Solution: In x²-x+2, the co-efficient of x is 1.

3. The co-efficient of x² in 8x-19

Solution: In 8x-19= 0x² + 8x-19, co-efficient of x² is 0.

4. The co-efficient of x° in √11-3√11x+x²

Solution: In √11-3√11x+x² = √11x° -3√11x+x², co-efficient of x° is √11.

Question 4. I write the degree of each of the following polynomials :

1. x⁴ + 2x³ +x² + x

Solution: x⁴ + 2x³ +x² + x Degree – 4

2. 7x-5

Solution: 7×5 Degree – 1

3. 16

Solution: 16 = 16.1 16x° Degree – 0

4. 2-y-y³

Solution: 2-y-y³ Degree – 3

5. 7t

Solution: 7t Degree – 1

6. 5 – x² + x¹⁹

Solution: 5 – x² + x¹⁹ Degree – 19

Class 9 Mathematics West Bengal Board

Question 5. I write two separate binomials in one variable whose degrees are 17.

1. 5x¹⁷+1

Solution: 5x¹⁷+1 Binomial with two variables & degree 14.

2. 2y¹⁷-5

Solution: 2y¹⁷-5 Binomial with two variables & degree 17.

Question 6. I write two separate monomials in one variable whose degrees are 4.

1. 2x⁴

Solution: 2x⁴ Monomial with one variable & degree 4.

2. 3y⁴

Solution:: 3y⁴ Monomial with one variable & degree 4.

Question 7. I write two separate trinomials in one variable whose degrees are 3.

1.  2x³ + 3x² + 4x

Solution: 2x³ + 3x² + 4x Trinomial with one variable & degree 3.

2. y³+2y²+5

Solution: y³+2y²+5 Trinomial with one variable & degree 3.

Class 9 Math Solution WBBSE

Question 8. In the following algebraic expressions, which are polynomials in one variable, which are polynomials in two variables, and which are not polynomials – Let us write them.

1.  x² + 3x + 2

Solution: x² + 3x + 2, one variable

2. x² + y² + a²

Solution: x² + y² + a², one variable

3. y²– 4ax

Solution: y² – 4ax, one variable

4. x + y + 2

Solution: x + y +2, one variable

5. x⁸+y⁴+ x⁵y⁹

Solution: x⁸+y⁴+ x⁵y⁹, one variable

6. \(x+\frac{5}{x}\)

Solution: \(x+\frac{5}{x}=x+5 x^{-1}\) is not a polynomial as the degree of variable is not a whole number.

WBBSE Class 9 Maths Solutions Chapter 7 Polynomial Exercise 7.2

Question 1. If f(x) = x2+9x-6, then let us write by calculating the values of f(0), f(1) and f(3)

Solution: f(x) = x2+9x-6

\(\begin{aligned}
&f(0)=(0)^2+9.0-6=-6 \\
& f(1)=(1)^2+9.1-6=10-6=4 \\
& f(3)=(3)^2+9.3-6=9+27-6 \\
& =36-6=30 \\
&
\end{aligned}\)

Question 2. By calculating the following polynomials f(x), let us write the values of f(1) and f(-1).

1. f(x) = 2x³ + x² + x + 4

Solution: f(x) = 2x³ + x² + x + 4

\(\begin{aligned}
f(1) & =2(1)^3+(1)^2+1+4 \\
& =2+1+1+4=8 \\
f(-1) & =2(-1)^3+(-1)^2+(-1)+4 \\
& =-2+1-1+4 \\
& =5-3 \\
& =2
\end{aligned}\)

2. f(x) = 3x⁴– 5x³ + x² + 8

Solution: f(x) = 3x⁴– 5x³ + x² + 88

\(\begin{aligned}
f(1) & =3(1)^4-5(1)^3+(1)^2+8 \\
& =3-5+1+8 \\
& =7 \\
f(-1) & =3(-1)^4-5(-1)^3+(-1)^2+8 \\
& =3+5+1+8 \\
& =17
\end{aligned}\)

3. f(x) = 4 + 3x – x³ + 5x⁶

Solution: f(x) = 4 + 3x – x³ + 5x⁶

\(\begin{aligned}
f(1) & =4+3.1-(1)^3+5(1)^6 \\
& =4+3-1+5 \\
& =11 \\
f(-1) & =4+3(-1)-(-1)^3+5(-1)^6 \\
& =4-3+1+5 \\
& =7
\end{aligned}\)

4. f(x) = 6 + 10x – 7x²

Solution: f(x) = 6 + 10x – 7x²

\(\begin{aligned}
therefore f(1) & =6+10.1-7(1)^2 \\
& =6+10-7 \\
& =9 \\
f(-1) & =6+10(-1)-7(-1)^2 \\
& =6-10-7 \\
& =-11
\end{aligned}\)

Class 9 Maths WBBSE

Question 3. Let us check the following statements –

1. The zero of the polynomial P(x) = x – 1.

Solution: P(x)=x-1=0
∴ X = 1

2. The zero of the polynomial P(x) = 3-x is 3.

Solution: P(x)=3-x=0
∴ -x=-3 or, x = 3
∴ X=3,

3. The zero of the polynomial P(x) = 5x + 1 is

Solution: P(x)=5x+1=0
∴ 5x + 1 = 0 or, 5x = -1
∴ x = -1/5

4. The two zeroes of the polynomial P(x) = x² -9 are 3 and -3.

Solution: P(x) = x² -9=0
∴ x2-9=0 or, x2 = 9
or, x = ±3
∴ The two zeroes of the polynomial P(x) are 3 and -3.

5. The two zeroes of the polynomial P(x) = x²-5x are 0 and 5. Solve: P(x) = x²-5x=0

Solution: P(x) = x²-5x=0
∴ x² – 5x = 0 or, x(x-5)=0
or, x = 0 and, x-5=0; x = 5
∴ x = 0, 5
∴The two zeroes of the polynomial P(x) are 0 and (5).

6. The two zeroes of the polynomial P(x) = x²-2x-8 are 4 and (-2).

Solution: P(x) = x²-2x-8=0
∴ x²-2x-8=0
or, x²-4x+2x-8=0
or, x(x-4)+2(x-4)=0
or, (x-4) (x+2)=0
x = 0 and x + 2 = 0
∴ x = 4 and x = -2
∴ P(x) is a polynomial whose two zeroes are 4 and (-2).

Class 9 Maths WBBSE

Question 4. Let us determine the zeroes of the following polynomials –

1. f(x) = 2-x

Solution: f(x)=2-x … f(x) = 0
∴ 2-x=0
or, -x=-2
or, x = 2
∴ f(x) is a polynomial whose zero is 2.

2. f(x) = 7x + 2

Solution: f(x)=7x+2
∴ f(x) = 0
∴7x+2=0
or, 7x=-2
or, x = -2/7
∴ f(x) is a polynomial whose zero is -2/7

3. f(x) = x + 9

Solution: f(x)=x+9
∴f(x) = 0
∴x+9=0
or, x=-9
∴ f(x) is a polynomial whose zero is (-9).

4. f(x) = 62x

Solution: f(x) = 6-2x
∴ f(x) = 0
∴6-2x=0
or, -2x=-6
or, 2x = 6
or, x = 3
∴ f(x) is a polynomial whose zero is 3.

5. f(x) = 2x

Solution: f(x) = 2x
∴ f(x) = 0
∴2x = 0
or, x = 0
∴ f(x) is a polynomial whose zero is 0.

6. f(x) = ax + b, (a = 0)

Solution: f(x) = 0
∴ax + b = 0
∴ X = -a/b
∴ f(x) is a polynomial whose zero is (-b/a)

Class 9 Mathematics West Bengal Board Chapter 7 Polynomial Exercise 7.3

1. By applying Remainder Theorem, let us calculate and write the remainder that I shall get in each case, when x³-3x²+ 2x + 5 is divided by:

1. x-2

Solution: x-2
The zero of the linear polynomial x 2 = 0
∴ X = 2
From the Remainder Theorem, division of f(x) = x³-3x²+ 2x + 5 by (x-2) gives the remainder f(2).

∴ The required remainder = f(2) =(2)3-3 (2)² +2.2 +5 = 8-12+ 4+ 5 = 17-12 = 5

2. x+2

Solution: The zero of the linear polynomial x + 2 = 0
∴ X=-2
The required remainder = f(-2) =(-2)³-3(-2)² + 2 (-2)+5 =-8-12-4+5 =-19

3. 2x-1

Answer: The zero of the linear polynomial 2x-1=0 ∴x =1/2

\(\begin{aligned}
& =f\left(\frac{1}{2}\right) \\
& =\left(\frac{1}{2}\right)^3-3\left(\frac{1}{2}\right)^2+2 \cdot \frac{1}{2}+5 \\
& =\frac{1}{8}-\frac{3}{4}+1+5
\end{aligned}\)

 

\(\begin{aligned}
& =\frac{1}{8}-\frac{3}{4}+6 \\
& =\frac{1-6+48}{8} \\
& =\frac{43}{8} \\
& =5 \frac{3}{8}
\end{aligned}\)

 

4. 2x+1

Solution:

\(\begin{aligned}
& =f\left(-\frac{1}{2}\right) \\
& =\left(-\frac{1}{2}\right)^3-3\left(-\frac{1}{2}\right)^2+2\left(-\frac{1}{2}\right)+5 \\
& =-\frac{1}{8}-\frac{3}{4}-1+5 \\
& =\frac{-1-6-8+40}{8} \\
& =\frac{40-15}{8}=\frac{25}{8}=3 \frac{1}{8}
\end{aligned}\)

 

Question 2. By applying Remainder Theorem, let us calculate and write the remainders that I shall get when the following polynomials are divided by (x-1).

1. x³– 6x² + 13x + 60

Solution: Zero of the linear polynomial x -1 = 0
∴ X = 1
= f(1) = (1)³-6(1)² + 13.1 +60
= 1-6+13+60 =74-6=68

2. x³-3x² + 4x + 50

Solution: Let f(x) = x³-3x² + 4x + 50
= f(1) =(1)³-3(1)²+4.1 +50 = 1-3+4+ 50= 55 – 3 = 52

(3)4x³ + 4x²-x-1

Solution: Let f(x) = 4x³ + 4x²-x-1
= f(1)= 4(1)³-4(1)²-1-1= 4+4-1-1=8-2. = 6

(4)11x³– 12x² – x + 7

Solution: Let f(x) =11x³– 12x² – x + 7
= f(1) =11(1)³ – 12(1)²-1+7 11121+7 = 18 – 13= 5

Question 3. Applying Remainder Theorem, let us write the remainders, when –

1. The polynomial (x³-6x² + 9x-8) is divided by (x-3)

Solution: x-3=0
∴ X=3
(x) = x³-6x² + 9x-8

∴ Remainder = f(3) = (3)³-6(3)²+9(3)-8 =2754 +27-8 = 54-62=-8

2. The polynomial (x³ -ax² + 2x -a) is divided by (x-a).

Solution: x-a=0
∴ X = a
f(x) = x³ -ax² + 2x -a

∴ Remainder = f(a) = (a)³– a(a)² + 2.a – a = a³-a³+2a-a=a

Question 4. Applying Remainder Theorem, let us calculate whether the polynomial p(x) = 4x³+4x²-x -1 is a multiple of (2x + 1) or not.

Solution: 2x+1=0
∴ X=-1/2

∴ The zero of the linear polynomial (2x + 1) = -1/2
p(x) = 4x³+4x²-x -1
(2x+1) is a factor of P(x) if P(-1/2)=0

\(\begin{aligned}
& P\left(-\frac{1}{2}\right)=4\left(-\frac{1}{2}\right)^3+4\left(-\frac{1}{2}\right)^2-\left(-\frac{1}{2}\right)-1 \\
& =4\left(-\frac{1}{8}\right)+4\left(\frac{1}{4}\right)+\frac{1}{2}-1 \\
& =\frac{-1}{2}+1+\frac{1}{2}-1 \\
& =0
\end{aligned}\)

 

Question 5. For what value of a, the divisions of two polynomials (ax³+3x²-3) and (2x³-5x+a) by (x-4) give the same remainder – let us calculate and write it.

Solution: Let f(x) = ax³+3x²-3 and g(x) = 2x³– 5x + a.
If f(x) is divided by (x-4) the remainder is

\(\begin{aligned}
& f(4)=a(4)^3+3(4)^2-3 \\
& =64 a+48-3 \\
& =64 a+45
\end{aligned}\)

If g(x) is divided by'(x-4) the remainder is

\(\begin{aligned}
g(4) & =2(4)^3-5.4+a \\
& =128-20+a \\
& =108+a
\end{aligned}\)

∴ f(4) = g(4)
∴ 64a+45=108+a
or, 64a-a=108-45
or, 63a= 63
or, a = 1.
∴The value of a = 1

Question 6. The two polynomials x³ + 2x²-px-7 and x³ + px² – 12x + 6 are divided by (x + 1) and (x-2) respectively and if the remainder R₁ and R₂ are obtained such that 2R₁ + R₂=6, then let us calculate the value of p.

Solution: Let f(x) =x³ + 2x²-px-7
If f(x) is divided by (x + 1) the remainder is f(-1)=(-1)³ + 2(-1)²-P(-1)-7 =-1+2+P-7 =P-6
According to 1st condition, R₁ = P-6 …..(1)

Again, let g(x) = x³ + px² – 12x + 6
If g(x) is divided by (x-2) the remainder is g(2) = (2)³ + P(2)² – 12.2 +6 =8+4P-24 + 6 = 4P-10
According to 2nd condition, R₂ = 4P – 10 ………(2)

∴ 2R₁ + R₂ = 6
or, 2(P-6)+4P-10=6 or, 2P-12+ 4P-10=6
or, 6P 22=6
or, 6P=6+22
or, 6P=28

\(or, P=\frac{28}{6} or, P=\frac{14}{3}=4 \frac{2}{3}\)

∴The value of \(P=4 \frac{2}{3}\)

Question 7. The polynomial x⁴ – 2x³ + 3x²– ax + b is divided by (x-1) and (x + 1) and the remainders are 5 and 19 respectively. But if that polynomial is divided by x + 2, then what will be the remainder – Let us calculate.

Solution: Let f(x) = x⁴ – 2x³ + 3x²– ax + b
If f(x) is divided by (x-1) the remainder is f(1) =(1)⁴-2(1)³+3(1)-a.1+b = 1-2+3- a+b =2-a+b

According to 1st condition, 2-a+b=5
or,a+b=5-2
or, a – b = -3…(1)

Again, if f(x) is divided by (x + 1) the remainder is
f(-1)=(-1)⁴-2(-1)³+3(-1)²-a(-1)+b =1+2+3+a+b = a+b+6

According to 2nd condition, a+b+6=19
or, a+b=19-6
or, a+b=13 ……. (2)

from the eqation (2)/(1)
a+b=13/a – b = -3 = 2a =10

Adding, 2a = 10
or, a =10/2
or,a = 5

Putting value 4 in equation (2) we get, 5+b=13
b=13-5=8
∴ f(x) = x⁴ – 2x³ + 3x²– 5x + 8

If f(x) is divided by (x + 2) the remainder is
f(-2)=(-2)⁴ -2 (-2)³ + 3(-2)² -5(-2)+8 = =16+16 +12 + 10 + 8 = 62
The required remainder = 62.

Question 8. If \(f(x)=\frac{a(x-b)}{a-b}+\frac{b(x-a)}{b-a}\) then let us show that f(a) + f(b) = f(a + b).

Solution: \(f(x)=\frac{a(x-b)}{a-b}+\frac{b(x-a)}{b-a}\)

\(\begin{aligned}
& therefore f(a)=\frac{a(a-b)}{a-b}+\frac{b(a-a)}{b-a} \\
& =a+0 \\
& =a \\
& f(b)=\frac{a(b-b)}{a-b}+\frac{b(b-a)}{b-a} \\
& =0+b \\
& =b \\
&∴f(a)+f(b)=a+b \\
&
\end{aligned}\)

 

Again, \(\begin{aligned}
f(a+b) & =\frac{a(a+b-b)}{a-b}+\frac{b(a+b-a)}{b-a} \\
& =\frac{a^2}{a-b}+\frac{b^2}{-(a-b)} \\
& =\frac{a^2}{a-b}-\frac{b^2}{a-b} \\
& =\frac{a^2-b^2}{a-b} \\
& =\frac{(a+b)(a-b)}{(a-b)} \\
& =a+b
\end{aligned}\)

Question 9. If f(x) = ax + b and f(0) = 3, f(2) = 5, then let us determine the values of a and b.

Solution: f(x) = ax + b
∴f(0) = a.0+ b = 0 + b = b
∴b = 3
Again, f(2) = a.2 + b = 2a + b
∴2a + b = 5
or, 2a+35 [∴ b = 3]
or, 2a=5-3
or, 2a = 2
or, a = 1
∴ a = 1 and b = 3

Question 10. If f(x) = ax² + bx + c and f(0) = 2, f(1) = 1, and f(4) = 6, then let us calculate the values of a, b, and c.

Solution: f(x) = ax² + bx + c
∴ f(0) = a(0)²+ b.0+ c =0+0+c=c
∴ c = 2 ….(1)

Again, f(1)= a(1)²+b.1+ c= a+b+c
∴ a+b+c=1
or, a+b+2=1(∴c=2)
or, a+b=1-2
or, a+b=-1 ……(2)

Again, f(4) = a(4)2 + b.4 + c = 16a+ 4b + C
16a+ 4b+c=6
or, 16a+ 4b+2=6(c=2)
or, 16a+ 4b6-2
or, 16a+ 4b4
or, 4(4a + b) = 4

or, \(4 a+b=\frac{4}{4}\)

4a + b = 1 …..(3)

Divide equation (3)/(2)

\(\begin{aligned}
& 4 a+b=1 \\
& a+b=-1 \\
& (-) \quad(-) \quad(+) \\
& 3 a \quad=2
\end{aligned}\)

or, a =2/3

Putting the value of a in equation (2),

\(\frac{2}{3}+b=-1\)

or, \(b=-1-\frac{2}{3}\)

or,\(b=-\left(\frac{3+2}{3}\right)\)

or,\(b=\frac{-5}{3}\)

∴\(a=\frac{2}{3}, b=\frac{-5}{3}, c=2\)

Question 11. Multiple Choice Questions

1. Which of the following is a polynomial in one variable?

1. \(x+\frac{2}{x}+3\)

2. \(3 \sqrt{x}+\frac{2}{\sqrt{x}}+5\)

3. \(\sqrt{2} x^2-\sqrt{3} x+6\)

4. \(x^{10}+y^5+8\)

Solution: 3. \(\sqrt{2} x^2-\sqrt{3} x+6\)

2. Which of the following is a polynomial?

1. x-1

2. \(\frac{x-1}{x+1}\)

3. \(x^2-\frac{2}{x^2}+5\)

4. \(x^2+\frac{2 x^{\frac{3}{2}}}{\sqrt{x^2}}+6\)

Solution: 1. x-1

3. Which of the following is a linear polynomial?

1. x + x²
2. x + 1
3. 5x²-x+3
4. \(x+\frac{1}{x}\)

Solution:2. x + 1

4. Which of the following is a second-degree polynomial?

1. √x-4
2. x³ + x
3. x³+ 2x + 6
4. x²+ 5x + 6

Solution: 4. x²+ 5x + 6

5. The degree of the polynomial √3 is

1. 1/2
2.  2
3. 1
4. 0

Solution: 4. 0

Question 12. Short answer type questions :

1. Let us write the zero of the polynomial p(x) = 2x – 3.

Solution: 2x-3=0
∴ X =3/2
∴The zero of polynomial p(x) = 2x – 3 is 3/2.

2. If p(x) = x + 4, let us write the value of p(x) + P(-x).

Solution: p(x) = x+4
∴ p(x) + p(x) = x+4x+4=8.

3. Let us write the remainder, if the polynomial x³ + 4x² + 4x – 3 is divided by x.

Solution: Let f(x) =x³ + 4x² + 4x – 3
∴ The required remainder =f(0) = (0)³ + 4(0)²+4.0-3=-3.

4. If \((3 x-1)^7=a_7 x^7+a_6 x^6+a_5 x^5+\ldots \ldots \ldots+a_1 x+a_0\) then let us write the value of \(a_7+a_6+a_5+\ldots \ldots \ldots+a_0\) (where a₇, a₆……….a₀ are constants).

Solution: \((3 x-1)^7=a_7 x^7+a_6 x^6+a_5 x^5+\ldots \ldots \ldots+a_1 x+a_0\)

or, (3.1-1)⁷ = a₇(1)7+ a₆(1)⁶ + a₅(1)⁵+. . ..+a₁x+a₀ (Putting x = 1 on both sides)
∴ (2)⁷ = a₇+ a₆+ a₅… a₁+ a₀
∴ a₇+ a₆ + a₅ + ……. a₁+ a₀ = 128.

 

Chapter 7 Polynomial Exercise 7.4

 

Question 1. Let us calculate and write, which of the following polynomials will have a factor (x+1).

Solution: x + 10 ∴x=-1
∴ The zero of polynomial (x + 1) is – 1.

1. 2x³ + 3x² – 1

Solution: Let f(x) =2x³ + 3x² – 1
∴f(-1) =2(-1)³+3(-1)²-1 =-2+3-1=3-3 = 0
∴ The factor of 2x³ + 3x² – 1 is (x + 1).

2. x⁴ + x³– x² + 4x + 5

Solution: Let f(x) = x⁴ + x³– x² + 4x + 5
∴ f(-1)=(-1)⁴+(-1)³-(-1)² + 4(-1)+5 1-1-1-4+5 =6-6 = 0
∴ One factor of x⁴ + x³– x² + 4x + 5 is x + 1.

3. 7x³ + x² + 7x + 1

Solution: Let f(x) = 7x³ + x² + 7x + 1 =7(-1)³+(-1)²+7(-1)+1 =-7+1-7+1 = 2 – 14 = 12
∴ (x + 1) is a factor of (7x³ + x² + 7x + 1).

4. 3+3x-5x³– 5x⁴

Solution: Let f(x)=3+3x-5x³– 5x⁴
f(-1)= 3+3(-1)-5(-1)³-5(-1)⁴ = 3-3+5-5 = 8-8 = 0
∴ One factor of 3+3x-5x³– 5x⁴ is (x + 1).

5. x⁴ + x² + x +1

Solution: Let f(x) =x⁴ + x² + x +1
f(-1) = (-1)⁴+(-1)²+(-1)+1 =1+1 1+1 = 3-1 = 2
∴ (x + 1) is a factor of (x⁴ + x² + x +1).

6. x³ + X² + X +1

Solution: f(-1) = (-1)³+(-1)²+(-1)+1=-1+1+1+1=2-2 = 0
∴ One factor of x³ + X² + X +1 is (x + 1).

Question 2. By using Factor Theorem, let us write whether g(x) is a factor of the following polynomials f(x):

1. f(x) = x⁴-x²-12 and g(x) = x + 2

Solution: g(x) = x + 2 is a factor of f(x) if f(-2) = 0
∴ f(x)= x⁴-x²-12 = (-2)⁴-(-2)²-12=16- 4- 12 = 16- 16 = 0
∴ g(x) is a factor of f(x).

2. f(x) = 2x³ +9x²-11x-30 and g(x) = x + 5

Solution: x+5=0
∴ X=-5
∴ f(x) = 2x³ +9x²-11x-30
f(-5) = 2(-5)³ +9(-5)²-11x-30=250+225 +55 – 30 = 280 280= 0
∴ g(x) is a factor of f(x).

3. f(x) = 2x³ + 7x²-24x-45 and g(x) = x – 3

Solution: x-3=0
∴ X=3
∴ f(x) = 2x³ + 7x²-24x-45
∴ f(3) = 2(3)³+7(3)²-24.3-45=54+63 72-45= 117 117 = 0
∴ g(x) is a factor of f(x).

4. f(x) = 3x³ + x² – 20x + 12 and g(x) = 3x – 2

Solution:3x-2=0
∴ f(x) = 3x³ + x² – 20x + 12

\(\begin{aligned}
f\left(\frac{2}{3}\right) & =3\left(\frac{2}{3}\right)^3+\left(\frac{2}{3}\right)^2-20 \cdot \frac{2}{3}+12 \\
& =3 \cdot \frac{8}{27}+\frac{4}{9}-\frac{40}{3}+12 \\
& =\frac{8}{9}+\frac{4}{9}-\frac{40}{3}+12 \\
& =\frac{8+4-120+108}{9} \\
& =\frac{120-120}{9}=\frac{0}{9}=0
\end{aligned}\)

∴ g(x) is a factor of f(x).

Question 3. Let us calculate and write the value of k for which the polynomial 2x + 3×3+2kx2 + 3x + 6 is divided by x + 2.
Solution: Let f(x) = 2x + 3x³+2kx² + 3x + 6
The zero of (x + 2) is – 2.
∴ (x+2) is a factor of f(x).
∴ f(-2)=0
∴ 2(-2)⁴+3(-2)³+2.k.(-2)²+3(-2)+6=0
or, 32-24+8k-6+6=0
or, 8+ 8k = 0
or, 8k=-8
or, k=-1

Question 4. Let us calculate the value of k for which g(x) will be a factor of the following polynomials f(x):

1.  f(x) = 2x³ +9x²+ x + k and g(x) = x-1

Solution: g(x) = (x-1) is a factor of f(x) if x = 1 (x-1=0. x = 1)
∴ g(x) is a factor of f(x)
∴ f(1) = 0
∴ 2(1)³+9(1)²+1+k=0
or, 2+9+1+k=0
or, k=-12
∴ If k = 12, g(x) is a factor of f(x).

2. f(x)=kx²-3x+k and g(x)=x-1

Solution: g(x) = (x-1) is a factor of f(x) if x = 1 (x-1=0. x = 1)
∴g(x) is a factor of f(x)
∴f(1) = 0
∴ k(1)²-3.1+k=0
or, 2k-3=0
or, k = 3/2
∴ If k = 3/2,g(x) is a factor of f(x).

3. f(x) =2x⁴ + x³ – kx²-x+6 and g(x) = 2x-3

Solution: g(x) = 2x-3.
∴ The zero of g(x) is 3/2(2x-3=0 ∴X =3/2)
∴ g(x) is a factor of f(x)

\(\begin{aligned}
& f\left(\frac{3}{2}\right)=0 \\
&2\left(\frac{3}{2}\right)^4+\left(\frac{3}{2}\right)^3-k\left(\frac{3}{2}\right)^2-\frac{3}{2}+6=0 \\
& \text { or, } 2 \cdot \frac{81}{16}+\frac{27}{8}-\frac{9 k}{4}-\frac{3}{2}+6=0 \\
& \text { or, } \frac{81}{8}+\frac{27}{8}-\frac{9 k}{4}-\frac{3}{2}+\frac{6}{1}=0 \\
& \text { or, } \frac{81+27-18 k-12+48}{8}=0 \\
& \text { or, } 144-18 k=0 \\
& \text { or, }-18 k=-144 \\
& \text { or, } k=\frac{-144}{-18} \\
& k=8
\end{aligned}\)

∴If k= 8 then g(x) will be a footer of W

4. f(x) =2x³ + kx² + 11x+ k + 3 and g(x) = 2x – 1

Solution: The zero of polynomial g(x) = (2x-1) is 1/2(2x-1=0 ∴x = 1/2)
∴ g(x) is the factor of f(x)

\(\begin{aligned}
& f\left(\frac{1}{2}\right)=0 \\
& 2\left(\frac{1}{2}\right)^3+K\left(\frac{1}{2}\right)^2+11 \cdot \frac{1}{2}+K+3=0
\end{aligned}\)

 

\(\text { 2. } \frac{1}{8}+k \cdot \frac{1}{4}+\frac{11}{2}+k+3=0\)

 

\(\frac{1}{4}+\frac{k}{4}+\frac{11}{2}+k+3=0\)

 

\(\frac{1+k+22+4 k+12}{4}=0\)

 

or, 5k + 35 = 0
or, 5k = -35
or, k = -35/5 = -7
∴ If k=-7, g(x) is a factor of f(x).

Question 5. Let us calculate and write the values of a and b if x²-4 is a factor of the polynomial ax⁴ + 2x³-3x²+ bx-4.

Solution: x²-4=0
or, (x+2) (x-2)=0
x+2=0 or, x-2=0
Let f(x) = ax⁴ + 2x³-3x²+ bx-4

∴ (x²-4) is a factor of f(x)
∴ f(2) = 0 and f(-2) = 0
f(2) = 0
∴ a(2)⁴+2(2)³-3(2)²+ b.2-4=0
or, 16a+ 16-12+2b-4=0
or, 16a+2b=0
or, 8a+ b = 0 …..(1)

f(-2)=0
∴a(-2)⁴+2(-2)³-3(-2)²+ b.(-2)-4=0
or, 16a-16-12-2b-4=0
or, 16a-2b= 32
or,8a-b=16 ….(2)

8a+ b = 0

Divide by the equation (2)/(1)

\(\begin{aligned}
& 8 a-b=16 \\
& 8 a+b=0
\end{aligned}\)

 

or, a =16/16
or, a =1

Putting the value of a in equation (1),
8.1+b=0
or, b=-8
∴ a = 1, b=-8

Question 6. If (x + 1) and (x+2) are two factors of the polynomial x³ + 3x²+2ax + b, then let us calculate and write the values of a and b.

Solution: Let f(x) = x³ + 3x²+2ax + b
The zero of (x + 1) is -1 (x+1=0  ∴x=-1)
∵ (x + 1) is a factor of f(x)
∴ f(-1)-0
∴ (-1)³+3(-1)²+2a(-1)+b=0
or, -1+3-2a+b=0
or, 2-2a+b=0
or, 2a+b=-2
or, 2a-b=2 ….(1)

Again, the zero of (x + 2) is -2
∵ (x+2) is a factor of f(x)
∴ f(-2)=0
∴ (-2)³+3(-2)²+2a(-2)+b=0
or, -8+12-4a+b=0
or, 4-4a+b=0
or, 4a+b=-4
or, 4a-b = 4 …(2)

(2)-(1)

\(\begin{aligned}
& 4 a-b=4 \\
& 2 a-b=2 \\
& (-)(+)(-) \\
& 2 a=2
\end{aligned}\)

Subtracting, 2a=2
or, a = 1

Putting the value of in equation (1), 2.1-b=2
or, -b = 2-2
or, – b = 0
or, b = 0
∴ a = 1, b = 0.

Question 7. If the polynomial ax³ + bx²+x-6 is divided by x-2 and the remainder is 4, then et us calculate the values of a and b when x + 2 is a factor of this polynomial.

Solution: Let f(x) = ax³ + bx²+x-6
∴ If f(x) is divided by (x-2), the remainder is 4
∴f(2) = 4[ The zero of (x-2) is 2]
or, 8a+ 4b-4 = 4
or, 4(2a + b) = 4+4
or, (2a + b) = 8/4
or, 2a + b = 2

Again, (x+2) is a factor of f(x)
∴ f(-2) = 0 (∵x+2=0 ∴x=-2)
∴ a(-2)³+(-2)2+(-2)-6-0
or, -8a+ 4b-2-6=0
or, -8a+ 4b = 8
or, -4(2a – b) = 8

or, \(2 a-b=\frac{8}{-4}\)

or, 24-b =-2 ….(2)

Adding, (2)+(1)

\(\begin{aligned}
& 2 a-b=-2 \\
& 2 a+b=2 \\
& 4 a=0
\end{aligned}\)

or, a = 0
∴ 2.0 + b = 2
or, 0+b=2
or, b = 2
∴ a = 0, b = 2

Question 8. Let us show that if n is any positive integer (even or odd), x-y is a factor of the polynomial xⁿ – yⁿ

Solution: x – y = 0
∴ x = y
Let f(x) = xⁿ – yⁿ
∴f(y) = yⁿ – yⁿ= 0
(x-y) is a factor of xⁿ – yⁿ.

Question 9. Let us show that if n is any positive odd integer, then x + y is a factor of xⁿ + yⁿ.

Solution: x + y = 0
∴ x = – Y
Let f(x) = xⁿ + yⁿ
∴ f(-y)= (-y)ⁿ+ yⁿ =-yⁿ+ yⁿ= 0
∴ (x + y) is a factor of xⁿ + yⁿ.

Question 10. Let us show that if n be any positive integer (even or odd), then x – y will never be a factor of the polynomial xⁿ + yⁿ

Solution: x-y=0
∴ x = y
Let f(x) = xⁿ + yⁿ
f(y) = (y)ⁿ+ yⁿ
f(y) = 2yⁿ ≠0
∴ (x-y) can never be a factor of f(x) = (xⁿ + yⁿ).

Question 11. Multiple Choice Questions

1. x³ + 6x² + 4x + k (x + 2)

(1)-6
(2)-7
(3)-8
(4) 10

Solution: Let f(x) = x³ + 6x² + 4x + k
The zero of (x+2) is -2
∴ (x+2) is a factor of f(x)
∴ f(-2) = 0
∴ (-2)³+6(-2)²+4(-2)+k=0
or, 8+24-8+k=0
or, 8+ k = 0.
or, k=-8

∴(3) – 8

2. In the polynomial f(x) if \(f\left(-\frac{1}{2}\right)=0\)  then a factor of f(x) will be

(1) 2x-1
(2) 2x + 1
(3) x-1
(4) x + 1

Solution: \(f\left(-\frac{1}{2}\right)=0\)

∴X =-1/2
or, 2x = -1
or, 2x+1=0
∴(2x+1) is a factor of f(x).

∴ (2)2x + 1

3. (x-1) is a factor of the polynomial f(x) but it is not a factor of g(x). So (x-1) will be a factor of

(1)f(x) g(x)
(2)- f(x) + g(x)
(3)f(x) = g(x)
(4){f(x) + g(x)}g(x)

Solution: (x-1) is a factor of f(x) g(x)
∴ (1)f(x) g(x)

4. (x + 1) is a factor of the polynomial xⁿ + 1 when

(1) n is a positive odd integer
(2) n is a positive even integer
(3) n is a negative integer
(4) n is a positive integer

Solution: We know if n is an odd positive integer, (x+y) is a factor of (xⁿ + yⁿ )
∴ (x + 1) is a factor of xⁿ+ 1, i.e., xⁿ+1ⁿ when n is an odd positive integer.

∴(1) n is a positive odd integer

5. If n²-1 is a factor of the polynomial an⁴ + bn³ + cn² + dn + e

(1) a +c+e=b+d
(2) a+b+e=c+d
(3) a+b+c=d+e
(4) b+c+d=a+e

Solution: Let f(n) = an⁴ + bn³ + cn² + dn + e
Factor of 1(n) = n²-1-(n + 1) (n-1)
∴ f(-1)=0 and f(1) = 0 When f(-1) = 0.

or, a(-1)⁴+b(-1)³+c(-1)² +d(-1)+e=0
or, a-b+c-d+e=0
∴ a+c+e=b+d

Again, f(1)=0
∴ a(1)⁴+b(1)³+c(1)²+d.1+e=0
or, a+b+c+d+e=0
∴ a+c+e-b-d

∴(1) a +c+e=b+d

Question 12. Short answer type questions:

1. Let us calculate and write the value of a for which x + a will be a factor of the polynomial x³+ax²-2x+a-12.

Solution: Let f(x) = x³+ax²-2x+a-12
Factor of f(x) is (x + a)
∴f(-a) = 0
∴(-a)³ + a(-a)²-2(-a) + a-12=0
or,(-a)³+ a³+2a + a-12=0
or, 3a= 12
or, a = 12/3
or, a = 4

2. Let us calculate and write the value of k for which x-3 will be a factor of the polynomial k²x³-kx²+3kx-k.

Solution: Let f(x) = k²x³-kx²+3kx-k
Factor of f(x) is (x-3)
∴f(3) = 0
∴k²(3)³-k(3)²+3k.3-k=0
or, 27k²-9k+9k – k=0
or, 27k²-k=0
or, k(27k-1)=0 = k = 0 and 27k10.
∴ k = 1/27
∴ k = 0, 1/27

3. Let us write the value of f(x) + f(-x) when f(x) = 2x + 5.

Solution: f(x) = 2x + 5
∴ f(x) + f(x) = 2x+5+2(-x)+5 =2x+5-2x+5=10

4. Both (x-2) and \(\left(x-\frac{1}{2}\right)\) are factors of the polynomial px² + 5x + r, let us calculate and write the relation between p and r.

Solution: Let f(x) = px² + 5x + r
Factor of f(x) is (x-2)
∴ f(2) = 0 (x-2=0; x=2) .
∴ p(2)²+ 5.2 + r=0
or, 4p+r+10=0
or, 4p+r=-10  ….(1)

Again, factor of f(x) is (x) is \(\left(x-\frac{1}{2}\right)\)

\(\begin{aligned}
&  f\left(\frac{1}{2}\right)=0\left(because x-\frac{1}{2}=0 therefore x=\frac{1}{2}\right) \\
& p\left(\frac{1}{2}\right)^2+5 \cdot \frac{1}{2}+r=0 \\
& \text { or, } \frac{p}{4}+\frac{5}{2}+r=0 \\
& \text { or, } \frac{p+10+4 r}{4}=0
\end{aligned}\)

or, p +4r + 10 = 0

or, p + 4r = -10 .,…..(2)

(1) and (2)

4p + r = p + 4r
or, 4p – p = 4r -r
or, 3p = 3r
or, p = r

5. Let us write the roots of the linear polynomial f(x) = 2x + 3. Solve: 2x+3=0

Solution: 2x=-3
or, 2x = -3
or, x = -3/2
∴ The roots of f(x) = 2x + 3 = -3/2

 

Class IX Maths Solutions WBBSE Chapter 8 Factorisation: Formulae of Factorisation

1. a² – b² = (a + b) (a – b)
2. a³+b³ = (a + b) (a² – ab + b²)
3. a³-b³ (a – b) (a² + ab + b²)
4. a³ + b + c³-3abc = (a+b+c) (a²+ b²+c²-ab-bc-ca)

Read and Learn More WBBSE Solutions For Class 9 Maths

Ganit Prakash Class 9 Solutions Chapter 8 Factorisation Exercise 8.1

 

Let us factorise the following polynomials

Question 1. x³-3x+2

Solution: x³-3x+2

\(\begin{aligned}
& =x^3-1-3 x+3 \\
& =(x)^3-(1)^3-3(x-1) \\
& =(x-1)\left(x^2+x+1\right)-3(x-1) \\
& =(x-1)\left(x^2+x+1-3\right) \\
& =(x-1)\left(x^2+x-2\right) \\
& =(x-1)\left(x^2+2 x-x-2\right) \\
& =(x-1)\{x(x+2)-1(x+2)\} \\
& =(x-1)(x+2)(x-1) \\
& =(x-1)^2(x+2)
\end{aligned}\)

 

Question 2. x³ + 2x + 3

Solution: x³ + 2x + 3

\(\begin{aligned}
& =x^3+1+2 x+2 \\
& =(x)^3+(1)^3+2(x+1) \\
& =(x+1)\left(x^2-x+1\right)+2(x+1) \\
& =(x+1)\left(x^2-x+1+2\right) \\
& =(x+1)\left(x^2-x+3\right)
\end{aligned}\)

Ganit Prakash Class 9 Solutions

Question 3.a³-12a-16

Solution: a³-12a-16

\(\begin{aligned}
& =a^3+8-12 a-24 \\
& =(a)^3+(2)^3-12(a+2) \\
& =(a+2)\left(a^2-2 a+4\right)-12(a+2) \\
& =(a+2)\left(a^2-2 a+4-12\right) \\
& =(a+2)\left(a^2-2 a-8\right) \\
& =(a+2)\left(a^2-4 a+2 a-8\right) \\
& =(a+2)\{a(a-4)+2(a-4)\} \\
& =(a+2)(a+2)(a-4) \\
& =(a+2)^2(a-4)
\end{aligned}\)

 

Question 4. x³– 6x + 4

Solution: x³– 6x + 4

\(\begin{aligned}
& =x^3-8-6 x+12 \\
& =(x)^3-(2)^3-6(x-2) \\
& =(x-2)\left(x^2+2 x+4\right)-6(x-2) \\
& =(x-2)\left(x^2+2 x+4-6\right) \\
& =(x-2)\left(x^2+2 x-2\right)
\end{aligned}\)

Ganit Prakash Class 9 Solutions

Question 5. x³-19x-30

Solution: x³-19x-30

\(\begin{aligned}
& =x^3+8-19 x-38 \\
& =(x)^3+(2)^3-19(x+2) \\
& =(x+2)\left(x^2-2 x+4\right)-19(x+2) \\
& =(x+2)\left(x^2-2 x+4-19\right) \\
& =(x+2)\left(x^2-2 x-15\right) \\
& =(x+2)\left(x^2-5 x+3 x-15\right) \\
& =(x+2)\{x(x-5)+3(x-5)\} \\
& =(x+2)(x+3)(x-5)
\end{aligned}\)

 

Question 6. 4a³-9a²+ 3a +2

Solution: 4a³-9a²+ 3a +2
If a =1, 4a³-9a²+ 3a +2=0
∴ (a-1) is a factor of 4a³-9a²+ 3a +2

\(\begin{aligned}
& =4 a^3-9 a^2+3 a+2 \\
& =4 a^3-4 a^2-5 a^2+5 a-2 a+2 \\
& =4 a^2(a-1)-5 a(a-1)-2(a-1) \\
& =(a-1)\left(4 a^2-5 a-2\right)
\end{aligned}\)

Ganit Prakash Class 9 Solutions

Question 7. x³-9x²+23x-15

Solution: If x = 1, x³-9x²+23x-15=0
∴ (x-1) is a factor of  x³-9x²+23x-15

\(\begin{aligned}
& =x^3-x^2-8 x^2+8 x+15 x-15 \\
& =x^2(x-1)-8 x(x-1)+15(x-1) \\
& =(x-1)\left(x^2-8 x+15\right) \\
& =(x-1)\left(x^2-5 x-3 x+15\right) \\
& =(x-1)\{x(x-5)-3(x-5)\} \\
& =(x-1)(x-3)(x-5)
\end{aligned}\)

 

Question 8. 5a³ +11a²+ 4a-2

Solution: If a=-1,5a³ +11a²+ 4a-2=0
∴ (a + 1) is a factor of 5a³ +11a²+ 4a-2

\(\begin{aligned}
& =5 a^3+5 a^2+6 a^2+6 a-2 a-2 \\
& =5 a^2(a+1)+6 a(a+1)-2(a+1) \\
& =(a+1)\left(5 a^2+6 a-2\right)
\end{aligned}\)

Class 9 Math Solution WBBSE In English

Question 9. 2x³– x² + 9x + 5

Solution: If x = -1/2, 2x³– x² + 9x + 5=0
∴(2x + 1) is a factor of 2x³– x² + 9x + 5

\(\begin{aligned}
& =2 x^3+x^2-2 x^2-x+10 x+5 \\
& =x^2(2 x+1)-x(2 x+1)+5(2 x+1) \\
& =(2 x+1)\left(x^2-x+5\right)
\end{aligned}\)

 

Question 10. 2y³-5y²-19y+ 42

Solution: Putting, y = 2 then 2y³-5y²-19y+ 42= 0
∴ (y-2) is a factor of 2y³-5y²-19y+ 42

\(\begin{aligned}
& =2 y^3-4 y^2-y^2+2 y-21 y+42 \\
& =2 y^2(y-2)-y(y-2)-21(y-2) \\
& =(y-2)\left(2 y^2-y-21\right) \\
& =(y-2)\left(2 y^2-7 y+6 y-21\right) \\
& =(y-2)\{y(2 y-7)+3(2 y-7)\} \\
& =(y-2)(y+3)(2 y-7)
\end{aligned}\)

Class 9 Math Solution WBBSE In English Chapter 8 Factorisation Exercise 8.2

Question 1. \(\frac{x^4}{16}-\frac{y^4}{81}\)

Solution: \(\frac{x^4}{16}-\frac{y^4}{81}\)

\(\begin{aligned}
& =\left(\frac{x^2}{4}\right)^2-\left(\frac{y^2}{9}\right)^2 \\
& =\left(\frac{x^2}{4}+\frac{y^2}{9}\right)\left(\frac{x^2}{4}-\frac{y^2}{9}\right) \\
& =\left(\frac{x^2}{4}+\frac{y^2}{9}\right)\left\{\left(\frac{x}{2}\right)^2-\left(\frac{y}{3}\right)^2\right\} \\
& =\left(\frac{x^2}{4}+\frac{y^2}{9}\right)\left(\frac{x}{2}+\frac{y}{3}\right)\left(\frac{x}{2}-\frac{y}{3}\right)
\end{aligned}\)

 

Question 2. \(m^2+\frac{1}{m^2}+2-2 m-\frac{2}{m}\)

Solution: \(\begin{aligned}
& \left(m^2+\frac{1}{m^2}+2-2 m-\frac{2}{m}\right. \\
& (m)^2+\left(\frac{1}{m}\right)^2+2-2\left(m+\frac{1}{m}\right)
\end{aligned}\)

\(\begin{aligned}
& =\left(m+\frac{1}{m}\right)^2-2 m \cdot \frac{1}{m}+2-2\left(m+\frac{1}{m}\right) \\
& =\left(m+\frac{1}{m}\right)^2-2+2-2\left(m+\frac{1}{m}\right) \\
& =\left(m+\frac{1}{m}\right)^2-2\left(m+\frac{1}{m}\right) \\
& =\left(m+\frac{1}{m}\right)\left(m+\frac{1}{m}-2\right)
\end{aligned}\)

 

Question 3. \(9 p^2-24 p q+16 q^2+3 a p-4 a q\)

Solution: \(9 p^2-24 p q+16 q^2+3 a p-4 a q\)

\(\begin{aligned}
& =(3 p)^2-2.3 p .4 q+(4 q)^2+a(3 p-4 q) \\
& =(3 p-4 q)^2+a(3 p-4 q) \\
& =(3 p-4 q)(3 p-4 q+a)
\end{aligned}\)

Class 9 Maths WBBSE

Question 4. 4x⁴+81

Solution: 4x⁴+81

\(\begin{aligned}
& =\left(2 x^2\right)^2+(9)^2 \\
& =\left(2 x^2+9\right)^2-2.2 x^2 .9 \\
& =\left(2 x^2+9\right)^2-36 x^2 \\
& =\left(2 x^2+9\right)^2-(6 x)^2 \\
& =\left(2 x^2+9+6 x\right)\left(2 x^2+9-6 x\right) \\
& =\left(2 x^2+6 x+9\right)\left(2 x^2-6 x+9\right)
\end{aligned}\)

 

Question 5. x⁴ – 7x² + 1

Solution: x⁴ – 7x² + 1

\(\begin{aligned}
& =\left(x^2\right)^2+(1)^2-7 x^2 \\
& =\left(x^2+1\right)^2-2 \cdot x^2 \cdot 1-7 x^2 \\
& =\left(x^2+1\right)^2-9 x^2 \\
& =\left(x^2+1\right)^2-(3 x)^2 \\
& =\left(x^2+1+3 x\right)\left(x^2+1-3 x\right) \\
& =\left(x^2+3 x+1\right)\left(x^2-3 x+1\right)
\end{aligned}\)

 

Question 6. p⁴-11p²q²+q⁴

Solution: p⁴-11p²q²+q⁴

\(\begin{aligned}
& =\left(p^2\right)^2+\left(q^2\right)^2-11 p^2 q^2 \\
& =\left(p^2-q^2\right)^2+2 \cdot p^2 \cdot q^2-11 p^2 q^2 \\
& =\left(p^2-q^2\right)^2-9 p^2 q^2 \\
& =\left(p^2-q^2\right)^2-(3 p q)^2 \\
& =\left(p^2-q^2+3 p q\right)\left(p^2-q^2-3 p q\right) \\
& =\left(p^2+3 p q-q^2\right)\left(p^2-3 p q-q^2\right)
\end{aligned}\)

Class 9 Maths WBBSE

Question 7. a² + b² -c² -2ab

Solution: a² + b² -c² -2ab

\(\begin{aligned}
& =a^2-2 a b+b^2-c^2 \\
& =(a-b)^2-(c)^2 \\
& =(a-b+c)(a-b-c)
\end{aligned}\)

 

Question 8. 3a(3a+2c) – 4b(b + c)

Solution: 3a(3a+2c) – 4b(b + c) = 9a2+6ac4b2-4bc

\(\begin{aligned}
& =9 a^2+6 a c-4 b^2-4 b c \\
& =9 a^2-4 b^2+6 a c-4 b c \\
& =(3 a)^2-(2 b)^2+2 c(3 a-2 b) \\
& =(3 a+2 b)(3 a-2 b)+2 c(3 a-3 b) \\
& =(3 a-2 b)(3 a+2 b+2 c)
\end{aligned}\)

 

Question 9. a²-6ab + 12bc-4c²

Solution: a²-6ab + 12bc-4c²

\(\begin{aligned}
& =(a)^2-(2 c)^2-6 a b+12 b c \\
& =(a+2 c)(a-2 c)-6 b(a-2 c) \\
& =(a-2 c)(a+2 c-6 b) \\
& =(a-2 c)(a-6 b+2 c)
\end{aligned}\)

Class 9 Maths WBBSE

Question 10. 3a²+4ab+b²-2ac- c²

Solution: 3a²+4ab+b²-2ac- c²

\(\begin{aligned}
& =4 a^2+4 a b+b^2-a^2-2 a c-c^2 \\
& =(2 a)^2+2 \cdot 2 a \cdot b+(b)^2-\left(a^2+2 a c+c^2\right) \\
& =(2 a+b)^2-(a+c)^2 \\
& =(2 a+b+a+c)(2 a+b-a-c) \\
& =(3 a+b+c)(a+b-c)
\end{aligned}\)

Class 9 Maths WBBSE

Question 11. x² -y²-6ax + 2ay + 8a²

Solution: x² -y²-6ax + 2ay + 8a²

\(\begin{aligned}
& =x^2-6 a x+9 a^2-a^2+2 a y-y^2 \\
& =(x)^2-2 \cdot x \cdot 3 a+(3 a)^2-\left(a^2-2 a y+y^2\right) \\
& =(x-3 a)^2-(a-y)^2 \\
& =(x-3 a+a-y)(x-3 a-a+y) \\
& =(x-2 a-y)(x-4 a+y)
\end{aligned}\)

 

Question 12. a²-9b²+4c²-25d²-4ac+30bd

Solution: a²-9b²+4c²-25d²-4ac+30bd

\(\begin{aligned}
& =a^2-4 a c+4 c^2-9 b^2+30 b d-25 d^2 \\
& =(a)^2-2 \cdot a \cdot 2 c+(2 c)^2-\left(9 b^2-30 b d+25 d^2\right) \\
& =(a-2 c)^2-\left\{(3 b)^2-2 \cdot 3 b \cdot 5 d+(5 d)^2\right\} \\
& =(a-2 c)^2-(3 b-5 d)^2 \\
& =(a-2 c+3 b-5 d)(a-2 c-3 b+5 d) \\
& =(a+3 b-2 c-5 d)(a-3 b-2 c+5 d)
\end{aligned}\)

WBBSE Class 9 Maths Solutions

Question 13. 3a²b²-c²+2ab-2bc + 2ca

Solution: 3a²b²-c²+2ab-2bc + 2ca

\(\begin{aligned}
& =3 a^2-a b-a c+3 a b-b^2-b c+3 a c-b c-c^2 \\
& =a(3 a-b-c)+b(3 a-b-c)+c(3 a-b-c) \\
& =(3 a-b-c)(a+b+c)
\end{aligned}\)

 

Question 14. x² -2x-22499

Solution: x²-2x-22499

\(\begin{aligned}
& =x^2-(151-149) x-22499 \\
& =x^2-151 x+149 x-22499 \\
& =x(x-151)+149(x-151) \\
& =(x-151)(x+149)
\end{aligned}\)

WBBSE Class 9 Maths Solutions

Question 15. (x² – y²) (a² – b²) + 4abxy

Solution: (x² – y²) (a² – b²) + 4abxy

\(\begin{aligned}
& =a^2 x^2-b^2 x^2-a^2 y^2+b^2 y^2+4 a b x y \\
& =a^2 x^2+2 a b x y+b^2 y^2-b^2 x^2+2 a b x y-a^2 y^2 \\
& =(a x)^2+2 \cdot a x \cdot b y+(b y)^2-\left(b^2 x^2-2 a b x y+a^2 y^2\right) \\
& =(a x+b y)^2-(b x-a y)^2 \\
& =(a x+b y+b x-a y)(a x+b y-b x+a y)
\end{aligned}\)

WBBSE Class 9 Maths Solutions Chapter 8 Factorisation Exercise 8.3

Question 1. t⁹-512

Solution: t⁹-512

\(\begin{aligned}
& =\left(t^3\right)^3-(8)^3 \\
& =\left(t^3-8\right)\left\{\left(t^3\right)^2+t^3 .8+(8)^2\right\} \\
& =\left\{(t)^3-(2)^3\right\}\left(t^6+8 t^3+64\right) \\
& =(t-2)\left(t^2+2 t+4\right)\left(t^6+8 t^3+64\right)
\end{aligned}\)

Question 2. 729p⁶-q⁶

Solution: 729p⁶-q⁶

\(\begin{aligned}
& =\left(27 p^3\right)^2-\left(q^3\right)^2 \\
& =\left(27 p^3+q^3\right)\left(27 p^3-q^3\right) \\
& =\left\{(3 p)^3+(q)^3\right\}\left\{(3 p)^3-(q)^3\right\} \\
& =(3 p+q)\left\{(3 p)^2-3 p \cdot q+(q)^2\right\}(3 p-q)\left\{(3 p)^2+3 p . q+(q)^2\right\} \\
& =(3 p+q)\left(9 p^2-3 p q+q^2\right)(3 p-q)\left(9 p^2+3 p q+q^2\right) \\
& =(3 p+q)(3 p-q)\left(9 p^2-3 p q+q^2\right)\left(9 p^2+3 p q+q^2\right)
\end{aligned}\)

Question 3. 8(p-3)³+343

Solution: 8(p-3)³+343

\(\begin{aligned}
& =\left\{2(p-3\}^3+(7)^3\right. \\
& =(2 p-6)^3+(7)^3 \\
& =(2 p-6+7)\left\{(2 p-6)^2-(2 p-6) 7+(7)^2\right\} \\
& =(2 p+1)\left\{(2 p)^2-2.2 p \cdot 6+(6)^2-14 p+42+49\right\} \\
& =(2 p+1)\left(4 p^2-24 p+36-14 p+42+49\right) \\
& =(2 p+1)\left(4 p^2-38 p+127\right)
\end{aligned}\)

Question 4. \(\frac{1}{8 a^3}+\frac{8}{b^3}\)

Solution: \(\frac{1}{8 a^3}+\frac{8}{b^3}\)

\(\begin{aligned}
& =\left(\frac{1}{2 a}\right)^3+\left(\frac{2}{b}\right)^3 \\
& =\left(\frac{1}{2 a}+\frac{2}{b}\right)\left\{\left(\frac{1}{2 a}\right)^2-\frac{1}{2 a} \cdot \frac{2}{b}+\left(\frac{2}{b}\right)^2\right\} \\
& =\left(\frac{1}{2 a}+\frac{2}{b}\right)\left(\frac{1}{4 a^2}-\frac{1}{a b}+\frac{4}{b^2}\right)
\end{aligned}\)

Question 5.(2a³-b³)³-b⁹

Solution: (2a³-b³)³-b⁹

\(\begin{aligned}
& =\left(2 a^3-b^3\right)^3-\left(b^3\right)^3 \\
& =\left(2 a^3-b^3-b^3\right)\left\{\left(2 a^3-b^3\right)^2+\left(2 a^3-b^3\right) b^3+\left(b^3\right)^2\right\} \\
& =\left(2 a^3-2 b^3\right)\left\{\left(2 a^3\right)^2-2 \cdot 2 a^3 \cdot b^3+\left(b^3\right)^2+2 a^3 b^3-b^6+b^6\right\} \\
& =2\left(a^3-b^3\right)\left(4 a^6-4 a^3 b^3+b^6+2 a^3 b^3\right) \\
& =2(a-b)\left(a^2+a b+b^2\right)\left(4 a^6-2 a^3 b^3+b^6\right)
\end{aligned}\)

Question 6. AR³-Ar³+ AR²h – Ar²h

Solution: AR³-Ar³+ AR²h – Ar²h

\(\begin{aligned}
& =A\left(R^3-r^3\right)+A h\left(R^2-r^2\right) \\
& =A(R-r)\left(R^2+R r+r^2\right)+A h(R+r)(R-r) \\
& =A(R-r)\left\{R^2+R r+r^2+h(R+r)\right\} \\
& =A(R-r)\left(R^2+R r+r^2+h R+h r\right)
\end{aligned}\)

Question 7. \(a^3+3 a^2 b+3 a b^2+b^3-8\)

Solution:

\(\begin{aligned}
&a^3+3 a^2 b+3 a b^2+b^3-8 \\
& =(a+b)^3-(2)^3 \\
& =(a+b-2)\left\{(a+b)^2+(a+b) \cdot 2+(2)^2\right\} \\
& =(a+b-2)\left(a^2+2 a b+b^2+2 a+2 b+4\right)
\end{aligned}\)

Question 8. \(32 x^4-500 x\)

Solution:

\(\begin{aligned}
& 32 x^4-500 x \\
& =4 x\left(8 x^3-125\right) \\
& =4 x\left\{(2 x)^3-(5)^3\right\} \\
& =4 x(2 x-5)\left\{(2 x)^2+2 x .5+(5)^2\right\} \\
& =4 x(2 x-5)\left(4 x^2+10 x+25\right)
\end{aligned}\)

Class 9 Mathematics West Bengal Board

Question 9. \(8 a^3-b^3-4 a x+2 b x\)

Solution:

\(\begin{aligned}
&8 a^3-b^3-4 a x+2 b x \\
& =(2 a)^3-(b)^3-2 x(2 a-b) \\
& =(2 a-b)\left\{(2 a)^2+2 a \cdot b+(b)^2\right\}-2 x(2 a-b) \\
& =(2 a-b)\left(4 a^2+2 a b+b^2-2 x\right)
\end{aligned}\)

 

Question 10. \(x^3-6 x^2+12 x-35\)

Solution:

\(\begin{aligned}
&x^3-6 x^2+12 x-35 \\
& =(x)^3-3 \cdot x^2 \cdot 2+3 \cdot x \cdot(2)^2-(2)^3-27 \\
& =(x-2)^3-(3)^3 \\
& =(x-2-3)\left\{(x-2)^2+(x-2) 3+(3)^2\right\} \\
& =(x-5)\left(x^2-4 x+4+3 x-6+9\right) \\
& =(x-5)\left(x^2-x+7\right)
\end{aligned}\)

Class 9 Mathematics West Bengal Board Chapter 8 Factorisation Exercise 8.4

 

Question 1. \(8 x^3-y^3+1+6 x y\)

Solution:

\(\begin{aligned}
& =(2 x)^3+(-y)^3+(1)^3-3.2 x(-y) \cdot 1 \\
& =(2 x-y+1)\left\{(2 x)^2+(-y)^2+(1)^2-2 x(-y)-(-y) \cdot 1-1.2 x\right\} \\
& =(2 x-y+1)\left(4 x^2+y^2+1+2 x y+y-2 x\right) \\
& =(2 x-y+1)\left(4 x^2+y^2+1-2 x+y+2 x y\right)
\end{aligned}\)

 

Question 2. \(8 a^3-27 b^3-1-18 a b\)

Solution:

\(\begin{aligned}
& 8 a^3-27 b^5-1-18 a d \\
& =(2 a)^3+(-3 b)^3+(-1)^3-3 \cdot 2 a \cdot(-3 b)(-1) \\
& =(2 a-3 b-1)\left\{(2 a)^2+(-3 b)^2+(-1)^2-2 a(-3 b)-(-3 b)(-1)-(-1) \cdot 2 a\right\} \\
& =(2 a-3 b-1)\left(4 a^2+9 b^2+1+6 a b-3 b+2 a\right) \\
& =(2 a-3 b-1)\left(4 a^2+9 b^2+1+2 a-3 b+6 a b\right) \\
&
\end{aligned}\)

Class 9 Mathematics West Bengal Board

Question 3. \(1+8 x^3+18 x y-27 y^3\)

Solution:

\(\begin{aligned}
& =8 x^3-27 y^3+1+18 x y \\
& =(2 x)^3+(-3 y)^3+(1)^3-3 \cdot 2 x(-3 y) .1 \\
& =(2 x-3 y+1)\left\{(2 x)^2+(-3 y)^2+(1)^2-2 x \cdot(-3 y)-(-3 y) .1-1.2 x\right\} \\
& =(2 x-3 y+1)\left(4 x^2+9 y^2+1+6 x y+3 y-2 x\right) \\
& =(2 x-3 y+1)\left(4 x^2+9 y^2+1-2 x+3 y+6 x y\right)
\end{aligned}\)

 

Question 4. \(x^3+y^3-12 x y+64\)

Solution:

\(\begin{aligned}
& =x^3+y^3+64-12 x y \\
& =(x)^3+(y)^3+(4)^3-3 \cdot x \cdot y \cdot 4 \\
& =(x+y+4)\left\{(x)^2+(y)^2+(4)^2-x \cdot y-y \cdot 4-4 \cdot x\right\} \\
& =(x+y+4)\left(x^2+y^2+16-x y-4 y-4 x\right) \\
& =(x+y+4)\left(x^2+y^2+16-4 x-4 y-x y\right)
\end{aligned}\)

Class 9 Mathematics West Bengal Board

Question 5. \((3 a-2 b)^3+(2 b-5 c)^3+(5 c-3 a)^3\)

Solution:

 

 

Question 6. \((2 x-y)^3-(x+y)^3+(2 y-x)^3\)

Solution:

 

Let a = 2x-y, b= -(x + y), c = 2y –x

a + b + c = 2x – y – x – y + 2y – x =0

a³ + b³ = c³ = 3abc

= 3(2x –y) {-(x + y)} (2y –x)

= 3(2x –y) (x + y) (x–2y)

 

Class 9 Mathematics West Bengal Board

Question 7. \(a^6+32 a^3-64\)

Solution:

\(\begin{aligned}
& a^6+32 a^3-64 \\
& =\left(a^2\right)^3+(2 a)^3+(-4)^3-3 a^2 .2 a(-4) \\
& =\left(a^2+2 a-4\right)\left\{\left(a^2\right)^2+(2 a)^2+(-4)^2-a^2 .2 a-2 a(-4)-(-4) a^2\right\} \\
& =\left(a^2+2 a-4\right)\left(a^4+4 a^2+16-2 a^3+8 a+4 a^2\right) \\
& =\left(a^2+2 a-4\right)\left(a^4-2 a^3+8 a^2+8 a+16\right)
\end{aligned}\)

 

Question 8. \(a^6-18 a^3+125\)

Solution:

\(\begin{aligned}
&a^6-18 a^3+125 \\
& =\left(a^2\right)^3+(3 a)^3+(5)^3-3 \cdot a^2 \cdot 3 a \cdot 5 \\
& =\left(a^2+3 a+5\right)\left\{\left(a^2\right)^2+(3 a)^2+(5)^2-a^2 \cdot 3 a-3 a \cdot 5-5 \cdot a^2\right\} \\
& =\left(a^2+3 a+5\right)\left(a^4+9 a^2+25-3 a^3-15 a-5 a^2\right) \\
& =\left(a^2+3 a+5\right)\left(a^4-3 a^3+4 a^2-15 a+25\right) \\
&
\end{aligned}\)

 

Question 9. \(p^3(q-r)^3+q^3(r-p)^3+r^3(p-q)^3\)

Solution:

\begin{aligned}
& p^3(q-r)^3+q^3(r-p)^3+r^3(p-q)^3 \\
& =\{p(q-r)\}^3+\{q(r-p)\}^3+\{r(p-q)\}^3 \\
& \text { Let } a=p(q-r), b=q(r-p), c=r(p-q) \\
& ∴ a+b+c=p(q-r)+q(r-p)+r(p-q) \\
& =p q-p r+q r-p q+p r-q r \\
& =0 \\
& ∴a^3+b^3+c^3-3 a b c=0 \\
& ∴ a^3+\dot{b}^3+c^3=3 a b c \\
&∴ \{p(q-r)\}^3+\{q(r-p)\}^3+\{r(p-q)\}^3 \text {. } \\
& =3 p(q-r) \cdot q(r-p) r(p-q) \\
& =3 p q r(p-q)(q-r)(r-p) \\
&
\end{aligned}

Class 9 Mathematics West Bengal Board

Question 10. \(p^3+\frac{1}{p^3}+\frac{26}{27}\)

Solution:

\(\begin{aligned}
& p^3+\frac{1}{p^3}+\frac{26}{27} \\
& =p^3+\frac{1}{p^3}+\frac{27-1}{27} \\
& =p^3+\frac{1}{p^3}+\frac{27}{27}-\frac{1}{27} \\
& =p^3+\frac{1}{p^3}+1-\frac{1}{27}
\end{aligned}\)

Class 9 Maths WBBSE

\(\begin{aligned}
& =(p)^3+\left(\frac{1}{p}\right)^0+\left(-\frac{1}{3}\right)^0-3 \cdot p \cdot \frac{1}{p}\left(-\frac{1}{3}\right) \\
& =\left(p+\frac{1}{p}-\frac{1}{3}\right)\left\{(p)^2+\left(\frac{1}{p}\right)^2+\left(-\frac{1}{3}\right)^2-p \cdot \frac{1}{p}-\frac{1}{p} \cdot\left(-\frac{1}{3}\right)-\left(-\frac{1}{3}\right) \cdot p\right\}
\end{aligned}\)

 

\(\begin{aligned}
& =\left(p+\frac{1}{p}-\frac{1}{3}\right)\left(p^2+\frac{1}{p^2}+\frac{1}{9}-1+\frac{1}{3 p}+\frac{p}{3}\right) \\
& =\left(p+\frac{1}{p}-\frac{1}{3}\right)\left(p^2+\frac{1}{p^2}+\frac{p}{3}+\frac{1}{3 p}-\frac{8}{9}\right)
\end{aligned}\)

Class 9 Maths WBBSE Chapter 8 Factorisation Exercise 8.5

Question 1. \((a+b)^2-5 a-5 b+6\)

Solution:

\(
\begin{aligned}
& (a+b)^2-5 a-5 b+6 \\
& =(a+b)^2-5(a+b)+6
\end{aligned}
Let a+b=x\)

 

\(\begin{aligned}
& ∴ x^2-5 x+6 \\
& =x^2-(3+2) x+6 \\
& =x^2-3 x-2 x+6 \\
& =x(x-3)-2(x-3) \\
& =(x-3)(x-2)
\end{aligned}\)

pitting the value of x the given expression becomes (a+b-3)(a+b-2)

Question 2. (x+1)(x+2)(3x-1)(3x-4) +12

Solution: (x+1)(x+2)(3x-1)(3x-4) +12
=(x+1)(3x-1)(x+2)(3x-4)+12

\(\begin{aligned}
& =\left(3 x^2-x+3 x-1\right)\left(3 x^2-4 x+6 x-8\right)+12 \\
& =\left(3 x^2+2 x-1\right)\left(3 x^2+2 x-8\right)+12
\end{aligned}\)

 

\(\begin{aligned}
& \text { Let } 3 x^2+2 x=a \\
& \qquad \begin{array}{l}
therefore(a-1)(a-8)+12 \\
\quad=a^2-8 a-a+8+12 \\
\quad=a^2-9 a+20 \\
\quad=a^2-5 a-4 a+20 \\
\quad=a(a-5)-4(a-5) \\
\quad=(a-5)(a-4)
\end{array}
\end{aligned}\)

putting the value of a,

\(\begin{aligned}
& \left(3 x^2+2 x-5\right)\left(3 x^2+2 x-4\right) \\
& =\left(3 x^2+5 x-3 x-5\right)\left(3 x^2+2 x-4\right) \\
& =\{x(3 x+5)-1(3 x+5)\}\left(3 x^2+2 x-4\right) \\
& =(3 x+5)(x-1)\left(3 x^2+2 x-4\right)
\end{aligned}\)

Class 9 Maths WBBSE

Question 3. \(x\left(x^2-1\right)(x+2)-8\)

Solution:

\(\begin{aligned}
& x\left(x^2-1\right)(x+2)-8 \\
& =x(x+1)(x-1)(x+2)-8 \\
& =\left(x^2+x\right)\left(x^2+2 x-x-2\right)-8 \\
& =\left(x^2+x\right)\left(x^2+x-2\right)-8
\end{aligned}\)

 

Let,\(\begin{aligned}
& x^2+x=a \\
& therefore \mathrm{a}(\mathrm{a}-2)-8 \\
& =\mathrm{a}^2-2 \mathrm{a}-8 \\
& =a^2-(4-2) a-8 \\
& =a^2-4 a+2 a-8 \\
& =a(a-4)+2(a-4) \\
& =(a-4)(a+2) \\
&
\end{aligned}\)

 

Pitting the value of a, the given expression becomes \(\left(x^2+x-4\right)\left(x^2+x+2\right)\)

Question 4. \(7\left(a^2+b^2\right)^2-15\left(a^4-b^4\right)+8\left(a^2-b^2\right)^2\)

Solution:

 

\(\begin{aligned}
& =7 x^2-7 x y-8 x y+8 y^2 \\
& =7 x(x-y)-8 y(x-y) \\
& =(x-y)(7 x-8 y)
\end{aligned}\)

Putting the value of x and y, the given expression becomes

\(\begin{aligned}
& \left(a^2+b^2-a^2+b^2\right)\left(7 a^2+7 b^2-8 a^2+8 b^2\right) \\
& =2 b^2\left(15 b^2-a^2\right)
\end{aligned}\)

Class 9 Maths WBBSE

Question 5. \(\left(x^2-1\right)^2+8 x\left(x^2+1\right)+19 x^2\)

Solution:

\(\begin{aligned}
&\left(x^2-1\right)^2+8 x\left(x^2+1\right)+19 x^2 \\
& =\left(x^2+1\right)^2-4 \cdot x^2 \cdot 1+8 x\left(x^2+1\right)+19 x^2 \\
& =\left(x^2+1\right)^2+8 x\left(x^2+1\right)+15 x^2
\end{aligned}\)

 

Let,\(\begin{aligned}
& \text { Let } x^2+1=a \\
& \quad therefore a^2+8 a x+15 x^2 \\
& =a^2+(5+3) a x+15 x^2 \\
& =a^2+5 a x+3 a x+15 x^2 \\
& =a(a+5 x)+3 x(a+5 x) \\
& =(a+5 x)(a+3 x)
\end{aligned}\)

 

Putting the value of a the given expression becomes

\(\begin{aligned}
& \left(x^2+1+5 x\right)\left(x^2+1+3 x\right) \\
& =\left(x^2+5 x+1\right) \cdot\left(x^2+3 x+1\right)
\end{aligned}\)

 

Question 6. \((a-1) x^2-x-(a-2)\)

Solution:

\(\begin{aligned}
& (a-1) x^2-x-(a-2) \\
&=(a-1) x^2-\{(a-1)-(a-2)\} x-(a-2) \\
&=(a-1) x^2-(a-1) x+(a-2) x-(a-2) \\
&=(a-1) x(x-1)+(a-2)(x-1) \\
&=(x-1)\{(a-1) x+(a-2)\} \\
&=(x-1)(a x-x+a-2)
\end{aligned}\)

Class 9 Maths WBBSE

Question 7. \((a-1) x^2+a^2 x y+(a+1) y^2\)

Solution:

\(\begin{aligned}
&(a-1) x^2+a^2 x y+(a+1) y^2 \\
& =(a-1) x^2+\left\{\left(a^2-1\right)+1\right\} x y+(a+1) y^2 \\
& =(a-1) x^2+\left(a^2-1\right) x y+x y+(a+1) y^2 \\
& =(a-1) x^2+(a+1)(a-1) x y+x y+(a+1) y^2 \\
& =(a-1) x\{x+(a+1) y\}+y\{x+(a+1) y\} \\
& =\{x+(a+1) y\}\{(a-1) x+y\} \\
& =(x+a y+y)(a x-x+y)
\end{aligned}\)

 

Question 8. \(x^2-q x-p^2+5 p q-6 q^2\)

Solution:

\(\begin{aligned}
& x^2-q x-p^2+5 p q-6 q^2 \\
& =x^2-q x-\left(p^2-5 p q+6 q^2\right) \\
& =x^2-q x-\left(p^2-3 p q-2 p q+6 q^2\right) \\
& =x^2-q x-\{p(p-3 q)-2 q(p-3 q)\} \\
& =x^2-q x-(p-3 q)(p-2 q) \\
& =x^2-\{(p-2 q)-(p-3 q)\} x-(p-3 q)(p-2 q) \\
& =x^2-(p-2 q) x+(p-3 q) x-(p-3 q)(p-2 q) \\
& =x(x-p+2 q)+(p-3 q)(x-p+2 q) \\
& =(x-p+2 q)(x+p-3 q)
\end{aligned}\)

 

Question 9. \(2\left(a^2+\frac{1}{a^2}\right)-\left(a-\frac{1}{a}\right)-7\)

Solution:

\(2\left(a^2+\frac{1}{a^2}\right)-\left(a-\frac{1}{a}\right)-7
\begin{aligned}
& =2\left\{(a)^2+\left(\frac{1}{a}\right)^2\right\}-\left(a-\frac{1}{a}\right)-7 \\
& =2\left\{\left(a-\frac{1}{a}\right)^2+2 \cdot a \cdot \frac{1}{a}\right\}-\left(a-\frac{1}{a}\right)-7
\end{aligned}
\)

 

\(\begin{aligned}
& \text { Let } a-\frac{1}{a}=x \\
& \qquad \begin{aligned}
therefore & 2\left(x^2+2\right)-x-7 \\
= & 2 x^2+4-x-7 \\
= & 2 x^2-x-3 \\
= & 2 x^2-3 x+2 x-3 \\
= & x(2 x-3)+1(2 x-3) \\
= & (2 x-3)(x+1)
\end{aligned}
\end{aligned}\)

 

Putting the value of a, the given expression becomes

\(\begin{aligned}
& =\left\{2\left(a-\frac{1}{a}\right)-3\right\}\left(a-\frac{1}{a}+1\right) \\
& =\left(2 a-\frac{2}{a}-3\right)\left(a-\frac{1}{a}+1\right)
\end{aligned}\)

 

Question 10. \(\left(x^2-x\right) y^2+y-\left(x^2+x\right)\)

Solution:

\(\begin{aligned}
& \left(x^2-x\right) y^2+y-\left(x^2+x\right) \\
& =x(x-1) y^2+y-x(x+1) \\
& =x(x-1) y^2+\left\{x^2-\left(x^2-1\right)\right\} y-x(x+1) \\
& =x(x-1) y^2+x^2 y-\left(x^2-1\right) y-x(x+1) \\
& =x y\{(x-1) y+x\}-(x+1)\{(x-1) y+x\} \\
& =\{(x-1) y+x\}\{x y-(x+1)\} \\
& =(x y-y+x)(x y-x-1)
\end{aligned}\)

 

Question 11. Multiple Choice Questions

1)If a² – b²= 11 x 9; a and b are positive integers (a> b) then

(1) a = 11, b = 9
(2) a = 33, b = 3
(3) a = 10, b = 1
(4) a = 100, b = 1

Solution: a² – b²= 11 x 9
or, (a + b) (a – b) (10+ 1) (10-1)
∴ a = 10, b = 1

∴ (3) a = 10, b = 1

2) If \(\frac{a}{b}+\frac{b}{a}=1\) then the value of a³ + b³ is

(1)1
(2)a
(3)b
(4)0

Solution: \(\frac{a}{b}+\frac{b}{a}=1\)

or, \(\frac{a^2+b^2}{a b}=1\)

 

or,\(\begin{aligned}
& a^2+b^2=a b \\
& a^2-a b+b^2=0 \\
& therefore a^3+b^3 \\
& =(a+b)\left(a^2-a b+b^2\right) \\
& =(a+b) \times 0 \\
& =0
\end{aligned}\)

∴ (4) 0

3)The value of \(25^3-75^3+50^3+3 \times 25 \times 75 \times 50\) is

(1) 150
(2) 0
(3) 25
(4) 50

Solution: Let a = 25, b=-75 and c =
∴ a+b+c=25-75+ 50 = 0

\(\begin{aligned}
& therefore a^3+b^3+c^3-3 a b c=(a+b+c)\left(a^2+b^2+c^2-a b-b c-c a\right) \\
& =0 \times\left(a^2+b^2+c^2-a b-b c-c a\right) \\
& =0 \\
& therefore 25^3-75^3+50^3+3 \times 25 \times 75 \times 50 \\
& =(25)^3+(-75)^3+(50)^3-3.25 .(-75) .50 \\
& =0
\end{aligned}\)

4) If a + b + c = 0, then the value of \(\frac{a^2}{b c}+\frac{b^2}{c a}+\frac{c^2}{a b}\) is

(1) 0
(2) 1
(3) 1
(4) 3

Solution: a+b+c=0
∴ a³+b³+ c³-3abc = 0
or, a³+b³+ c³= 3abc

or, \(\frac{a^3+b^3+c^3}{a b c}=\frac{3 a b c}{a b c}\)

or, \(\frac{a^3}{a b c}+\frac{b^3}{a b c}+\frac{c^3}{a b c}=3\)

or, \(\frac{a^2}{b c}+\frac{b^2}{a c}+\frac{a^2}{a b}=3\)

∴ (4)3

5) If p²– px + 12 = (x-3) (x – a) is an identity, then the values of a and p are respectively
(1) a = 4, p = 7
(2) a = 7, p = 4
(3) a = 4, p = -7
(4) a = 4, p = 7

Soltion:  p²-px+12= (x-3) (x – a)
or,  p²-px+12=x²-ax-3x + 3a
or, p²-px+12= x²– (a + 3)x + 3a
∴ 3a= 12

or, a =12/3 =4
Again, a + 3 = p
or, 4+ 3 = p
or, p=7

(1) a = 4, p = 7

Question 12. Short answer type questions:

(1) Let us write the simplest value of

\(\frac{\left(b^2-c^2\right)^3+\left(c^2-a^2\right)^3+\left(a^2-b^2\right)^3}{(b-c)^3+(c-a)^3+(a-b)^3}\)

Solution:

 

 

(2) Let us write the relation between a, b, and c if a³ + b³ + c³-3abc = 0 and a+b+c ≠ 0

Solution:

 

 

(3 ) If a²-b² = 224 and a and b are negative integers (a<b), then let us write the values of a and b.

Solution :

 

 

(4) Let us write the value of \((x-a)^3+(x-b)^3+(x-c)^3-3(x-a)(x-b)(x-c)\) if 3x= a+b+c.

Solution: 3x=a+b+c
or, 3x-a-b-c=0
or, x-a+x-b+x-c=0
Let x-a = p, x-b=q and x-c=r
∴ p+q+r=0

\(\begin{aligned}
& therefore p^3+q^3+r^3-3 p q r=0 \\
& therefore(x-a)^3+(x-b)^3+(x-c)^3-3(x-a)(x-b)(x-c)=0
\end{aligned}\)

 

(5) Let us write the values of a and p if 2x²+ px + 6 = (2x – a) (x-2) is an identity.

Solution:

\(2 x^2+p x+6=(2 x-a)(x-2)\)

 

\(\text { or, } 2 x^2+p x+6=2 x^2-4 x-a x+2 a\)

 

\(\text { or, } 2 x^2+p x+6=2 x^2-(4+a) x+2 a\)

 

\(\begin{aligned}
& therefore 2 a=6 \\
& \text { or, } a=\frac{6}{2}=3
\end{aligned}\)

 

Again,\(p=-(4+a)=-(4+3) or, p=-7
therefore a=3, p=-7
\)

 

 

Class IX Maths Solutions WBBSE Chapter 9 Transversal And Mid Point Theorem Exercise 9

Question 1. In the triangle ABC, D is the midpoint of the side BC. From point D, the parallel straight lines CA and BA intersect the sides BA and CA at points E and F respectively, Let us prove that, \(E F=\frac{1}{2} B C\)

Solution: In ΔABC the midpoint of side D is BC. From point D, CA and BA parallel to CA and BA are drawn which intersect BA at point E and CA at point F.

Prove that \(E F=\frac{1}{2} B C\)

 

 

Proof: DF II BA (Given) and D are the midpoint of BC
∴ Point F is the midpoint of side AC.
Similarly, DE II CA and point D is the midpoint of BC.
∴ point E is the midpoint of AB

Read and Learn More WBBSE Solutions For Class 9 Maths

Now E and F points respectively are mid points of AB and AC sides ΔABC.

∴ \(E F=\frac{1}{2} B C\)

Question 2. D and E lie on AB and AC respectively of the triangle ABC such that \(A D=\frac{1}{4} A B\) and \(A E=\frac{1}{4} A C\). Let us prove that DE II BC and \(D E=\frac{1}{4} B C\)

Solution: In ΔABCD and F lie on sides AB and AC respectively such that \(A D=\frac{1}{4} A B\) and \(A E=\frac{1}{4} A C\).

Prove that DE II BC and \(D E=\frac{1}{4} B C\)

 

 

Construction: Midpoints of sides F AB and AC, G and are joined.

Proof: In ΔABC the midpoints of sides AB and AC are F and G.

∴ FG II BC and \(F G=\frac{1}{2} B C\)

∴ F is the and midpoint of AB \(A D=\frac{1}{4} A B\)

Class IX Maths Solutions WBBSE

∴ D is the midpoint of AF.
Similarly, E is the midpoint of AG.
So, in ΔAFG the midpoints of sides AF and AG are respectively D and E

∴ DE II FG and FG II BC.
∴ DE II BC.

Again, \(D E=\frac{1}{2} F G=\frac{1}{2} \times \frac{1}{2} B C=\frac{1}{4} B C\)

Question 3. In the triangle PQR, the midpoints of the sides QR and QP are X and Z respectively. The side QP is extended upto the point S so that PS = ZP. SX intersects the side PR at the point Y. Let us prove that PY = \(P Y=\frac{1}{4} P R\)

Solution: In ΔPQR the midpoints of sides QR and PQ are X and Z. Side QP is extended up to points S so that PS = ZP. SX, at Y, intersects the side PR.

Prove that PY = \(P Y=\frac{1}{4} P R\)

 

 

Construction: X, and Y are joined.

Proof: Of QP and QR the midpoints are Z and X.

∴ ZX II PR and \(Z X=\frac{1}{2} P R\)

Now, in ΔSZX the midpoint SZ is and P and PY II XZ.
∴ Y is the midpoint of SX.

∴ \(P Y=\frac{1}{2} Z X\)       \(\left[because \mathrm{ZX}=\frac{1}{2} \mathrm{PR}\right]\)

or, \(P Y=\frac{1}{2} \times \frac{1}{2} P R\)

or, \(P Y=\frac{1}{4} P R\)

Class IX Maths Solutions WBBSE

Question 4. Let us prove that the quadrilateral formed by joining mid-points of the consecutive sides of a parallelogram is a parallelogram.

Solution: Let in the quadrilateral of ABCD the midpoints sides AB, BC, CD, and DA are P, Q, R, and S respectively joining. PQ, QR, RS and SP we get PQRS quadrilateral.

Prove that PQRS is a parallelogram.

 

 

 

Construction: diagonal BD is drawn.

Proof: In ΔABD the midpoints of sides AB and AD are P and S respectively.

∴ PS II BD and \(P S=\frac{1}{2} B D\)

Similarly, in ΔCBD the midpoints of sides CB and CD are Q and R respectively.

∴ QR II BD and \(\mathrm{QR}=\frac{1}{2} \mathrm{BD}\)

∴ PS = QR
Now, in quadrilateral PQRS, PS II QR and PS = QR. So, PQRS is a parallelogram.

Class IX Maths Solutions WBBSE

Question 5. Let us prove that the quadrilateral formed by joining the mid-points of the consecutive sides of a rectangular figure is not a square figure but a rhombus.

Solution: Let ABCD is a rectangle whose midpoints of sides AB, BC, CD, and DA are P, Q, R, and S respectively P, Q; Q, R; R, S; and S, P are joined.

Prove that PQRS is a rhombus, not a square.

 

 

Construction: P, R, and S, Q are joined.

Proof: In ΔAPS and ΔPBQ,
AP = BP, AS BQ [By construction]
∠PAS ≅ ∠PBQ [Both are right angles]

∴ ΔPAS ≅ ΔPBQ (S-A-S congruency)
∴ PS = PQ

Similarly, in ΔPBQ and ΔRCQ, PQ = QR
In ΔQRC and ΔRCD, QR = RS
In ΔRSD and ΔAPS, RS = PS
∴ PQ = QR RS = SP

Now, in quadrilateral PQRS, PQ = QR = RS = SP
But PR > SQ.
∴ The four sides of quadrilateral PQRS are equal but the diagonals are not. So is a rhombus but not a square.

Class IX Maths Solutions WBBSE

Question 6. Let us prove that the quadrilateral formed by joining the mid-points of consecutive sides of a square is a square.

Solution: Let the midpoints of sides AB, BC, CD, and DA of the square ABCD be P, Q, R & S respectively. P, Q; Q, R; R, S, and S, P are joined.

Prove that PQRS is a square.

 

 

Construction: Diagonals AC and BD are joined which intersect each other at O. In □PQRS, PQ cuts diagonal BD at point F and PS cuts diagonal AC at point E.

Proof: In ΔADC the midpoint of AD is S and the midpoint DC is R.

∴ SR II AC and \(S R=\frac{1}{2} A C\)  ….(1)

Similarly, in ΔABC, PQ II AC and \(P Q=\frac{1}{2} A C\) …(2)

∴ PQ II SR and PQ = SR.
∴ PQRS is a parallelogram.

Class 9 Mathematics West Bengal Board

∴ In ΔABD the midpoints of sides AB and AD are P and S respectively.

∴ \(P S=\frac{1}{2} B D\). But ACBD.

∴ \(P Q=\frac{1}{2} A C=\frac{1}{2} B D=P S\)

∴ In Parallelogram PQRS the length of adjacent sides are equal.
∴PQRS is a rhombus.
∴ PE II FO and PF II EO.
∴ ∠EOF= 90°
∴ ∠EPF = 90°
∴ PQRS is a square.

Question 7. Let us prove that the quadrilateral formed by joining the mid-points of a rhombus is a rectangle.

Solution: Let in rhombus ABCD the midpoints of sides AB, BC, CD, and DA are E, F, G, and H respectively. E, F; F, G; G, H, and H, E are joined.

Prove that EFGH is a rectangle.

 

 

Construction: B, D; A, C; E, G and H, F are joined.

Proof: In ΔABD the midpoints of sides AB and AD are E and H respectively.

∴ EH II BD and \(\mathrm{EH}=\frac{1}{2} \mathrm{BD}\)

Class 9 Mathematics West Bengal Board

Similarly, in ΔBCD the midpoints of sides BC and CD are F and G respectively.∴ FG II BD and FG = 1BD

∴ EH II FG and \(\)

∴ EFGH is a parallelogram.

Now, in □ABFH, AH II BF and AH = BF
∴ ABFH is a parallelogram.
∴ AB = HF

Similarly, ADGE is a parallelogram.
∴ AD = EG
But AB = AD ( ABCD is a rhombus)
∴ FH = EG

∴ Diagonals of the parallelogram EFGH are equal in length.
∴ EFGH is a rectangle.

Question 8. In the triangle ABC, the mid-points of AB and AC are D and E respectively; the mid-points of CD and BD are P and Q respectively. Let us prove that BE and PQ bisect each other.

Solution: In ΔABC the midpoints of sides AB and AC are D and E respectively and P & Q are the midpoints of CD and BD respectively.

Prove that BE and PQ bisect each other.

 

 

Construction: B, P; P, E; E, Q and P, Q bisect each other.

Proof: In ΔACD the midpoints of sides AC and CD are E and P respectively.

∴ PE II AD and \(\mathrm{PE}=\frac{1}{2} \mathrm{AD}\)

Again, AD = BD and \(B Q=\frac{1}{2} B D\)

(∵ D is the midpoint of AB and Q the midpoint of BD)

∴ \(P E=\frac{1}{2} A D=\frac{1}{2} B D=B Q\)

In □BPEQ, PE II BQ and PE = BQ
∴ BPEQ is a parallelogram whose diagonals are BE and PQ.
∴ BE and PQ bisect each other.

Class 9 Mathematics West Bengal Board

Question 9. In the triangle ABC, AD is perpendicular on the bisector of ZABC. From point D, a straight line DE parallel to side BC is drawn which intersects side AC at point E. Let us prove that AE = EC.

Solution: In triangle ABC on the bisector of ZABC AD is perpendicular. From point D parallel to BC of parallel straight line DE is drawn which intersects AC side at point E.

Prove that AE = EC.

 

 

Construction: AD is extended forwards which at intersects BC point F.

Proof: In ΔABD and ΔBDF,
∠BDA = ∠BDF (∵ AD ⊥ BD)
∠ABD = ∠FBD (∵ ∠ZABC is the bisector of BD) and BD is a common side.

∴ ΔABD ≅ ΔBDF (A-A-S Congrency)
∴ AD DF, that is D is the midpoint of AF

In ΔAFC of AF D is the midpoint and DE II FC.
∴ E is the midpoint of AC
∴ AE = EC

Class 9 Maths WB Board

Question 10. In the triangle ABC, AD is a median. From points B and C, two straight lines BR and CT, parallel to AD are drawn, which meet extended BA and CA at points T and R respectively. Let us prove that \(\frac{1}{A D}=\frac{1}{R B}+\frac{1}{T C}\)

Solution: In a triangle, ABC AD is the median. from points BC and parallel to AD straight lines BR and CT are drawn which intersect extended BA and CA at points T and R.

Prove that = \(\frac{1}{A D}=\frac{1}{R B}+\frac{1}{T C}\)

 

 

Proof: In AD is the median
∴ D, is the midpoint of BC

In ΔBCT the midpoint of BC is D and DA II CT
∴ A is the midpoint of BT

∴ AD II CT and \(\mathrm{AD}=\frac{1}{2} \mathrm{TC}\)
∴ TC= 2AD

Now, in ΔBCR the mid point of BC is D and DA II BR
∴ A is the midpoint of RC

Class 9 Maths WB Board

∴ AD II RB and \(A D=\frac{1}{2} R B\)

∴ RB = 2AD

\(\frac{1}{\mathrm{RB}}+\frac{1}{T C}=\frac{1}{2 A D}+\frac{1}{2 A D}=\frac{2}{2 A D}=\frac{1}{A D}\) \(\frac{1}{A D}=\frac{1}{R B}+\frac{1}{T C}\)

 

Question 11. In the trapezium ABCD, AB II DC and AB > DC; the mid-points of two diagonals AC and BD are E and F respectively. Let us prove that \(E F=\frac{1}{2}(A B-D C)\)

Solution: In trapezium ABCD AB II DC and AB > DC; E and F are the midpoints of AC and BD diagonals respectively.

Prove that \(E F=\frac{1}{2}(A B-D C)\)

 

 

Construction: E, and F are joined. CF is extended forwards which intersect AB at point G point.

Proof: In ΔCFD and ΔBFG,
∠CDF = alternate ∠FBG (∴ DC II AB and BD is transversal)
∠CFD = VOA ∠BFG and DF = BF (∵ F is the midpoint of BD).
∴ ΔCFD ≅ ΔBFG (A -A – S congruency)
∴ DC = GB and CF = FG

Now, in ΔACG the midpoints of sides AC and CG are E and F respectively

∴ EF II AG and \(E F=\frac{1}{2} A G\)

∴ \(E F=\frac{1}{2} A G=\frac{1}{2}(A B-G B)\)

∴ \(=\frac{1}{2}(A B-D C)(because G B=D C)\)

Class 9 Maths WB Board

12. C is the mid-point of the line segment AB and PQ is any straight line. The minimum distances of the line PQ from points A, B, and C are AR, BS, and CT respectively; let us prove that AR + BS = 2CT.

Solution: The midpoint of st. line AB is C and PQ is any st. line. from points A, B, and C the minimum distance of straight line PQ are respectively AR, BS, and CT.

Prove that AR + BS = 2CT

 

 

Proof:  ∵AR, BS, and CT are perpendicular on PQ
∴AR II BS II CT

In ΔABR of side AB, the midpoint is C and CM II AR
∴ M, BR is the midpoint

Again, in ΔBRS of BR is the midpoint M and MT II BS
∴ T, RS is the midpoint of
∴ RT=TS

In ΔABR we get, \(C M=\frac{1}{2} A R\)
∴ AR = 2CM

Again, in ΔBRS we get \(M T=\frac{1}{2} B S\)
Or, BS = 2MT
∴ AR+ BS 2CM + 2MT = 2(CM + MT) = 2CT Proved

Class 9 Math Solution WBBSE

Question 13. In a triangle ABC, D is the mid-point of the side BC; through point A, PQ is any straight line. The perpendiculars from the points B, C, and D on PQ are BL, CM, and DN respectively; let us prove that, DL = DM.

Solution:

 

 

Construction: D, Land D, and M are joined.

Proof:  On a straight line PQ BL, CM, and DN are perpendicular.
∴ BL II DN II CM
∵ BD = DC ( ∵D, is the midpoint of BC)
∴ LN = NM

In ΔDLN and ΔDMN, LN = NM Proved
∠DNL = ∠DNM  and DN is a common side. (∵ DN ⊥ PQ)
∴ ΔDLN ≅ ΔDMN (S-A-S Congruency)
∴ DL = DM

Ganit Prakash Class 9 Solutions

Question 14. ABCD is a square figure. The two diagonals AC and BD intersect each other at point O. The bisector of ∠BAC intersects BO at the point P and BC at point Q. Let us prove that \(O P=\frac{1}{2} C Q\)

Solution:

 

 

Construction from point C parallel to OP an st. line CR is drawn which cuts extended AQ at point R.

Proof: In ΔACR midpoint AC the is O and OP II CR.

\(O P=\frac{1}{2} C R\)

∴ ∠AOP = 90° ( ∵The diagonals of a square bisect each other at right angles.) and ∠AOP = ∠ACR = 90° (corresponding angles).

In ΔACR and ΔABQ,
∠ACR = ∠ABQ ( ∵ Each angle is a right angle)
∠CAR = ∠BAQ ( ∵ ∠BAC is the bisector of AQ)

Remaining ∠ARC= remaining ∠AQB
But ∠AQB VOA ∠CQR
∠CRQ = ∠CQR ( Each is equal to ∠AQB)
∴ CR = CQ

\(O P=\frac{1}{2} C R=\frac{1}{2} C Q\)

Ganit Prakash Class 9 Solutions

Question 15. Multiple Choice Question

1. In the triangle PQR, ∠PQR = 90° and PR = 10 cm. If S is the midpoint of PR, then the length of QS is

 

 

 

(1)4 cm
(2)5 cm
(3)6 cm
(4)3 cm

Solution: PR= 10 cm
We know that in a right-angled triangle the straight line from the vertex of the right angle to the midpoint of the hypotenuse is equal to half of the hypotenuse.

∴ \(\begin{aligned}
& Q S=\frac{1}{2} P R \\
& =\frac{1}{2} \times 10 \mathrm{~cm}
\end{aligned}\) = 5

∴ (2)5 cm.

2. In the trapezium ABCD, AB II DC and AB = 7 cm and DC = 5 cm. The mid-points of AD and BC are E and F respectively, the length of EF is

 

 

(1)5 cm
(2)6 cm
(3)7 cm
(4)12 cm

Solution: EF =1/2(AB+DC)

\(\begin{aligned}
& =\frac{1}{2}(7+5) \mathrm{cm} \\
& =\frac{1}{2} \times 12 \mathrm{~cm}=6 \mathrm{~cm}
\end{aligned}\)

Ganit Prakash Class 9 Solutions

∴ 2. 6cm

3. In the triangle ABC, E is the mid-point of the median AD; the extended BE intersects AC at the point F. If AC = 10.5 cm then the length of AF is

 

 

(1)3 cm
(2)3.5 cm
(3)2.5 cm
(4)5 cm

Solution: In triangle ABC the midpoint of median AD is E. The extended part of BE AC cuts at F. From point D parallel to BF a straight line is drawn which cuts AC at G point.

\(\begin{aligned}
& A F=\frac{1}{3} A C=\frac{1}{3} \times 10.5 \mathrm{~cm} \\
& =3.5 \mathrm{~cm}
\end{aligned}\)

∴(2) 3.5 cm

4. In the triangle ABC, the mid-points of BC, CA, and AB are D, E, and F respectively; 3E and DF intersect at the point X and CF and DE intersect at the point Y, the length of XY is equal to

 

 

(1)1/2 BC
(2)1/4 BC
(3)1/3 BC
(4)1/8 BC

Solution: E and F are joined.

∴ \(\mathrm{FE}=\frac{1}{2} \mathrm{BC}\) and FE II BC, i.e., FE II BD

∴ ED II AB, i.e., ED II FB
∴ FEDB is a parallelologram.
∴ Diagonals of a paralelogram bisect each other.
∴ BE and DF bisect each other at point X.
∴  X is the midpoint of FD
∴ Y, is the midpoint of the ED

\(X Y=\frac{1}{2} F E=\frac{1}{2} \cdot \frac{1}{2} B C=\frac{1}{4} B C\)

∴ (2)1/4 BC

Ganit Prakash Class 9 Solutions

5. In the parallelogram ABCD, E is the mid-point of the side BC; DE and extended AB meet at point F. The length of AF is equal to

 

 

 

(1) 3/2 AB
(2)2AB
(3)3AB
(4)5/4 AB

Solution: ΔDEC and ΔBEF
CE = BE ( ∵E, is the midpoint of BC)
∠CED = VOA ∠BEF and ∠DCE = alternate ∠EBF
∴ΔDEC ≅ ΔBEF (A-A-S congruency)
∴ DC = BF
∴ AF = AB+ BF =AB+ DC ( ∵BF = DC)
= AB+ AB (∵DC = AB)
= 2AB

∴ (2)2AB

Question 16. Short answer type questions

1. In the triangle ABC, AD and BE are two medians, and DF parallel to BE, meets AC at point F. If the length of the side AC is 8 cm, then let us write the length of the side CF.

 

 

Solution: In ΔABC, AD, and BE are medians.
∴ D and E are the midpoints of BC and AC respectively.

∴ \(C E=\frac{1}{2} A C\)

In ΔBCE the midpoint of BC is D and DF II BE.
∴ F, is the midpoint of CE

\(\begin{aligned}
& therefore C F=\frac{1}{2} C E=\frac{1}{2} \times \frac{1}{2} A C \\
& =\frac{1}{4} \times 8 \mathrm{~cm}=2 \mathrm{~cm}
\end{aligned}\)

 

2. In the triangle ABC, the mid-points of BC, CA, and AB are P, Q, and R respectively; if AC = 21 cm, BC= 29 cm, and AB = 30 cm, then let us write the perimeter of the quadrilateral ARPQ.

 

 

 

Solution: Q, is the midpoint of AC

∴ \(A Q=\frac{1}{2} A C=\frac{1}{2} \times 21 \mathrm{~cm}\) = 10.5 cm

∵ R is the midpoint of AB

∴ \(A R=\frac{1}{2} A B=\frac{1}{2} \cdot 30 \mathrm{~cm}\)= 15 cm

In ΔABC the midpoints of sides BC and AB are P and R respectively.

\(P R=\frac{1}{2} A C=\frac{1}{2} \times 21 \mathrm{~cm}=10.5 \mathrm{~cm}\)

Similarly,\(P Q=\frac{1}{2} A B=\frac{1}{2} \times 30 \mathrm{~cm}=15 \mathrm{~cm}\)

Perimeter of quadrilateral ARPQ = AQ + PQ+ PR + AR=(10.5+15+ 10.5+15) cm = 51 cm

3. In the triangle ABC, D is any point on the side AC. The mid-points of AB, BC, AD, and DC are P, Q, X, Y respectively. If PX = 5 cm, then let us write the length of the side QY.

 

 

Solution: B, and D are joined.
In ΔABD the midpoints of AB are ads are P and X respectively.

\(P X=\frac{1}{2} B D\)

∴ BD = 2PX = 2 x 5 cm = 10 cm

In ΔBCD the midpoints of BC and CD are Q and Y respectively.

\(Q Y=\frac{1}{2} B D=\frac{1}{2} \times 10 \mathrm{~cm}=5 \mathrm{~cm}\)

 

4. In the triangle ABC, the medians BE and CF intersect at point G. The mid-points of BG and CG are P and Q respectively. If PQ is 3 cm, then let us write the length of BC.

 

 

Solution: In ΔGBC the midpoints of BG and CG are P and Q respectively.

\(P Q=\frac{1}{2} B C\)

∴ BC= 2PQ = 2 x 3 cm = 6 cm

5. In the triangle ABC, the mid-points of BC, CA, and AB are D, E, and F respectively; FE intersects AD at the point O. If AD = 6 cm, let us write the length of AO.

 

 

Solution: D, E, and DF are joined.
In □AEDF, AF II ED, and FD II AE.
∴ AEDF is a parallelogram.

∵ Diagonals of a parallelogram bisect each other.
∴ AO = OD
∴O, is the midpoint  of AD

∴ \(A O=\frac{1}{2} A D=\frac{1}{2} \times 6 \mathrm{~cm}=3 \mathrm{~cm}\)