WBBSE Class 10 Physical Science Chapter 5 Light Long Answer Questions

Physics Class 10 WBBSE Chapter 5 Light Broad Answer Type Questions

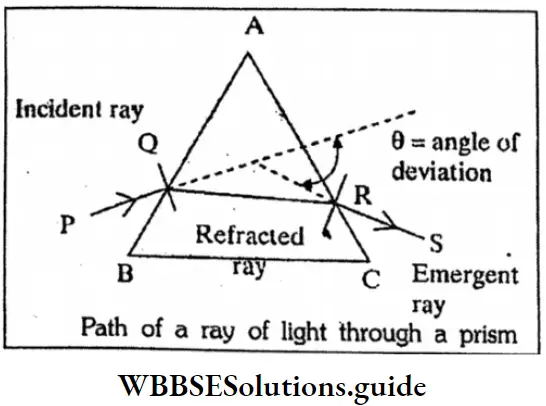
Question 1. Draw a labelled diagram to show refraction of a light ray through a prism.
Or
Path of a ray of light through a prism
Answer:

Path of a ray of light through a prism:

• Path of a ray of light through a prism Let ABC be the principal section of a prism AB and AC are two refracting surfaces. BC is the base. PQRS is the path of a ray. It is to be noticed that in i a prism.
• The refracted ray and the emer- gent ray bend towards the base of the prism, when the surrounding medium is optically rarer than the material of the prisms.
• But, if the medium outside the prism be optically denser than the prism material, the refracted and emergent rays bend oppositely i.e they bend towards the angle of the prism.

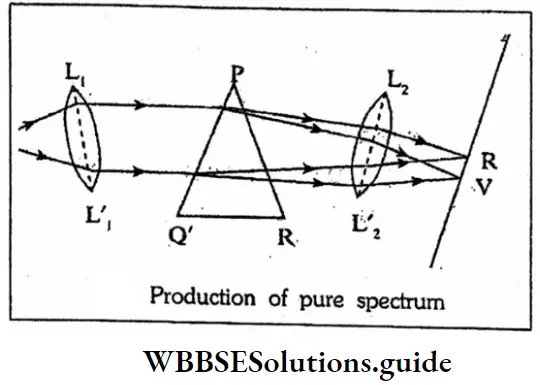
Question 2. What are the conditions of formation of pure spectrum?
Or
Production of pure spectrum
Answer:

Conditions of formation of pure spectrum:

• Slit should be very narrow to allow, rays of light to pass as minimum as possible.
• The slit should be placed at the focus so that the emergent light rays may be Parallel.
• Prism should be placed at the minimum deviation position of spec- trum i.e. at the mean deviation of yellow spectrum.
• The second lens (L₂ L₂) should be placed in such a position so that the screen may be in the focal plane of the lens.

Question 3. How will you explain twinkling of stars?
Answer:

Twinkling of stars:

The light coming from stars is refracted continuously by different layers of the atmosphere before coming to us. Due to there repeated refractions, The apparent position of a star is different from its actual position. The temperature and density of air in the atmosphere continuously changing and so apparent position of the star also changes continuously. Due to this continuous change in the apparent position of a star, it appears to be twinkling.

Question 4. A ray of light shows no dispersion on emerging from a glass slab. Explain why?
Answer:

A ray of light shows no dispersion on emerging from a glass slab

A rectangular glass slab cut diagonally into two pieces behaves like a pair of prisms placed side by side in reverse order. So the light dispersed by the first prism, recombines by the second prism. Thus there is no dispersion of light in a rectangular glass slab, bulb it is only laterally displaced.

Question 5. State the laws of reflection.
Answer:

The laws of reflection:

• The incident ray, the refracted ray and the normal to the refracting Surface at the point of incidence lie in the same plane.
• 2. For a given pair of media and for a given colour of light, sine of the angle of incidence bears a constant ratio to the sine of the angle of refraction.
• Thus if i and r be the angles of incidence and of refraction respectively when the ray passes from medium 1 to medium 2.

⇒\(\frac{sini}{sin r}\) = constant

= 1^μ2

Physics Class 10 WBBSE Question 6. Deseribe about the action of periscope.
Answer:

The action of periscope

The mirror M, forms a virtual image I₁, of the object O and this image ₁, acts as virtual object-for the mirror M₂, which forms an image I₂, which is seen by the eye the final image F₂, is also virtual. Since light rays from the object are reflected by two mirrors, the lateral inversion caused by the first mirror is reversed by the second mirror. This males the final image to appear with on lateral invession.

Question 7. State the laws of refraction.
Or
What is snell’s law?
Answer: 

1. Laws of refraction: The incident ray, the reflected ray and the normal to the refracting. Surface at the paint of incidence lie in the same plane.
2. For a given pair of media and for a given colour of light, the sine of the angle of incidence bears a constant ratio in the sine of the angle of refraction.

Thus, it i and r be the angles of incidence and of refraction respectively when the ray passes from medium 1 to medium 2.

\(\frac{sini}{sinr}\)= Constant

= 1^μ2

The second law of refraction was devolped by W, Snell and is know a Snell’s law.

Question 8. Establish the relation between 1μ₂ and 2μ₁.
Answer:

The relation between 1μ₂ and 2μ₁

From Snell’s law:  sin¹/sin^r = 2μ₁

Where 2 u₁, is the R. I. of medium 1 w.r.t. medium combining equations we get

1μ₂×2μ₁= \(\frac{sin1}{sinr}\)×\(\frac{sinr}{sin1}\) = 1

Or

1μ₂X = 1/2μ₁

Thus R.I. of medium 2 w.r.t. medium 1 is the reciprocal of R.I of medium 1 w.r.t. medum 2

Physics Class 10 WBBSE Question 9. State generalised Snell’s law.
Answer:

Generalisation Of Snell’s law

If i₁ and i₂ be the angle of incidence and of refraction respectively then we have from Snell’s law

sini₁/sini₂ =1, μ₂

We can write

1, μ₂ =μ₂ /μ₁

⇒μ₂/μ₁

∴ sini₁/sini₂= μ₂/μ₁

Or,μ₁ sini₁ = μ₂ sini₂ → Generalised Snell’s Law

Question 10. How far from a concave mirror of radius 2m would you place the object to get an image magnified 3 times?
Answer:

Given m = ± 3 \(\frac{v}{u}\) i.e.u= 7.3u

For real image v = -3u, r = -2m and u is negetive.

So, from relation \(\frac{1}{v}\)+ \(\frac{1}{u}\)= \(\frac{2}{r}\)

Or, – \(\frac{1}{3u}\) – \(\frac{1}{u}\) = – \(\frac{2}{2}\)

For virtual image u =+ 3u, r = -2m and u is negative

So =-1 or, u= = 0.67m

= \(\frac{1}{3u}\) – \(\frac{1}{u}\) = – \(\frac{2}{2}\)  Or \(\frac{1-3}{3u}\) = -1

u= \(\frac{2}{3}\)

= 0.67 m

WBBSE Class 10 Physical Science Question Answer In English Question 11. A concave mirror forms an image of 20 cm high object on a screen placed 5m away from the mirror. The height of the image is 50 cm. Find the focal lenght of the mirror and the distance between mirror and the object.
Answer:

Given

concave mirror forms an image of 20 cm high object on a screen placed 5m away from the mirror. The height of the image is 50 cm.

size of object = 20cm.

Size of the image = 50cm.

u = -5m.

= \(\frac{-10}{7}\)

= −1.43m

And distance between the mirror and the object = 2m.

Question 12. Light falls from glass of refractive index 1.5 to air. Find the angle of incidence for which the angle of deviation is 90°.
Answer:

Light falls from glass of refractive index 1.5 to air.

Refractive index of glass is 1.5. So, the critical angle of glass to air medium is given by

⇒ − q= sin^-1(\(\frac{1}{u}\)) sin^-1(\(\frac{1}{1.5}\))

= 41.8°

So, reflection in the glas medium takes only when the angle of incidence exceeds  41.8°. Now the angle of deviation. Would be 90° when the angle between the incident ray and the reflected ray becomes 90° i.e. the angle of incidence be equal to 45°.

Question 13. Where should an object be placed from a converging lens of focal length 20cm, so as to obtain a real image of magnification 2 ? u =2, or, v = + 2u, f= + 20cm
Answer:

Given m =\(\frac{u}{u}\)= 2 or  , v= +2u, f= +20cm

∴ \(\frac{1}{v}\)– \(\frac{1}{u}\) = \(\frac{1}{f}\) or,

=  \(\frac{1}{2u}\) – \(\frac{1}{u}\)

= \(\frac{1}{20}\) or,

= \(\frac{1-2}{2u}\)

∴ u= \(\frac{− 20×1}{2}\)

= – 10 cm

i.e. object to be placed 10 cm in fornt of the lens.

WBBSE Class 10 Physical Science Question Answer In English

Question 14. Two thin lens of focal lengths 15cm. and 30cm. respectively are kept in contact with each other. What is the power of the combined system?
=
Answer: Given

Two thin lens of focal lengths 15cm. and 30cm. respectively are kept in contact with each other.

f₁= 15cm, f₂ = 30cm.

Then focal length F of the equivalent lens

\(\frac{1}{F}\)= 1/f₁+1/f₂

\(\frac{1}{15}\) + \(\frac{1}{30}\)

= \(\frac{2+1}{30}\)

= \(\frac{1}{10}\)

Power of the combination = \(\frac{100}{F}\)

= \(\frac{100}{10}\)

= +10

Question 15. The dew drops deposited on hairy leaves appear glittering way?
Answer:

The hairs out the leaf and the surface tension of water create an air gap under the dew drop. Hence some of the rays trying to pass from the drop to the side air gap suffer total internal reflection. Such reflected rays may reach our eyes after refraction through the upper surface of the drop and the drop appears glittering.

16. Show that, for a spherical mirror the focal length is half of its radius of curvature.
Answer:

P = Pole

F= Focus of the mirror.

PF = f

C = Centre of curvature.

∠BP’C = ∠P’CF (alternate angles.) and

∠BP’C = ∠CP’F (law of reflections i = r)

Hence ∠’P’CF = ∠CP’F

ΔFP’ C is isosceles. Hence P’F = FC

If the aperture of the mirror is small, the point P i very close to the point P, then P’F = PF

∴ PF=  FC or, PF + PF = PF + FC

or , 2PF = PC or, PF =  ½ PC

or, f = ½ R.

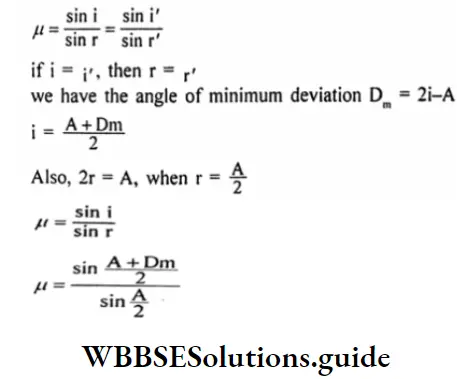
Question 17. Establish relation between the angle of deviation (Dm) and the refractive index of the material of the prism (μ).
Answer:

Let, the angle of deviation be D, the refractive index of the material of the prism be μ.

For minimum deviation, we have ¡=i’

Question 18.

1. Write Cauchy’s equation 
2. Define-angular dispersion.

Answer:

1. The Cauchy’s equation: = А+B/ λ2

A, B = Cauchy’s constant

2. The angular dispersion is defined as the difference between the angles of deviation of the extreme colours, red and violet of the spectrum.

WBBSE Class 10 Physical Science Question Answer In English

Question 19. 

1. What is the condition for dispersion with deviation and deviation without dispersion?
2. What is the pure and impure spectrum?

Answer:

1. Condition for dispersion with deviation

⇒(μ_v-1)A = (μ’_y-1)A’

The condition for devotion without dispersion :

⇒ δ_y -δ_r (μ_y-1)A(w-w’)

2. 

• Pure spectrum: A spectrum in which the colours present do not overlap one another so that different colours occupy distinct and separate positions is called pure spectrum.
• Impure spectrum: A spectrum in which the colours present overlap one another and hence can not be seen distinctly separated from one another is called an impure spectrum.

Question 20. Light of two colours (say X, Y) are sent through a prism. Suppose X bents more than Y. Which colour travels more slowly in the material of the prism?
Answer:

Light of two colours (say X, Y) are sent through a prism. Suppose X bents more than Y.

We know, D, (μ_x-1)A

D1 = (μ_y-1)A

⇒ D_y>D_y

⇒ μ _x> μ_y

C/ V_x>C/ V_y

∴ V_y > V_x

The colour X travels more slowly in the material of the prism.

Question 21. Draw a ray diagram for the formation of a concave mirror when the object is at focus F.
Answer:

AB is an object placed at the focus F. The ray AD is incident on the mirror parallel axis and it gets reflected along DF, passing through the focus f. AE appears to be coming from the centre of curvature C, so it gets reflected back along EA. The two reflected rays DF and EA are parallel to each other.

The image is formed at infinity (very far from the mirror) which is real, inverted and highly magnified.

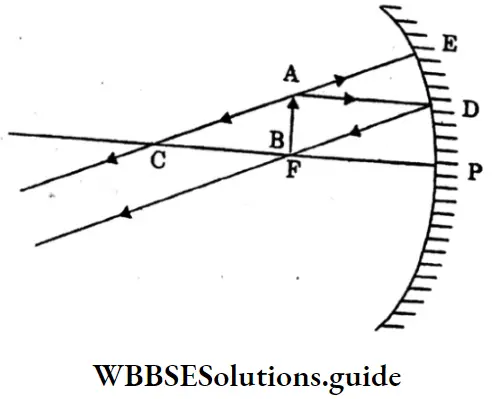
When the object is at focus F.

Thus when the object is at the focus F, the image is at infinity. It is

1.  Real
2. Inverted, and
3. Highly magnified.

Question 22. How the angle of minimum deviation of a glass prism changes when it is immersed in a liquid of R.I. greater than I? sin A+D m
Answer:

Minimum deviation of a glass prism:

Hence the angle of minimum deviation decreases.

Question 23. Define-Absorption Spectrum.
Answer:

Absorption Spectrum:

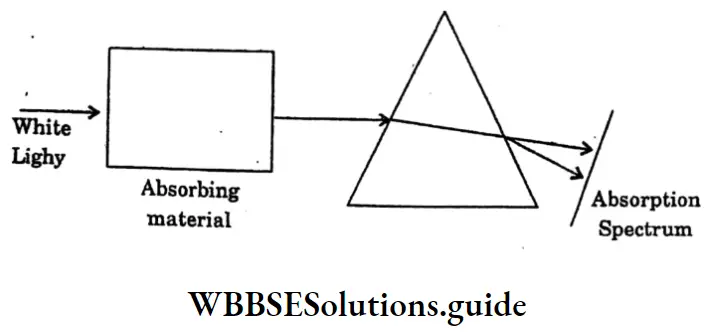
The missing wavelengths provide information about the absorbing material. Such a spectrum is called an absorption spectrum. The absorption spectrum may be of two types depending on the absorbing material and the conditions such as temperature.

Question 24 . An object 4 cm. high is placed at a distance of 10cm from a convex lens of focal length 20cm. Find the position, nature and size of the image.
Answer:

An object 4 cm. high is placed at a distance of 10cm from a convex lens of focal length 20cm.

O= 4cm, u=-10 cm, f= + 20cm.

\(\frac{1}{V}\) = \(\frac{1}{f}\) +\(\frac{1}{u}\)

= \(\frac{1}{20}\) – \(\frac{1}{10}\)

= – \(\frac{1}{20}\)

V= -20 cm

\(\frac{1}{o}\) – \(\frac{V}{u}\)

I = \(\frac{V}{u}\) × 4

= \(\frac{-20}{-10}\) ×4

= 8cm

Question 25. Two lenses one of focal length 20 cm. and another focal length -15cm, are placed in contact. What is the focal length and power of the combination?
Answer:

Two lenses one of focal length 20 cm. and another focal length -15cm, are placed in contact.

f, 20cm=0.2m f15cm – 0.15m

Power of the first lens, P₁ = \(\frac{1}{0.2}\) = 5D

Power of the second lens, P₂ = \(\frac{-1}{0.2}\)

= 6.67 D

Power of combination, P = P₁ + P₂ = 5 – 6.67 = -1.67D

Focal length, f= \(\frac{1}{1.67}\)  0.599m

The -ve sign indicates that the combination acts as a concave lens.

Question 26. An object 4 cm. high is placed at a distance of 10cm from a convex lens of focal length 20cm. Find the position nature and size of the image.
Answer:

An object 4 cm. high is placed at a distance of 10cm from a convex lens of focal length 20cm.

0=  4cm, u = -10cm, f= + 20cm,

\(\frac{1}{V}\) =\(\frac{1}{F}\)+ \(\frac{1}{u}\)

= \(\frac{1}{20}\) – \(\frac{1}{10}\)

= – \(\frac{1}{20}\)

V= – 20 cm

\(\frac{I}{O}\)= \(\frac{V}{u}\) or, \(\frac{V}{u}\)×0

= \(\frac{-20}{-10}\)×4

= 8cm