West Bengal Board Class 10 Math Book Solution In English Chapter 18 Similarity Exercise 18.2
Question 1. A line parallel to the side BC of AABC intersects the sides AB and AC at points P and Q respectively.
1. If PB AQ, AP = 9 units, QC = 4 units, then let us calculate the length of PB.
Solution:
Given
PB = AQ, AP = 9 unit, QC = 4 unit, PB.
As PQ || BC
AP/BP = AQ/QC
9/ AQ = AQ/4
∴AQ² = 36
∴ AQ= 6 unit
∴ Length of PB = 6 units.
Read and Learn More WBBSE Solutions For Class 10 Maths
2. The length of PB is twice of AB and the length of QC is 3 units more than the length of AQ, then let us write by calculating the length of AC.
Solution:
Given
The length of PB is twice of AB and the length of QC is 3 units more than the length of AQ,
PB = 2AQ,
QC = AQ + 3
AP/2AP = AQ/AQ+3
or, 1/2 = AQ/AQ+3
2AQ = AQ +3
∴ AQ = 3 unit
∴ QC 3+3 = 6 unit
∴ Length AC AQ + QC = 3 +6 = 9 unit.

3. If AP QC, the length of AB is 12 units and the length of AQ is 2 units, then let us calculate the length of CQ.
Solution:
Given, AP QC, AB = 12 units, AQ= 2 units.
Now, AP/ PB = AQ /QC
QC/PB = 2/QC
or, QC/AB – AP = 2/QC
or, QC² = 24 – 2QC
or, QC²+2QC – 24 = 0
or, QC²+6QC-4QC-24 = 0
or, QC(QC+6)-4(QC +6) = 0
or, (QC+6)(QC-4) = 4
∴ QC-6, QC = +4.
As length cannot be negative.
∴ CQ = 4 units.
Question 2. I took two points X and Y on the sides PQ and PR respectively of APQR.
1. If PX = 2 units, XQ = 3.5 units, YR = 7 units, and PY = 4.25 units, then let us write with reason whether XY and QR will be parallel to each other or not.
Solution:
Given
If PX = 2 units, XQ = 3.5 units, YR = 7 units, and PY = 4.25 units,
Join X, Y.
Now, XY || QR if PX/XQ = PY/YR
Here given, PX = 2 unit, XQ = 3.5 unit
YR = 7 unit, & PY = 4.25 unit.
PX/XQ = 4.25 x 100 / 7 x 100
= 425/700
= 17/28
∴ PX/XQ =not PY/YR
∴ XY is not parallel to QR.
2. If PQ = 8 units, YR = 12 units, PY = 4 units and the length of PY is less by 2 units than the length of XQ, then let us write with reason whether XY and QR will be parallel or not.
Solution:
Given, PQ = 8 unit; YR = 12 unit, PY = 4 unit & PY = XQ – 2.
∴ XQ = PY + 2 = (4+2) unit = 6 unit.
Now, PX = PQ-XQ
= 8-6
= 2 unit
∴ PX/XQ = 2/6
= 1/3
& PY/YR = 4/12
= 1/3
∴ PX/XQ = PY/YR
∴ XY || QR.
3. Let us prove that the line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. [Let us prove it with the help of Thales theorem.]
Solution:
Let in ΔABC, a straight line is drawn from P, the midpoint of AB, parallel to BC cuts AC at Q.
To prove Q is the midpoint of AC, i.e., AQ = QC.
Proof: In ΔABC, PQ is parallel to BC.
AP/PQ = AQ/QC but APPQ (as P is the midpoint of AB)
PQ/PQ = AQ/QC = 1/1
∴ AQ = QC
i.e., Q is the midpoint of AC.
4. P is a point in the median AD of AABC. The extended BP and CP intersect AC and AB at points Q and R respectively. Let us prove that RQ || BC.
Solution:
Given
P is a point in the median AD of AABC. The extended BP and CP intersect AC and AB at points Q and R respectively.
In ΔABC, AD is a median.
∴ ΔABD = ΔACD, again in ABPC, PD is the median.
∴ ΔBPD = ΔCPD
ΔABD – ΔBPD = ΔACD- ΔCPD
i.e., ΔABP = ΔACP
Now as P is a point on AD.
∴ ΔARP = ΔAQP
∴ ΔABP – ΔQRP = ΔACP – AAQP
ΔARP = ΔQPC
Now,ΔARP/ΔRPB = AR/RB
& ΔAQP/ΔQPC = AQ/QC
∴ AR/RB = AQ/QC i.e., RQ cut AB & AC of ΔABC proportionately.
∴ RQ || BC. Proved.

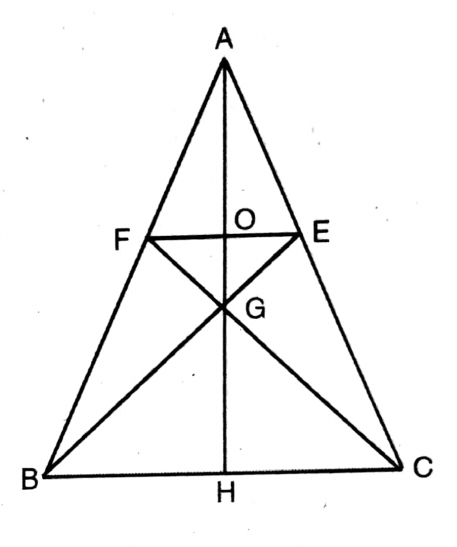
5. The two medians BE and CF of AABC intersect each other at point E and if the line segment FE intersects the line segment AG at point O, then let us prove that AO 3 OG.
Solution:
Given
The two medians BE and CF of AABC intersect each other at point E and if the line segment FE intersects the line segment AG at point O
Produced AG which cuts BC at H.
Proof: InΔABC, F is the midpoint of AB
& E is the midpoint of AC.
∴ ΔEF || BC & in ΔABH, FO || BH
G is the centroid of AABC.
∴ AG: GH = 2:1
or, AG/GH 2/1 or AO+OG / GH = 2/1
Again, GH = 1/3 or, AO+OG/ 1/3 AH = 2
or, AO + OG = ⅔ AH
Or, AO + OG = 2/3 X 2 OA[ AO = OH]
=4/3 OA
OG = 4/3 OA-OA
= 1/3 OA
∴ OA = 3OG Proved.
6. Let us prove that the line segment joining the mid-point of two transverse sides of a trapezium is parallel to its parallel sides.
Solution: Let in the trapezium ABCD, P & Q are the midpoints of the two slant sides AD & BC.
To prove AB || PQ or PQ || DC.
Side DA & CB is produced & they meet at R.
Proof: In trapezium ABCD, AB || CD
∴ From ΔRDC, RA/ ½ AD
or, RA/AP = RB/BQ
∴ In ΔRPQ, AB || PQ
From (1) RA/AD +1 = RB/BC + 1
RA+AD / AD = RB+BC / BC
or, RD/AD = RC/BC
or, RD/½ AD = RC/½ BC
∴ RD/PD =DRC/QC
∴ From ARDC, PQ II DC. Proved.
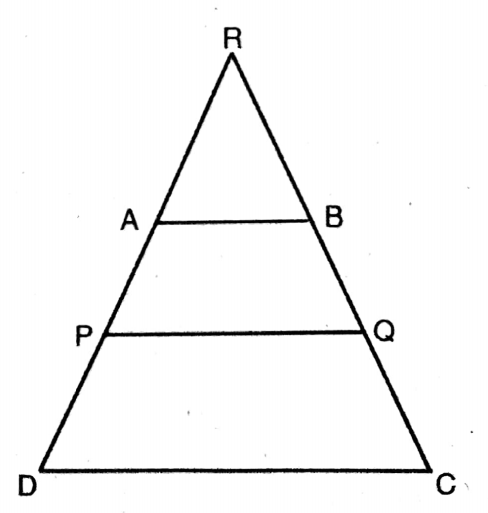
7. D is any point on the side BC of AABC. P and Q are centroids of AABD and AADC respectively. Let us prove that PQ || BC.
Solution:
Given
D is any point on the side BC of AABC. P and Q are centroids of AABD and AADC respectively.
Join DP & DQ+ produced, they cut AB & AC at M & N points respectively. Proof The line DM passing through P, the centroid of ΔABD.
∴ DM is a median.
M is the midpoint of AB.
Similarly, from AADC N is the midpoint of AC
of ΔADC.
Now in ΔABC, M & N are the midpoints of the sides AB & AC of ΔABC.
∴ MN || BC
Now, from ΔDMN, DP/PM = 2/1 = DQ/QN
i,e., DP/PM = DQ/QN
∴ PQ || MN
Now, MN || BC & PQ ||MN
∴ BC || PQ,
i.e., PQ || BC.
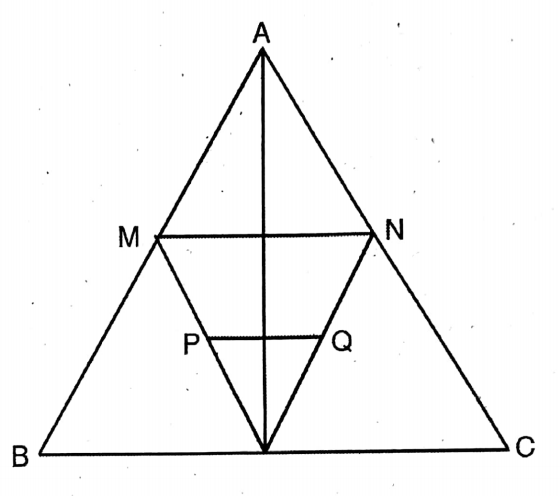
8. I have drawn two triangles APQR and ASQR on the same base QR and on the same side of QR and their areas are equal. If F and G are two centroids of two triangles, then let us prove that, FG || QR.
Solution:
Join P, S. Produce PE & SG. They meet at O on QR.
Proof: As ΔPQR & ΔSQR are on the same side of base QR, & their areas are equal.
∴ ΔPQR & ΔSQR are in between two parallel lines PS & QR.
∴ PS || QR
Now, OP is a median of APQR & OS is a median of ASQR.
OF: FP = 1:2
or, OG/GS = 1/2
∴ OF/FP = OG/GS
∴ FG cuts OP & OS of ΔPOS, proportionately
∴ FG|| PS.
Now PS || QR & FG || PS
∴ FG || QR.

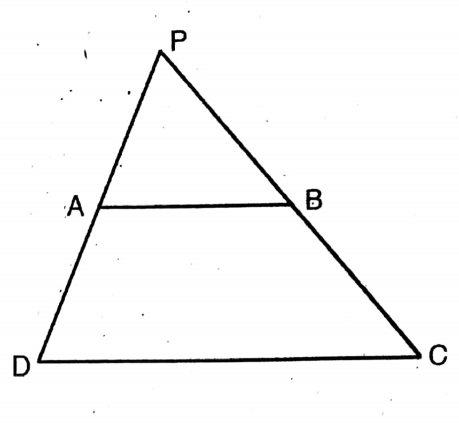
9. Let us prove that the two adjacent angles of any parallel side of a trapezium are equal.
Solution: Let ABCD is an isosceles trapezium whose
AD = BC & AB || DC.
To prove ∠DAB = <CBA.
Produced DA & CB of the trapezium ABCD: They meet at P.
Proof: Now, in ΔPDC, the straight line || to DC cuts PD & PC at A & B
As AB cuts DP & CP internally,
∴ PA/AD = PB/BC or, PA/AD = PB/AD
∴ PA = PB
Now, in ΔPAB, PA = PB, ∴ PA = PB
Now, ∠DAB = 180° – PAB &
∠CBA = 180° – ∠PBA
∴ ∠DAB = ∠CBA Proved.
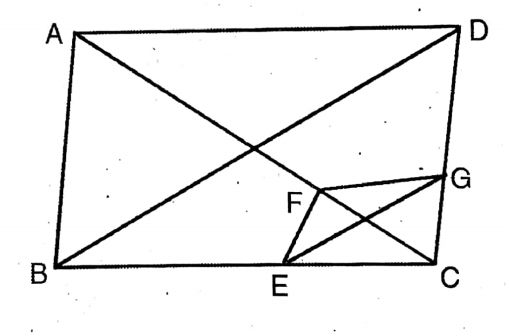
10. AABC and ADBC are situated on the same base BC and on the same side of BC. E is any point on the side of BC. A line through point E and parallel to AB and BD intersect the sides AC and DC at points F and G respectively. Let us prove that, AD || FG.
Solution:
Given
AABC and ADBC are situated on the same base BC and on the same side of BC. E is any point on the side of BC. A line through point E and parallel to AB and BD intersect the sides AC and DC at points F and G respectively.
Join A, D
Proof: From ΔABC, AB|| EF
∴ FC/FA = EC/FA———-(1)
&from (1) &(2), FC/FA= CG/DG
Again, from ΔADC, FC/FA = CG/DG
∴ FG||AD Proved
Class 10 WBBSE Math Solution In English Chapter 18 Similarity Exercise 18.2 Multiple Choice Question
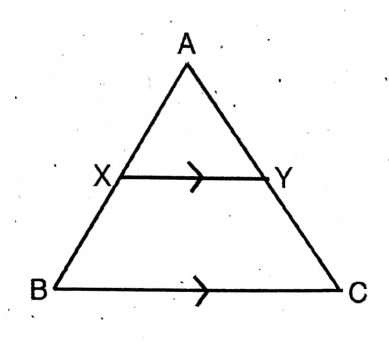
Question 1. A line parallel to the side BC of AABC intersects the sides AB and AC at points X and Y respectively. If AX = 2.4cm, AY 3.2cm and YC = 4.8cm, then the length of AB is
1. 3.6 cm.
2. 6 cm.
3. 6.4 cm.
4. 7.2 cm.
Solution:
Given
A line parallel to the side BC of AABC intersects the sides AB and AC at points X and Y respectively. If AX = 2.4cm, AY 3.2cm and YC = 4.8cm,
AB = 6 cm as AX/AB = AY/AC
Or, 2.4/AB = 32 / 3.2 + 4.8
∴ AB = 2.4 X 8/ 3.2
= 6
Answer. 2. 6 cm.
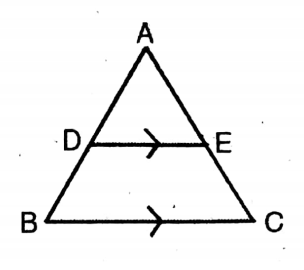
Question 2. The points D and E are situated on the sides AB and AC of AABC in such a way that DE || BC and AD: DB = 3: 1; if EA = 3.3 cm, then the length of AC is
1. 1.1 cm.
2. 4 cm.
3. 4.4 cm.
4. 5.5 cm.
Solution:
Given
The points D and E are situated on the sides AB and AC of AABC in such a way that DE || BC and AD: DB = 3: 1; if EA = 3.3 cm
AD/DB = AE/EC or, 3/1 = 3.3/EC
∴ EC = 1.1
∴ AC = AE +EC
= 3.3 + 1.1
=4.4
.. AC = 4.4 CM.
The length of AC is 4.4 CM.
Answer. 3. 4.4 CM.
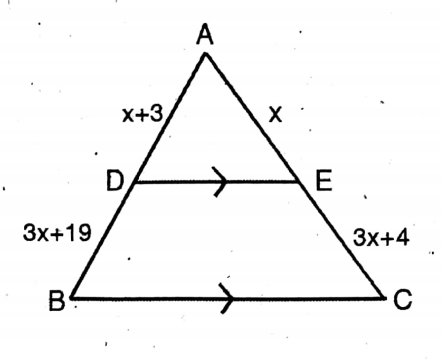
Question 3. In the adjoining figure if DE || BC, then the value of x is
1. 4
2. 1
3. 3
4. 2
Solution:
Given
In the adjoining figure if DE || BC
AD/DB = AE/EC
x+3 / 3x+19 = x / 3x +4
or, 3×2 + 19x = 3×2 + 13x + 12
or, 6x = 12
∴ x=2
Answer: 4. 2
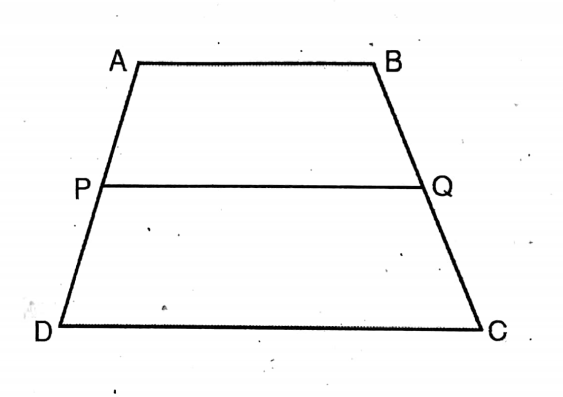
Question 4. In the trapezium, ABCD, AB || DC, and the two points are situated on the sides AD and BC in such a way that PQ || DC; if PD = 18 cm, BQ = 35 cm, QC = 15 cm, then the length of AD is
1. 60 cm.
2. 30 cm.
3. 12 cm.
4. 15 cm.
Solution:
Given
In the trapezium, ABCD, AB || DC, and the two points are situated on the sides AD and BC in such a way that PQ || DC; if PD = 18 cm, BQ = 35 cm, QC = 15 cm
AP/PD = BQ/QC
or, AP/18 = 35/15
∴ AD = AP + PD
= 42+18
= 60 cm.
The length of AD is 60 cm.
Answer: 1. 60 cm.
Question 5. In the adjoining figure, if DP = 5 cm, DE = 15 cm, DQ = 6 cm, and QF = 18 cm, then
1. PQ = EF
2. PQ || EF
3. PQ ≠ EF
4. PQ ∦ EFDP
Solution:
Given
In the adjoining figure, if DP = 5 cm, DE = 15 cm, DQ = 6 cm, and QF = 18 cm
DP/DE = 5/15 = 1/3
& DQ/DP = 6/ DQ+QF
= 6/ 6+18
=6/24
=1/4
∴ DP/DE ≠ DQ/DP
∴ PQ ∦ E
Answer: PQ ∦ EF
WBBSE Solutions Guide Class 10 Chapter 18 Similarity Exercise 18.2 True Or False
1. Two similar triangles are always congruent.
False
2. In the adjoining figure, if DE || BC, then/BD = BD/CE
True
Chapter 18 Similarity Exercise 18.2 Fill In The Blanks
1. The line segment parallel to any side of a triangle divides the other two sides of the extended two sides Proportional.
2. If the bases of two triangles are situated on the same line and the other vertex of the two commons, then the ratio of the areas of two triangles is to the Equal ratio of their bases.
3. The straight lines parallel to the parallel sides of a trapezium divide the Proportional other two sides.
WBBSE Class 10 Maths Solutions Chapter 18 Similarity Exercise 18.2 Short Answers
Question 1. In the adjoining figure, if in ABC, AB/DB = AE/EC and ZADE = ZACB, then let us write the type of the triangle according to the side AD = AE
Solution.
Given
In the adjoining figure, if in ABC, AB/DB = AE/EC and ZADE = ZACB,
As in the ΔABC, AD/DB = AE/EC ..DE || BC
∴ ∠ADE = ∠ABC
Again, ∠ADE = ∠ACB
∴ ∠ABC = ∠ACB
∴ ΔABC is an isosceles triangle.
Question 2. In the adjoining figure if DE || BC and AD: BD = 3:5, then let us write the ratio of the area of ΔADE: area of ΔCDE.
Solution.
Given
In the adjoining figure if DE || BC and AD: BD = 3:5
As DE || BC,
∴ AD/BD = AE/CE
∴ AD/BD = 3/5
∴ AE/CE = 3/5
Join C, D. Perpendicular drawn from D, on AC, cuts AC at F
Let DF = h.
Now the area of AADE = Area of ACDE
= 1/2 AE x h: 1/2 CF x h
∴ AE: CE 3:5
Question 3. In the adjoining figure, if LM || AB and AL = (x-3) unit, AC = 2x unit, BM = (x-2) unit and BC = (2x + 3) unit, then let us determine the value of x.
Solution.
Given
In the adjoining figure, if LM || AB and AL = (x-3) unit, AC = 2x unit, BM = (x-2) unit and BC = (2x + 3) unit,
As LN || AB,
∴ AC/AL = BC/BM
or, 2x / x-3 = 2x+3 / (x-2)
or, 2x(x-2) = (2x+3)(x-3)
or, 2×2-4x = 2×2-3x-3
or,-4x+3x = -9
or, -x = -9
∴ x = 9

Question 4. In the adjoining figure, if in AABC, DE || PQ || BC and AD = 3 cm, DP = x cm, AE = 4 cm, EQ = 5 cm, QC = y cm, then let us determine the value of x and y. Ans. In the DE || PQ
Solution:
Given
In the adjoining figure, if in AABC, DE || PQ || BC and AD = 3 cm, DP = x cm, AE = 4 cm, EQ = 5 cm, QC = y cm
In the DE || PQ
∴ AD/PD = AE/QE
or, 3/x = 4/5 ∴ x = 15/4
Again ,PQ/BP = AQ/CQ
or, AD+PD = AQ/CQ
AD+PD / BP = AE+EQ / CQ
or, 3+ 15/4 / 4
= 4+5 / y
or, 27/16 = 9/y
∴y = 16/3
∴ x = 15/4 , y = 16/3
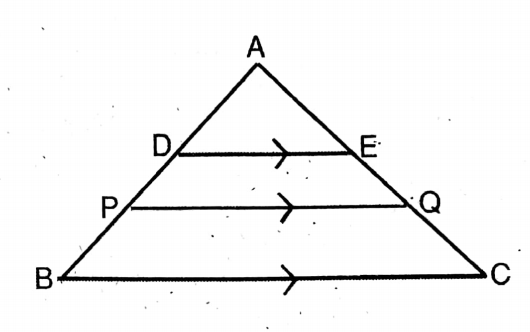
Question 5. In the adjoining figure, if DE || BC, BE || XC, and AD/DB = 2/1, then let us determine the value of AX/XB.
Solution:
Given
In the DE || BC
.. AD/DB = AE EC = 2/1
in ΔXC, BE || X C
∴ AB/XB = AE/CE
or, AB/XB +1 = AE/CE +1
or, AB+XB / XB = 2/1 +1
AX/AB = 3/1
∴ AX/XB = 3.
Question 3.
1. In the same way, like the following. (II) and (III), by putting AABC on APQR, I am observing that ∠B = ![]() and ∠C=
and ∠C= ![]()
Ans. ∠Q and ∠C.
2. If the sides of the triangles are proportional then they are similar.
Solution: In two triangles, ΔABC & ΔPQR sides are proportional.
i.e., AB/PQ = AC/PR = BC/QR
To prove that the triangles are similar.
ΔABC, Coincide on ΔPQR such that ∠A falls on ∠P.
i.e., ∠A = ∠P
Similarly, ∠C falls on ∠R & ∠B falls on ∠Q.
∠B = ∠Q Proved.
3. We have understood that if two angles of one triangle are equal to two angles of another triangle, then their corresponding sides are proportional. Let me write by understanding the reason.
Solution: If the two triangles are equiangular,
then their corresponding sides are proportional.
The angles B & C of ΔABC are equal to the angles E & F of ΔDEF.
∴ AB/DC = BC/EF = AC/DF
4. Like the above figure, I put ABC on DEF in such a way that vertex A and vertex D overlap with each other and side DE remains on side AB.
Solution: I am observing that
1. the side AC overlaps with the side DF [ ∠A = ∠D],
and 2. BC/EF = 1.6 /2.6
Application 1. Let us see the adjoining figure and write the value of P by calculating it.
Solution: In ΔABC and ΔPQR,
AB/PR = 5/10
= 1/2
BC/PQ = 4/8
= 1/2
AC/QR = 7/14
= 1/2
∴ AB/RP = BC/PQ = CA/QR = 1/2
ΔABC and ΔRPQ are similar>
∴ ∠A = ∠A , ∠A = ∠P and ∠C = ∠Q
∴ ∠P = ∠ B = 85º

Application 2. The perimeters of two similar triangles are 20 m and 16 cm respectively, if the length of one side of first triangle is 9 cm, then let us write by calculating, the length of the corresponding side of the second triangle. The perimeter of 1st triangle
Solution:
Given
The perimeters of two similar triangles are 20 m and 16 cm respectively, if the length of one side of first triangle is 9 cm, then let us write by calculating, the length of the corresponding side of the second triangle.
We know the Perimeter of the 2nd triangle/Corresponding side of the 2nd triangle = One side of the 1st triangle /Corresponding side of the 2nd triangle
or, 20cm/16cm = 9cm/Corresponding side of 2nd triangle
5/4 = 9/Length of corresponding side of 2nd triangle
∴ Length of corresponding side of 2nd triangle = 9 x 4 / 5
= 36/5
= 7.2 cm.
Application 3. In AABC, B = C, the points D and E are situated on BA and CA in such a way that BD = CE; let us prove that DE || BC.
Solution:
Given
In AABC, B = C, the points D and E are situated on BA and CA in such a way that BD = CE
To prove DE || BC.
∴ ABC, B = ∠ C ,
BD = CE
As ∠B = ∠C
∴ AB = AC,
or, AB/BD = AC/EC [as PB = EC]
∴ DE || BC Proved.
