WBBSE Solutions For Class 6 Maths Chapter 1 Fraction

Class 6 Math Solutions WBBSE Fraction

Question 1. Let us find the value of (40 ÷ 5) ÷ 4 (5 – 3) If there is no ‘sign’ after a number, it means multiplication
Solution:

Given

(40 ÷ 5) ÷ 4 (5 – 3)

⇒ (40 ÷ 5) ÷ 4 (5 – 3) = 8 ÷ 4 of 2 (4 of 2 = 4 * 2)

⇒ (40 ÷ 5) ÷ 4 (5 – 3) = 8 + 8

⇒ (40 ÷ 5) ÷ 4 (5 – 3) = 1

Question 2. Let me find the values of {25 – (4 ÷ 9)} ÷ 3 and 25 – 4 + 9 ÷ 3
Solution:

Given

{25 – (4 + 9)} ÷ 3

⇒ {25 – (4 + 9)} ÷ 3 = {25-13}÷3

⇒ {25 – (4 + 9)} ÷ 3 = 12÷3

⇒ {25 – (4 + 9)} ÷ 3 = 4

and

Read and Learn More WBBSE Solutions For Class 6 Maths

25 – 4 + 9÷3

⇒ {25 – (4 + 9)} ÷ 3 = 25-4 + 3

⇒ {25 – (4 + 9)} ÷ 3 = 28-4

⇒ {25 – (4 + 9)} ÷ 3 = 24

Class 6 Maths Solutions WBBSE

Question 3. Shall we get same values for (16 – 4) (5 – 3) and 16 – 4(5 -3).
Solution:

Given

(16 – 4) (5 – 3)

⇒ (16 – 4) (5 – 3) = 12×2

⇒ (16 – 4) (5 – 3) = 24 and

16-4(5-3)

⇒ (16 – 4) (5 – 3) = 16 – 4 of 2

⇒ (16 – 4) (5 – 3) = 16-8

⇒ (16 – 4) (5 – 3) = 8

Question 4. Let me find the values for 10 – 3 – 5 and 20 ÷ 5 ÷ 2.
Solution:

Given

10 – 3 – 5

⇒ (10 – 3) – 5 = 7 – 5

⇒ 7 – 5 = 2 and

20 ÷ 5 ÷ 2

⇒ 20 ÷ 5 ÷ 2 = 4 ÷ 2 = 2

Class 6 Maths Solutions WBBSE Fraction Exercise 1.1

Question 1. Verify, if the values are same for all these cases

1. 20 + 8 ÷ (4 – 2)
Solution:

Given

20 + 8 ÷ (4 – 2)

⇒ 20 + 8 ÷ (4 – 2) = 20 + 8 ÷ 2

⇒ 20 + 8 ÷ (4 – 2) = 20 + 4

⇒ 20 + 8 ÷ (4 – 2) = 24

2. (20 + 8) ÷ (4 – 2)
Solution:

Given

(20 + 8) ÷ (4 – 2)

⇒ (20 + 8) ÷ (4 – 2) = (20 + 8) ÷ 2

⇒ (20 + 8) ÷ (4 – 2) = 28 ÷ 2

⇒ (20 + 8) ÷ (4 – 2) = 14

3. (20-8)(4-2)
Solution:

Given

⇒ (20 – 8) (4 – 2)

⇒ (20 – 8) (4 – 2) = 12 x 2

⇒ (20 – 8) (4 – 2) = 24

4. 20 – 8 (4 – 2)
Solution:

Given

20 – 8 (4 – 2)

⇒ 20 – 8 (4 – 2) = 20 – 8(2)

⇒ 20 – 8 (4 – 2) = 20 – 16 =4

5. (20 + 8) ÷ 4 – 2
Solution:

Given

(20 + 8) ÷ 4 – 2

⇒ (20 + 8) ÷ 4 – 2 = 28 ÷ 4 – 2

⇒ (20 + 8) ÷ 4 – 2 = 7 – 2 = 5

Class 6 Maths Solutions WBBSE

Question 2. Let us form similar simplification sums with numbers 12, 6, 3 and 1 and then find their values:

1. 12 + 6 ÷ (3 – 1)
Solution:

Given

⇒ 12 + 6 ÷ (3 – 1 ) = 12 + 6 ÷2

⇒ 12 + 6 ÷ (3 – 1 ) = 12 + 3

⇒ 12 + 6 ÷ (3 – 1 ) = 15

2. (12 + 6) ÷ (3 -1)
Solution:

Given

(12 + 6) ÷ (3 -1)

⇒ 12 + 6 ÷ (3 – 1 ) = 18 ÷ 2

⇒ 12 + 6 ÷ (3 – 1 ) = 9

3. (12-6) (3-1)
Solution:

Given

(12-6) (3-1)

⇒ (12 – 6 ) (3 – 1) = 6 x 2

⇒ (12 – 6 ) (3 – 1) = 15

4. 12 – 6(3 – 1)
Solution:

⇒ 12 – 6 (3 – 1) = 12 – 6 x 2 =12-12 = 0

5. (12 + 6) ÷ 3-1
Solution:

⇒ (12 + 6) ÷ (3 – 1) = 18 ÷ 3-1

⇒ (12 + 6) ÷ (3 – 1) = 6-1 = 5

Class 6 WBBSE Math Solutions

Question 3. Let us find the value of simplification sums:

1. \(256 \div \overline{16 \div 2} \div \overline{18 \div 9} \times 2\)
Solution:

⇒ \(256 \div \overline{16 \div 2} \div \overline{18 \div 9} \times 2\)

⇒ = 256 ÷ 8 ÷ 2 x 2

⇒ = 32 ÷ 2 x 2

⇒ = 16 x 2

⇒ = 32

2. (72 ÷ 8 x 9)- (72 ÷ 8 of 9)
Solution:

(72 ÷ 5- 8 x 9) – (72 ÷ 5- 8 of 9)

⇒ (72 ÷ 5- 8 x 9) – (72 ÷ 5- 8 of 9) = (9 x 9) – (72 ÷ 72)

⇒ (72 ÷ 5- 8 x 9) – (72 ÷ 5- 8 of 9) = 81-1

⇒ (72 ÷ 5- 8 x 9) – (72 ÷ 5- 8 of 9) = 80

(72 ÷ 5- 8 x 9) – (72 ÷ 5- 8 of 9) = 80

3. 76-4 -[6 + {19-(48- \(\overline{57-17}\)))]
Solution:

76-4 -[6 + {19-(48- \(\overline{57-17}\)))]

⇒ = 76- 4 [6+ {19 – (48 – 40)}]

⇒ = 76 – 4 – [6+{19 – 8}]

⇒ = 76-4-[6+11]

⇒ = 76-4-17 = 72-17 = 55

76-4 -[6 + {19-(48- \(\overline{57-17}\)))] = 55

4. {25 x 16 ÷ (60 ÷15) – 4 x (77 – 62)} ÷ (20 x 6 ÷ 3)
Solution:

⇒ {25 x 16 ÷ (60 ÷15) – 4 x (77 – 62)} ÷ (20 x 6 ÷ 3)

⇒ = {25 x 16 ÷ 4 – 4 x 15} ÷ (120 ÷ 3)

= {25 x 4 – 60} ÷ 40

= {100 – 60} ÷ 40

= 40 ÷ 40

= 1

{25 x 16 ÷ (60 ÷15) – 4 x (77 – 62)} ÷ (20 x 6 ÷ 3) = 1

5. [16 ÷ {42 – 38 + 2}] 12 ÷ (24 ÷ 6) x 2 + 4
Solution:

[16 ÷ {42 – 38 + 2}] 12 ÷ (24 ÷ 6) x 2 + 4

= [16 ÷ {42 – 40}] 12 ÷ 4 x 2 + 4

= [16 ÷ 2] 12 ÷ 4 x 2 + 4

= 8 of 12 ÷ 4 x 2 + 4

= 96 ÷ 4 x 2 + 4

= 24 x 2 + 4

= 48 + 4

= 52

[16 ÷ {42 – 38 + 2}] 12 ÷ (24 ÷ 6) x 2 + 4 = 52

Class 6 WBBSE Math Solutions

6. 4 x [24-{110- \(\overline{11+3}\) x4) ÷ 9}] ÷ 2 of 9
Solution:

4 x [24-{110- \(\overline{11+3}\) x 4) ÷ 9}] ÷ 2 of 9

= 4 x [24 – {110-14×4) ÷ 9}] ÷18.

= 4 x [24 – {(110-56) ÷ 9}] ÷18.

= 4 x [24 – {54 ÷ 9}] ÷18 = 4 x [24 – 6] ÷18

= 4 x 18 ÷ 18

= 4 x 1

= 4

7. 200 ÷ [88-{(12×13)-3(40-9)}]
Solution:

200 ÷ [88 -{(12×13)- 3(40 – 9)}]

= 200 ÷ [88-{156-3×31}]

= 200 ÷ [88-{156-93}]

= 200 ÷ [88 – 63]

= 200 ÷ 25

= 8

200 ÷ [88 -{(12×13)- 3(40 – 9)}] = 8

8. (987- \(\overline{43+25}\))-10[5 + {(999 ÷ \(\overline{9×3}\)+(\(\overline{8×9}\) ÷ 6)4}]
Solution:

(987- \(\overline{43+25}\))-10[5 + {(999 ÷ \(\overline{9×3}\)+(\(\overline{8×9}\) ÷ 6)4}]

= (987 – 68) – 10 [5 + {999 ÷ 27 + (72 ÷ 6)4}]

= 919 -10 [5 + {37+12 of 4}]

= 919-10 [5+ {37+ 48}]

= 919-10 [5+ 85]

= 919-10 x 90

= 919 – 900

= 19

Class 6 WBBSE Math Solutions

Question 4. Let us form a story for the following simplification and then solve:

1. You bring 12 eggs from the market, but 2 eggs are rotten. Now you divide the rest of the eggs between 2 of your friends. Find how many eggs each will get.
Solution:

(12-2) ÷ 2

=10 ÷2 = 5

∴ Each will get 5 eggs.

2. You have 90 rupees, you spent Rs. 48 on books. Again, your father gives you Rs. 21. With this total money you buy 7 pens of equal price. What is the price of each pen?
Solution:

(90-48+ 21) ÷ 7

= (42 + 21) ÷ 7

= 63 ÷ 7

= 9

∴ Price of each pen = Rs. 9.

Question 4. Let us express in mathematical language and solve:

Rajdeep’s father sold 125 guavas from their guava orchard at Rs. 2 each to Baruipur market. With the money, he bought 2 pens for Rs. 5 each and 2 exercise books for Rs. 20 each and divided the remaining money among two brothers and sisters to buy sweets. Let us find out how much money Rajdeep got for sweets.
Solution:

The total amount of money Rajdeep’s father received by selling 125 guavas at Rs. 2 each = 125 x Rs. 2 = Rs. 250

Now, he bought 2 pens for Rs. 5 each = Rs. 5×2 = Rs. 10 and he bought 2 exercise books for 20 each = Rs. 20 x 2 = Rs 40

∴  Total amount he spent = Rs. 10 + Rs. 40 = Rs. 50

Rest amount = Rs. 250 -(40 + 10) = 200

Each will get = Rs. 200 ÷ 2 = Rs. 100.

∴ In mathematical language,

[125 x 2-{(5×2)+ (20×2)}] ÷ 2

= [250-{10+ 40}] ÷ 2

= [250-50] ÷ 2

= 200 ÷ 2

= 100

WBBSE Math Solutions Class 6 Fraction Excercise 1.2

Question 1. 6 multiples of 5 which do not not contain ‘O’.
Solution: 5,15,25, 35, 45,55.

Question 2. Let us find 3 multiples of 7 which are greater than 50.
Solution: 56, 63, 70.

Question 3. Let us think and find 2-digit numbers which are multiples of 4.
Solution: 12,16,20,24,28,32,36,40.

Question 4. Let’s find three numbers which are the factors of 4.
Solution: 1,2, 4.

Question 5. Let’s find two numbers whose L.C.M. is 12 and their sum is 10.
Solution: The required numbers are 4 and 6as4 + 6 = 10 and L.C.M of 4 and 6 = 12.

Question 6. What are the prime factors of 14?
Solution: Prime factors of 14: 2, 7.

Question 7. What is the least prime number?
Solution: The least prime number is 2.

Question 8. Which number is neither prime nor composite?
Solution: 1

Question 9.

1. 42 is a multiple of which of the following numbers?

1. 7
2. 13
3. 5
4. 6

Solution: 42 is a multiple of

1. 7,

4. 6.

2. Which of the following numbers has 11 as a factor?

1. 101
2. 111
3. 121
4. 112

Solution: 3. 121.

WBBSE Math Solutions Class 6

Question 10. Which of the following pairs of numbers are mutually prime?

1. 5, 7
2. 10, 21
3. 10, 15
4. 16, 15.

Solution: 1. 5, 7

Question 11. Let us find two pairs of composite numbers which are mutually prime.
Solution: (4,15) and (4,9).

Question 12.

1. Let’s write H.C.F. of mutually prime numbers,
2. Let’s write L.C.M. of mutually prime numbers.

Solution:

Let the two prime numbers are 15 and 16.

1. H.C.F of 15 and 16 is 1.
2. L.C.M of 1 5 and 1 6 is (15 x 16) = 24.

Question 13. Finding prime factors and let’s find H.C.F. of the following

1. 22,44;
2. 54, 72;
3. 27,64;
4. 36,30;
5. 28, 35, 49;
6. 30, 72, 96;
7. 20, 40, 80.

Solution:

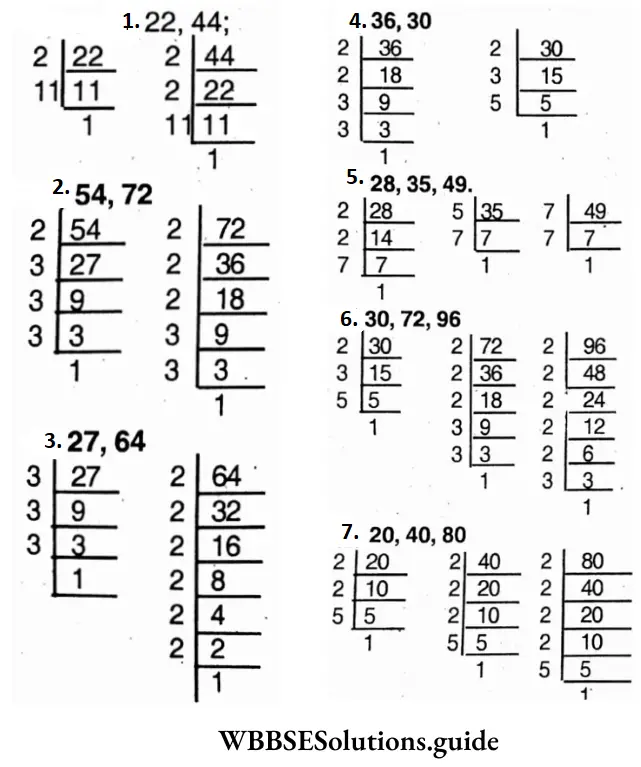
1. 22 = 2 x 1 1 x 1; 44 = 2 x 2 x 1 1 x 1

∴ H.C.F. = 2×11 x 1 =22

2. 54 = 2 x 3 x 3 x 3 x 1; 72 = 2x2x2x3x3x1

∴ H.C.F. = 2 x 3 x 3x 1 =18

3. 27 = 3 x 3 x 3 x 1; 64=2x2x2x2x2x2x1

∴ H.C.F =1

4. 36 = 2 x 2 x 3 x 3 x 1; 30 = 2x3x5x1

∴ H.C.F. = 2x3x1 =6

5. 28 = 2x2x7x1; 35 = 5 x 7 x 1; 49 = 7x7x1

∴ H.C.F. = 7×1=7

6. 30 = 2 x 3 x 5 x 1; 72 = 2x2x2x3x3x1; 96=2x2x2x2x2x3x1

∴ H.C.F =2x3x1=6

7. 20 = 2 x 2 x 5 x 1; 40 = 2x2x2x5x1; 80=2x2x2x2x5x1

∴ H.C.F = 2x2x5x1=20

WBBSE Math Solutions Class 6

Question 14. Let’s find L.C.M. of the following by division method

1. 28, 35;
2. 54, 72;
3. 27, 63;
4. 25, 35, 45;
5. 48, 72, 96.

Solution:

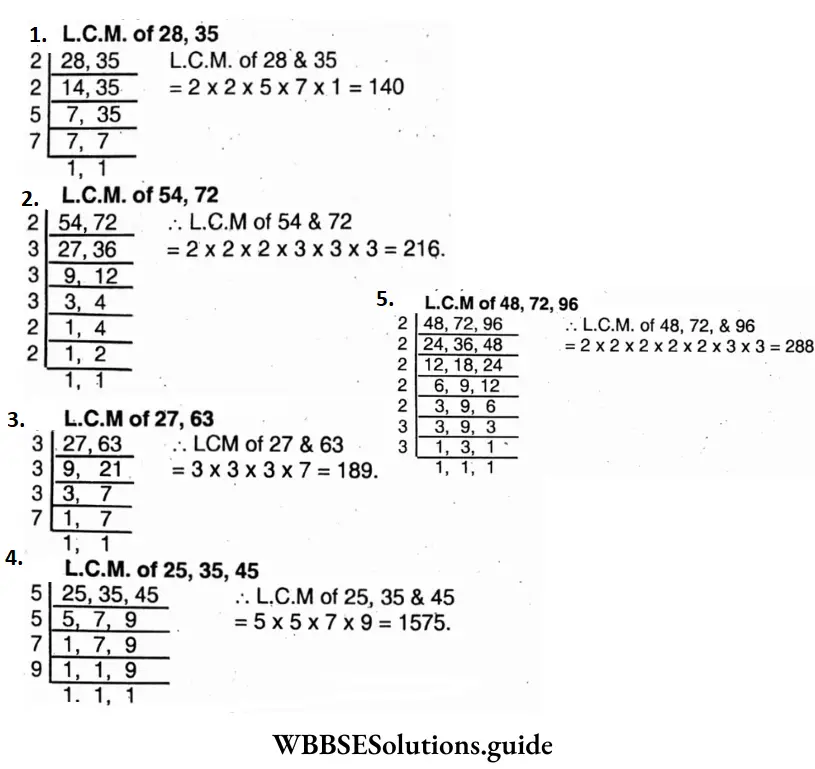
Question 15. Let’s find L.C.M. of the following by finding their prime factors

1. 25, 80;
2. 36, 39;
3. 32, 56;
4. 36, 48 and 72;
5. 25, 35 and 45;
6. 32,
7. 40 and 84.

Solution:

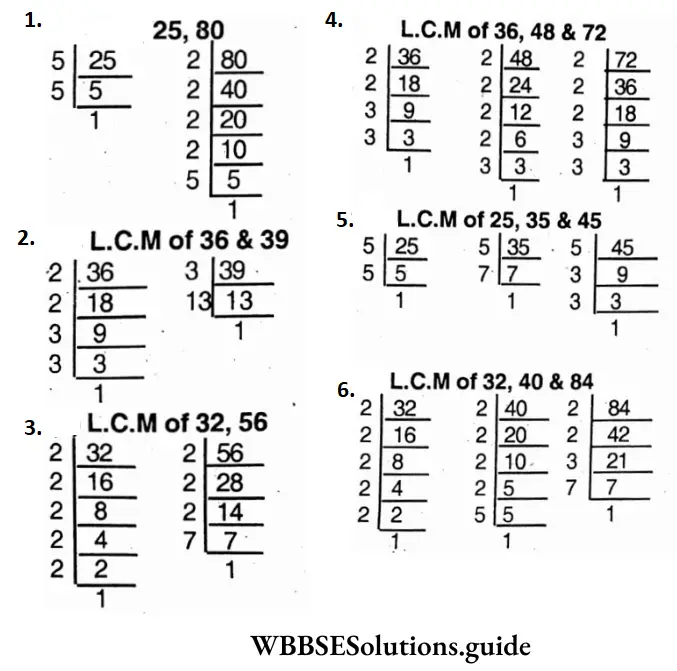
1. 25 = 5 x 5 x 1; 80=2x2x2x2x5x1

∴ L.C.M of 25, 35 & 45

2. 6 = 2 x 2 x 3 x 3 x 1; 39 = 3 x 13 x 1

∴ L.C.M. of 36 and 39 =2x2x3x3x13 = 468.

3. 32 = 2x2x2x2x2x1; 56 = 2x2x2x7

∴ L.C.M. of 32 and 56 =2x2x2x 2x2x7 = 224

4. 36 = 2 x 2 x 3 x 3 x 1; 48=2x2x2x2x3x1; 72 = 2x2x2x3x3x1

∴ L.C.M of 36, 48 and 72 = 2x2x2x2x3x3 = 144

5. 25 = 5 x 5 x 1; 35 = 5 x 7 x 1; 45 = 3x3x5x1

∴ L.C.M. of 25,35 and 45 = 3x3x5x5x7=1575

6. 32 = 2x2x2x2x2x1; 40 = 2 x 2 x 2 x 5 x 1; 84 = 2x2x3x7x1

∴ L.C.M. of 32, 40 and 84 =2x2x2x2x2x3x5x1 = 3360

WBBSE Math Solutions Class 6

Question 16. Let’s find which pairs of numbers are mutually prime 

1. 47, 23;
2. 25, 9;
3. 49,35;
4. 36, 54.

Solution:

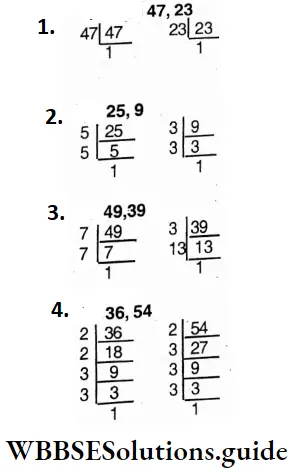
1. 47 = 47×1; 23 = 23 x 1

There are no common factors between 47 and 23.

∴ They are mutually prime.

2. 25 = 5 x 5 x 1; 9 = 3 x 3 x1

There are no common factors between 25 and 9.

∴ They are mutually prime.

3. 49 = 7 x 7 x 1; 39 = 3x13x1

There are no common factors between 49 and 39.

∴ They are mutually prime.

4. 36 = 2 x 2 x 3 x 3 x 1; 54 = 2x3x3x3x1

There are three common factors (2,3,3).

∴ 36  and 54 are not mutually prime.

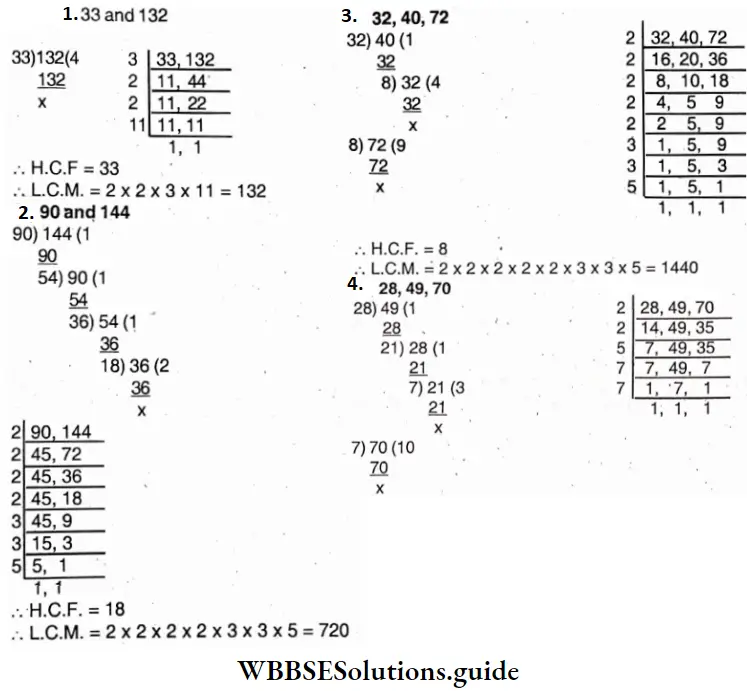
Question 18. Let’s find H.C.F, and L.C.M. of the following numbers by short division method:

1. 33 and 132,
2. 90 and 144,
3. 32, 40 and 72,
4. 28, 49, 70

Solution:

Question 19. Let’s find the least number which is divisible by 18, 24 and 42.
Solution:

First we have to find the L.C.M. of the least numbers.

L.C.M. = 2x2x2x3x3x7 = 504

∴ The required L.C.M = 504

Question 20. Let’s find the greatest number which when divided 45 and 60 does not leave any remainders.
Solution:

To find the greatest number we have to find the H.C.F. of 45 and 60.

∴ The required greatest number 15

Question 21. Let’s find the product of two numbers whose L.C.M. and H.C.F are 252 and 6 respectively.
Solution:

Product of two numbers = L.C.M. x H.C.F. = 252 x 6 = 1512

Question 22. If the H.C.F. and L.C.M. of two numbers are 8 and 280 and one of the numbers is 56, let’s find the other number.
Solution:

Product of two numbers = L.C.M. x H.C.F.

One number = \(\frac{\text { L.C.M } \times \text { H.C.F }}{\text { other number }}=\frac{280 \times 8}{56}=40\)

Question 23. Let’s write two numbers whose H.C.F. is 1.
Solution:

H.C.F. of two prime numbers is one. Similarly the H.C.F. of one prime number and one another number is one.

Question 24. Let’s find the maximum number of people among whom 48 rasogollas and 64 sandeshes are to be distributed in equal numbers.
Solution:

To find the number of people we have to find the H.C.F of 48 and 64.

H.C.F = 16

∴ The required number of people = 16.

WBBSE Math Solutions Class 6

Question 25. Bibhash and his friends decided to form a drama group of either 8 or 10 people. Let’s find the minimum number of people who will be able to form both types of groups.
Solution:

To find the number of people, we have to find the L.C.M. of 8 and 10.

L.C.M. =2x2x2x5 = 40

∴ The required number of people is 40

Question 26. Panchayat has sent flower saplings to the students of class 6 of Jodunath Vidya Mandir for their school garden. It was found that if the saplings can be put in rows of 20, 24 and 30, then the number of sap lings in each row will be equal. Let’s find the minimum number of saplings that Panchayat sent to school.
Solution:

Given

Panchayat has sent flower saplings to the students of class 6 of Jodunath Vidya Mandir for their school garden. It was found that if the saplings can be put in rows of 20, 24 and 30, then the number of sap lings in each row will be equal.

To find the minimum number of saplings, we have to find the L.C.M. of 20, 24 and 30.

L.C.M. of 20, 24, 30

L.C.M. = 2x2x2x3x5=120

∴ The minimum required of flower saplings = 120

Question 27. The perimeter of the front wheel and rear wheel of an engine are 14 dcm and 35 dcm respectively. Let’s find the minimum distance the engine can cover before both wheels take complete revolutions together.
Solution:

Given

The perimeter of the front wheel and rear wheel of an engine are 14 dcm and 35 dcm respectively.

To find the minimum distance we have to find the L.C.M of 14 and 25

∴ L.C.M = 2x5x7 = 70.

∴ The required minimum distance = 70 dcm.

Question 28. For each of the following cases, let’s write two numbers whose:

1. H.C.F. is 7,
2. L.C.M. is 12,
3. H.C.F. is (put 1 digit number),
4. L.C.M. is (Put 1 digit number.)

Solution:

1. The required numbers are 7 and 14 as H.C.F. of 7 and 14 is 7.
2. The required numbers are 3 and 12 as L.C.M of 3 and 12 is 12.
3. The required numbers are 4 and 8. H.C.F. = 4
4. The required numbers are 3 and 6. L.C.M. = 6

Fraction Exercise 1.3

Question 1. I took a white rectangular piece of paper. I divided it horizontally into 3 equal parts and coloured. In the colours of our national flag, find the proportion of each colour.
Solution: Portion of saffron colour = 1/3 part

Portion of white colour = 1/3 part

Portion of green colour = 1/3 part

Question 2. Seema took a paper, same as mine, but coloured it different. Let’s find how and what portion she coloured.
Solution:

Portion of saffron colour = 2/3 part

1/2 part is coloured.

Let’s colour 1/4 part

Portion of green colour = 1/3 part

3/5 part is coloured

3/4 part is coloured

2/5 is coloured

1/6 is coloured

2/6 is coloured

4/10 is coloured

1/2 is coloured

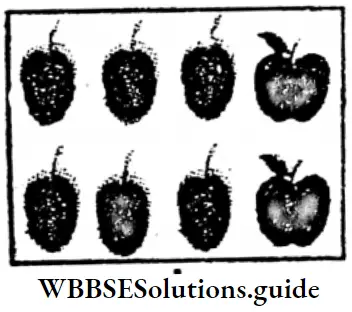
Question 3. Let me find what part of the mangoes I disposed of.

1/3 part of the number of mangoes collected are rotten.

In the picture 1/3 part of mangoes are circled with red —>

It is found, rotten mangoes are 1/3 part = 4 mangoes.

From picture it is found 2/3 part of mangoes are fish.

∴ 2/3 part of total number of mangoes = 8 mangoes.

Hence, from picture it is found, that 4 mangoes are rotten and 8 mangoes are fresh.

WBBSE Math Solutions Class 6

Question 4. Let’s find out from my picture in which parts the different fruits are present.
Solution:

In the picture, out of the 4 equal parts of the fruits, the apples are 1/4 part.

That is, in the picture number of apples are 1/4 part of the total number of furits.

In the picture, mango is 3 part of 4 equal parts.

That is, in the picture mango is 3/4 part of the total number of fruits.

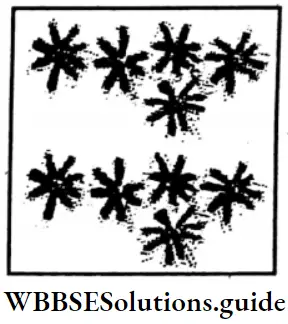
In the picture aside, red flowers are 2 part of 3 equal parts of flowers.

Thus, 10/15 part of flowers are red flowers.

White flowers are 1 part of 3 equal parts of flowers.

Thus, 5/15 part of flowers are white flowers.

∴ 2/5 parts = 2 parts out of 5 equal parts = 4 balls.

∴ 1/4 parts = 1 part out of 4 equal parts = 2 coins.

Question 5. I Painted ted one of the window panes and a part of the other pane. Let me find, how much has been painted.
Solution:

Coloured → \(\left(2+\frac{1}{2}\right)=2 \frac{1}{2}=\frac{2 \times 2+1}{2}=\frac{5}{2}\)

Coloured → \(\left(2+\frac{1}{6}\right)=2 \frac{1}{6}=\frac{2 \times 6+1}{6}=\frac{13}{6}\)

Question 6. Let’s find four equivalent fractions for each of the fractions 2/3 and 5/9
Solution:

Four equivalent fractions of 2/3 are

1. 4/6 [Multiply numerator and denominator by 2]
2. 6/9 [Multiply numerator and denominator by 3]
3. 8/12 [Multiply numerator and denominator by 4]
4. 10/15 [Multiply numerator & denominator by 5]

Four equivalent fractions of 5/9 are

1. 10/18 [Multiply numerator and denominator by 2]
2. 15/27 [Multiply numerator and denominator by 3]
3. 20/36 [Multiply numerator and denominator by 4]
4. 25/45 [Multiply numerator and denominator by 5]

WBBSE Class 6 Maths Solutions Fraction Exercise 1.4

Question 1. Let us write in blank boxes:
Solution:

1. 7/10 part is coloured.
2. 5/6 part is not coloured.
3. 2/5 part is coloured.

Question 2. Let us write in blank boxes:

1. When we say half bread, what part of the whole bread we see?
Solution:

When we say half bread, what part of the whole bread we see 1-1/2 = 1/2 part.

2. I have a big chocolate. Dividing it into 8 pieces, I gave 3 pieces to my sister, 2 pieces to my brother and rest I ate myself. Let’s find what parts of chocolate we individually ate.
Solution:

My sister got 2/8 part of the chocolate.

My brother got 2/8 part = 1/4 part

∴ Remaining part = \(\left[1-\left(\frac{3}{8}+\frac{1}{4}\right)\right]\)

= \(\left[1-\left(\frac{3+2}{8}\right)\right]\)

= \(\left[1-\frac{5}{8}\right]=\frac{8-5}{8}=\frac{3}{8} \text { part }\)

∴ Part of chocolate I received = 3/8 part.

3. Let us write all numbers from 1 to 10. Out of these numbers what fraction are the prime numbers?
Solution:

The prime numbers between 1 to 10 are 2,3,5, and 7.

Total number=4 Fractional part of the prime number = 4/10 = 2/5 part.

WBBSE Class 6 Maths Solutions

4. There are few oranges in a basket. After giving half of the oranges to my grandfather, 2 oranges are left. Let’s find how many oranges were there in the basket.
Solution:

Number of oranges = \(2 \div \frac{1}{2}=2 \times \frac{2}{1}\) = 4

5. In two glasses of equal size equal amount of lemon drink was prepared. One glass contains 1/5 part of sugar and the other contains 2/7 part of sugar. Let’s find without taking the drinks, which glass of lemon drink tastes sweeter.
Solution:

∴ \(\frac{2}{7}\) part of sugar glass of lemon drink testes sweeter.

6. \(\frac{5}{7}\) part of school gate has been painted. Let’s find out which part is still to be painted.
Solution:

⇒ \(\frac{5}{7}\) part of school gate has been painted.

Rest part of the gate which is still to be painted = \(\text { 1. }-\frac{5}{7}=\frac{7-5}{7}=\frac{2}{7} \text { part }\)

7. I have Rs. 20.1 spent Rs. 5. Let’s find what part I spent and what part is left with me.
Solution:

Total amount = Rs. 20.

I spent Rs. 5 = \(\frac{5}{20}\) = \(\frac{1}{4}\) part.

Remaianing part = \(\left(1-\frac{1}{4}\right) \text { part }=\left(\frac{4-1}{4}\right) \text { part }=\frac{3}{4} \text { part. }\)

8. Rajia has 36 berries. She will give me — part of her berries. Let me find out how many berries she will give me.
Solution:

Rajia has 36 berries.

She will give me = \(\frac{2}{3}\) of 36 berries = (2 x 12) = 24 berries.

Question 4. Let us mark proper fraction by ; improper fraction by and mixed fraction by Δ
Solution:

Let us mark

1. Proper fraction
2. Imoroper fraction
3. Mixed fraction Δ

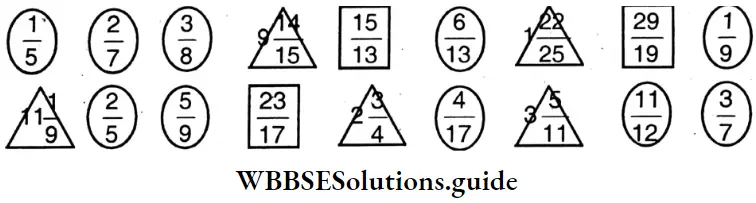
Proper fraction: \(\frac{1}{5}, \frac{2}{7}, \frac{3}{8}, \frac{6}{13}, \frac{1}{9}, \frac{2}{5}, \frac{5}{9}, \frac{4}{17}, \frac{11}{12}, \frac{3}{7}\)

Improper fraction: \(\frac{15}{13}, \frac{29}{19}, \frac{23}{17}\)

⇒ \(9 \frac{14}{15}, 1 \frac{22}{25}, 11 \frac{1}{9}, 2 \frac{3}{4}, 3 \frac{5}{11}\)

Mixed fraction: \(9 \frac{14}{15}, 1 \frac{22}{25}, 11 \frac{1}{9}, 2 \frac{3}{4}, 3 \frac{5}{11}\)

WBBSE Class 6 Maths Solutions

Question 5. Let us write three equivalent fractions for each of the following

1. \(\frac{1}{5}\)
2. \(\frac{2}{5}\)
3. \(1 \frac{1}{3}\)
4. \(6 \frac{1}{6}\)
5. \(3 \frac{4}{5}\)

Solution:

1. \(\frac{1}{5}\)

1st equivalent fraction of \(\frac{1}{5}=\frac{2}{10}\)

2nd equivalent fraction of \(\frac{1}{5}=\frac{3}{15}\)

3rd equivalent fraction of \(\frac{1}{5}=\frac{4}{20}\)

2. \(\frac{2}{5}\)

1st equivalent fraction of \(\frac{2}{5}=\frac{4}{10}\)

2nd equivalent fraction of \(\frac{2}{5}=\frac{6}{15}\)

3rd equivalent fraction of \(\frac{2}{5}=\frac{8}{20}\)

3. 1\(\frac{1}{3}=\frac{4}{3}\)

1st equivalent fraction of \(\frac{4}{3}=\frac{8}{6}\)

2nd equivalent fraction of \(\frac{4}{3}=\frac{12}{9}\)

3rd equivalent fraction of \(\frac{4}{3}=\frac{16}{12}\)

4. 6\(\frac{1}{6}=\frac{37}{6}\)

1st equivalent fraction of \(\frac{37}{6}=\frac{74}{12}\)

2nd equivalent fraction of \(\frac{37}{6}=\frac{111}{18}\)

3rd equivalent fraction of \(\frac{37}{6}=\frac{148}{24}\)

5. 3\(\frac{4}{5}=\frac{19}{5}\)

1st equivalent fraction of \(\frac{19}{5}=\frac{38}{10}\)

2nd equivalent fraction of \(\frac{19}{5}=\frac{57}{15}\)

3rd equivalent fraction of \(\frac{19}{5}=\frac{76}{20}\)

Question 6. Let us express the following in their lowest form:

1. \(\frac{28}{49}\)
2. \(\frac{54}{81}\)
3. \(\frac{72}{108}\)
4. \(\frac{243}{405}\)
5. \(\frac{165}{180}\)

Solution:

1. \(\frac{28}{49}=\frac{4}{7}\)

2. \(\frac{54}{81}=\frac{18}{27}=\frac{2}{3}\)

3. \(\frac{72}{108}=\frac{2}{3}\)

4. \(\frac{243}{405}=\frac{3}{5}\)

5. \(\frac{165}{180}=\frac{11}{12}\)

WBBSE Class 6 Maths Solutions

Question 7. Let us arrange the following fractions from smaller to bigger in ascending order

1. \(\frac{7}{2}, \frac{4}{4}, \frac{7}{5}\)
Solution:

L.C.M. of 2,4,5=20

⇒ \(\frac{7}{2}=\frac{7 \times 10}{2 \times 10}=\frac{70}{20}\)

⇒ \(\frac{7}{4}=\frac{7 \times 5}{4 \times 5}=\frac{35}{20}\)

⇒ \(\frac{7}{5}=\frac{7 \times 4}{5 \times 4}=\frac{28}{20}\)

∴ \(\frac{28}{20}<\frac{35}{20}<\frac{70}{20}\)

i.e., \(\frac{7}{5}<\frac{7}{4}<\frac{7}{2}\)

2. \(5 \frac{3}{4}, 5 \frac{5}{9}, 5 \frac{8}{12}\)
Solution:

⇒ \(5 \frac{3}{4}, 5 \frac{5}{9}, 5 \frac{8}{12}\)

or, \(\frac{23}{4}, \frac{50}{9}, \frac{68}{12}\)

L.C.M of 4,9 and 12=36

⇒ \(\frac{23}{4}=\frac{23 \times 9}{4 \times 9}=\frac{207}{36}\)

⇒ \(\frac{50}{9}=\frac{50 \times 4}{9 \times 4}=\frac{200}{36}\)

⇒ \(\frac{68}{12}=\frac{68 \times 3}{12 \times 3}=\frac{204}{36}\)

⇒ \(\frac{200}{36}<\frac{204}{36}<\frac{207}{36}\)

or, \(\frac{200}{36}<\frac{204}{36}<\frac{207}{36}\)

or, \(\frac{50}{9}<\frac{68}{12}<\frac{23}{4}\)

∴ \(5 \frac{5}{9}<5 \frac{8}{12}<5 \frac{3}{4}\)

3. \(1 \frac{1}{5}, 1 \frac{1}{7}, 1 \frac{1}{8}\)
Solution:

⇒ \(1 \frac{1}{5}, 1 \frac{1}{7}, 1 \frac{1}{8}\)

or, \(\frac{6}{5}, \frac{8}{7}, \frac{9}{8}\)

L.C.M. of 5, 7, 8 = 280

⇒ \(\frac{6}{5}=\frac{6 \times 56}{5 \times 56}=\frac{336}{280}\)

⇒ \(\frac{8}{7}=\frac{8 \times 40}{7 \times 40}=\frac{320}{280}\)

⇒ \(\frac{9}{8}=\frac{9 \times 35}{8 \times 35}=\frac{315}{280}\)

∴ \(\frac{315}{280}<\frac{320}{280}<\frac{336}{280}\)

i.e., \(\frac{9}{8}<\frac{8}{7}<\frac{6}{5}\)

⇒ \(1 \frac{1}{8}<1 \frac{1}{7}<1 \frac{1}{5}\)

Class 6 Math WBBSE Solutions

4. \(\frac{1}{3}, \frac{4}{5}, \frac{7}{15}\).
Solution:

L.C.M of 3,5 and 15=15

⇒ \(\frac{1}{3}=\frac{1 \times 5}{3 \times 5}=\frac{5}{15}\)

⇒ \(\frac{4}{5}=\frac{4 \times 3}{5 \times 3}=\frac{12}{15}\)

⇒ \(\frac{7}{15}=\frac{7 \times 1}{15 \times 1}=\frac{7}{15}\)

∴ \(\frac{5}{15}<\frac{7}{15}<\frac{12}{15}\)

i.e., \(\frac{1}{3}<\frac{7}{15}<\frac{4}{5}\)

5. \(\frac{5}{7}, \frac{3}{4}, \frac{1}{4}\)
Solution:

L.C.M. of 7,4 and 4=28

⇒ \(\frac{5}{7}=\frac{5 \times 4}{7 \times 4}=\frac{20}{28}\)

⇒ \(\frac{3}{4}=\frac{3 \times 7}{4 \times 7}=\frac{21}{28}\)

⇒ \(\frac{1}{4}=\frac{1 \times 7}{4 \times 7}=\frac{7}{28}\)

∴ \(\frac{7}{28}<\frac{20}{28}<\frac{21}{28}\)

∴ \(\frac{1}{4}<\frac{5}{7}<\frac{3}{4}\)

6. \(3 \frac{1}{2}, 7 \frac{5}{9}, 7 \frac{1}{5}\)
Solution:

⇒ \(3 \frac{1}{2}, 7 \frac{5}{9}, 7 \frac{1}{5}\)

⇒ \(\frac{7}{2}, \frac{68}{9}, \frac{36}{5}\)

L.C.M. of 2,9 and 5=90

⇒ \(\frac{7}{2}=\frac{7 \times 45}{2 \times 45}=\frac{315}{90}\)

⇒ \(\frac{68}{9}=\frac{68 \times 10}{9 \times 10}=\frac{680}{90}\)

⇒ \(\frac{36}{5}=\frac{36 \times 18}{5 \times 18}=\frac{648}{90}\)

⇒ \(\frac{315}{90}<\frac{648}{90}<\frac{680}{90}\)

or, \(\frac{7}{2}<\frac{36}{5}<\frac{68}{9}\)

∴ \(3 \frac{1}{2}<7 \frac{1}{5}<7 \frac{5}{9}\)

Class 6 Math WBBSE Solutions

7. \(\frac{1}{8}, \frac{7}{10}, \frac{3}{5}\)
Solution:

L.C.M. of 8,10 and 5=40

⇒ \(\frac{1}{8}=\frac{1 \times 5}{8 \times 5}=\frac{5}{40}\)

⇒ \(\frac{7}{10}=\frac{7 \times 4}{10 \times 4}=\frac{28}{40}\)

⇒ \(\frac{3}{5}=\frac{3 \times 8}{5 \times 8}=\frac{24}{40}\)

⇒ \(\frac{5}{40}<\frac{24}{40}<\frac{28}{40}\)

∴ \(\frac{1}{8}<\frac{3}{5}<\frac{7}{10}\)

8. \(3 \frac{1}{2}, 3 \frac{5}{9}, 3 \frac{1}{5}\)
Solution:

⇒ \(3 \frac{1}{2}, 3 \frac{5}{9}, 3 \frac{1}{5}\)

or, \(\frac{7}{2}, \frac{32}{9}, \frac{16}{5}\)

L.C.M. of 2,9 and 5=90

⇒ \(\frac{7}{2}=\frac{7 \times 45}{2 \times 45}=\frac{315}{90}\)

⇒ \(\frac{32}{9}=\frac{32 \times 10}{9 \times 10}=\frac{320}{90}\)

⇒ \(\frac{16}{5}=\frac{16 \times 18}{5 \times 18}=\frac{288}{90}\)

⇒ \(\frac{288}{90}<\frac{315}{90}<\frac{320}{90}\)

or, \(\frac{16}{5}<\frac{7}{2}<\frac{32}{9}\)

∴ \(3 \frac{1}{5}<3 \frac{1}{2}<3 \frac{5}{9}\)

Question 8. Let us find the values of the following

1. \(\frac{2}{7}+\frac{2}{3}+1 \frac{1}{2}\)
Solution:

= \(\frac{2}{7}+\frac{2}{3}+\frac{3}{2}\)

= \(\frac{2 \times 6+2 \times 14+3 \times 21}{42}\)

= \(\frac{12+28+63}{42}=\frac{103}{42}\)

= \(2 \frac{19}{42}\)

2. \(1 \frac{2}{5}-\frac{3}{8}+\frac{1}{4}\)
Solution:

L.C.M Of 5, 8 and 4

= \(\frac{7}{5}-\frac{3}{8}+\frac{1}{4}\)

= \(\frac{7 \times 8-3 \times 5+1 \times 10}{40}\)

= \(\frac{56-15+10}{40}\)

= \(\frac{51}{40}\)=1 \(\frac{11}{40}\)

Class 6 Math WBBSE Solutions

 

3. \(\frac{2}{5}+\frac{3}{8}-\frac{1}{4}\)
Solution: 

L.C.M Of 5, 8 and 4

= \(\frac{2 \times 8+3 \times 5-1 \times 10}{40}\)

= \(\frac{16+15-10}{40}=\frac{21}{40}\)

4. \(7-3 \frac{1}{8}-2 \frac{1}{3}\)
Solution:

L.C.M of 1, 8 and 3 = 24

= \(\frac{7}{1}-\frac{25}{8}-\frac{7}{3}\)

= \(\frac{24 \times 7-25 \times 3-7 \times 8}{24}\)

= \(\frac{168-75-56}{24}\)

= \(\frac{37}{24}=1 \frac{13}{24}\)

5. \(\frac{4}{5}+\frac{5}{8}-1 \frac{1}{3}\)
Solution: 

L.C.M of 5, 8 and 3

= \(\frac{4}{5}+\frac{5}{8}-\frac{4}{3}\)

= \(\frac{4 \times 24+5 \times 15-4 \times 40}{120}\)

= \(\frac{96+75-160}{120}\)

= \(\frac{171-160}{120}\)

= \(\frac{11}{120}\)

L.C.M = 2x2x2x3x5 =120

Class 6 Math WBBSE Solutions

6. \(1 \frac{3}{10}+1 \frac{4}{5}-1 \frac{1}{4}\)
Solution: 

L.C.M of 10, 5 and 4

= \(\frac{13}{10}+\frac{9}{5}-\frac{5}{4}\)

= \(\frac{13 \times 2+9 \times 4-5 \times 5}{20}\)

L.C.M = 2 x2 x 5 = 20

= \(\frac{26+36-25}{20}\)

= \(\frac{37}{20}=1 \frac{17}{20}\)

7. \(2 \frac{5}{6}-1 \frac{8}{9}+1 \frac{3}{4}\)
Solution: 

L.C.M of 6, 9, 4

= \(\frac{17}{6}-\frac{17}{9}+\frac{7}{4}\)

= \(\frac{17 \times 6-17 \times 4+7 \times 9}{36}\)

= \(\frac{102-68+63}{36}\) L.C.M = 2 x 2x 3x 3 = 36

= \(\frac{97}{36}=2 \frac{25}{36}\)

8. \(4 \frac{1}{7}\)+2 \(\frac{2}{5}-5\)
Solution: 

L.C.M of 7, 5 and 1

= \(\frac{29}{7}+\frac{12}{5}-\frac{5}{1}\) = 7 x 5x 1 = 35

= \(\frac{29 \times 5+12 \times 7-5 \times 35}{35}\)

= \(\frac{145+84-175}{35}\)

= \(\frac{54}{35}\)

= \(1 \frac{19}{35}\)

Question 9. Let us work out

1. During tiffin hours of school \(\frac{1}{4}\) part of drinking water of a tank was used. Another \(\frac{1}{3}\) part of water was used when school got over. Let’s find what part of water is still left in the tank.
Solution:

Let total amount of water = 1 part

Water used = \(\left(\frac{1}{4}+\frac{1}{3}\right) \text { part }=\frac{3+4}{12}=\frac{7}{12} \text { part }\)

∴ Remaining water = \(1-\frac{7}{12}=\frac{12-7}{12}=\frac{5}{12}\) part of the thank.

2. Today, I took a piece of cake for tiffin. My two friends Ayush ar Sabana respectively ate \(\frac{1}{3}\) part and \(\frac{2}{5}\) part of the cake. I ate the remaining part. Let me find who ate the most part of cake.
Solution:

Let total part of cake = 1

Ayush and Sabana ate = \(\frac{1}{3}+\frac{2}{5}\)

= \(\frac{5+6}{15}=\frac{11}{15}\)

Remaining part = \(1-\frac{11}{15}=\frac{15-11}{15}=\frac{4}{15}\) part

Now Ayush, Sabana and I get \(\frac{1}{3}, \frac{2}{5} and \frac{4}{15}\) parts of the cake respectively.

⇒ \(\frac{1}{3}=\frac{1 \times 5}{3 \times 5}=\frac{5}{15}\)

⇒ \(\frac{2}{5}=\frac{2 \times 3}{5 \times 3}=\frac{6}{15}\)

⇒ \(\frac{4}{15}=\frac{4 \times 1}{15 \times 1}=\frac{4}{15}\)

∴ \(\frac{2}{5}\) is the greatest part.

Sabana ate most part of the cake.

Class 6 Math WBBSE Solutions

3. Out of 25 bighas of land Ratanbabu cultivated jute in 16 bigha But, out of 15 bighas of land, Ushadebi cultivated jute in 8 bighas. Let work out to find in what part of their land Ratanbabu and Ushade cultivated jute and who cultivated jute more.
Solution:

Given

Out of 25 bighas of land Ratanbabu cultivated jute in 16 bigha But, out of 15 bighas of land, Ushadebi cultivated jute in 8 bighas.

Ratanbabu cultivated jute in \(\frac{16}{25}\) part of his land. Ushadebi cultivated jute in \(\frac{8}{15}\) part of her land.

⇒ \(\frac{16}{25}=\frac{16 \times 3}{25 \times 3}=\frac{48}{75}\) L.C.M. of 25 and15

⇒ \(\frac{8}{15}=\frac{8 \times 5}{15 \times 5}=\frac{40}{75}\)

⇒ \(\begin{array}{ccc}
3 & 25, & 15 \\
5 & 25, & 5 \\
5 & 5, & 1 \\
& 1, & 1
\end{array}\)

∴ L.C.M. = 3x5x5 = 75

∴ Ratanbabu cultivated jute more.

4. I have a 15-metre long white ribbon. I cut off \(\frac{1}{3}\) part from it. What part of ribbon is left? Let’s find the length of the remaining part of the ribbon.
Solution:

Total length of white ribbon = 15 metres.

I take = \(\frac{1}{3}\) x 15 m = 5 metre.

Remaining part = \(1-\frac{1}{3}=\frac{3-1}{3}=\frac{2}{3}\) part

And the length of the remaining part of the ribbon = (15 – 5) m = 10 metres.

Class 6 Math Solutions WBBSE Decimal Fractions

We decided to decorate the front portion of the dais of our village club with some coloured tiles of equal size. 2 red tiles were given by me, 3 yellow tiles by Reba, 4 green tiles by Samsur and 1 white tile by Tina.

We all gathered before the dais with a total of 10 tiles.

Out of 10 tiles I gave 2 tiles → \(\frac{2}{10}\) part = 0.2 part = .2

Out of 10 tiles, Reba gave 3 tiles → \(\frac{3}{10}\) part = 0.3 part = .3

Out of 10 tiles, Samsur gave 4 tiles →\(\frac{4}{10}\)part = 0.4 part = .4

Tena give 1 tile → \(\frac{1}{10}\) part = 0.1 part = .1

Hence, \(\begin{array}{cc}
\text { Decimal } & \text { Tenths }\left(\frac{1}{10}\right) \\
\text { number } & \\
0.2 & 2 \\
0.3 & 3 \\
0.4 & 4 \\
0.1 & 1
\end{array}\)

Now, few tiles on floor of the room of our club are broken. We need to replace them.

First, we do arrange for 100 tiles.

I shall give 12 tiles out of those 100 tiles.

I shall give \(\frac{12}{100}\) part = 0.12 part or point one two parts. .

Rohit will give 38 tiles. Thus, he will give \(\frac{38}{100}\)part = 0.38 part.

Mira will give \(\frac{34}{100}\) part = 0.34 part.

Class 6 Math Solutions WBBSE Fraction Exercise 1.5

Question 1. Let us express the coloured portion in terms of decimal fraction

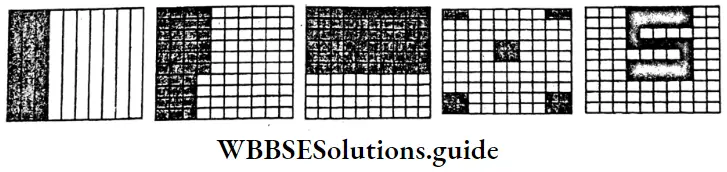
Solution:

1. \(\frac{3}{10}\) part = 0.3

2. \(\frac{36}{100}\) part = 0.36

3. \(\frac{60}{100}\) = 0.6

4. \(\frac{16}{100}\) part = 0.6

5. \(\frac{27}{100}\) part 0.27

Question 2.

1. Let us colour 0.15 part green and 0.53 part yellow. Find total coloured part.

Solution:

0.15 part green and 0.53 part yellow.

∴ Total coloured part = 0.15 + 0.53 = 0.68.

2. Let us colour 0.33 part blue first and 0.15 part red. Find the total uncoloured parts.

Class 6 Math  WBBSE Solution:

0.33 part blue and 0.1 5 part red

∴ Total coloured part = 0.33 + 0.1 5 = 0.48

Total uncoloured part = 1-0.48 = 0.52

Question 3. Let’s prepare a place value table for the following numbers and write the numbers in words.

1. 27.9
Solution:

=27+ \(\frac{9}{10}\)= Twenty seven point nine

= Two tens seven unit and nine tenth

2. 1.28
Solution:

= \(1+\frac{2}{10}+\frac{8}{100}\)

= one point two eight = one unit and two-tenth eight hundredths.

3.65.134
Solution:

65.134 = \(60+5+\frac{1}{10}+\frac{3}{100}+\frac{4}{1000}\)= Sixty five point one three four

= Six tens five units one tenth three hundredth four thousandths.

4. 42.009
Solution:

42.009+40+2+\(\frac{9}{1000}\)= Forty two point zero zero nine

= Four tens two units nine thousandths.

Class 6 Math Solutions WBBSE

5. 38.205
Solution:

= \(30+8+\frac{2}{10}+\frac{5}{1000}\)

= Thirty-eight point two zero five

= Three tens eight units and two-tenth five thousandths.

6. 4003.08
Solution:

4003.08 = Four thousand three point zero eight

= Four thousand three units eight hundedths.

7. 712.5
Solution:

712.5 = 700+10+2+\(\frac{5}{10}\)

= Seven hunderd twelve point five

= Seven hundred one tenth two units five tenths

8. 45.06
Solution:

45.06 = 40+5+\(\frac{6}{100}\)

= Forty-five point zero six

= Four tens five units six hundredths.

Question 4. Let’s convert the following decimal numbers to fractions:

1. 0.3,
2. 0.21,
3. 0.039,
4. 5.4,
5. 102.035.

Solution:

1. 0.3 = \(\frac{3}{10}\)
2. 0.21 = \(\frac{21}{100}\)
3. 0.039 = \(\frac{39}{1000}\)
4. 5.4 = \(\frac{54}{10}\) = \(\frac{27}{5}\)
5. 102.035 = \(\frac{102035}{1000}\) = \(\frac{20407}{200}\) = 102\(\frac{7}{200}\)

Question 5. Let’s arrange the following in increasing order (ascending order):

1. 0.534, 0.52, 5.34, 0.513
Solution: In ascending order 0. 513, 0.52; 0.534, 5.34

2. 0.536, 0.335, 0.3354, 0.52
Solution: In ascending order 0.335; 0.3354; 0.52; 0.536

3. 2.0, 2.005, 20.05, 2.5
Solution: In ascending order 2.0; 2.005; 2.5; 20.05

Question 6. Let’s arrange the following in decreasing order (descending order):

1. 13.3,11.3,1.33,2.31
Solution: In descending order 13.3,11.3, 2.31,1.33

2. 3.007, 3.07, 37.30, 7.13
Solution: In descending order 37.30; 7.13, 3.07, 3.007

3. 0.88, 0.45, 8.45, 0.8217
Solution: In descending order 8.45, 0.88, 0.8217, 0.45

Question 7.

Solution:

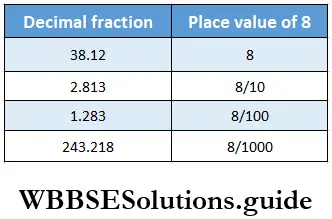
Question 8.

Solution:

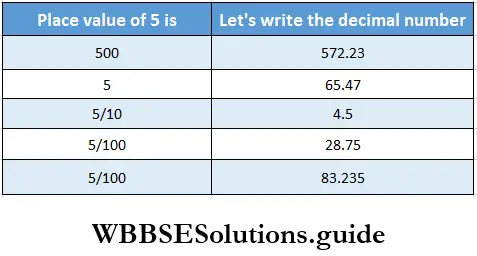
Question 9. Let’s put >, = or < in the boxes given below.

1. 5.0 □ 0.5
Solution: 5.0>0.5

2. 72.1 □ 72.10
Solution: 72.1 =72.10

3. 68.5 □ 68.52
Solution: 68.5 < 68.52

4. 72.93 □ 729.3
Solution: 72.93 <729.3

5. 42.6 □ 42.600
Solution: 42.6 = 42.600

6. 2.33 □ 3.22
Solution: 42.6 = 42.600

7. 924 □ 924.0
Solution: 924 = 924.00

8. □ □ □ (Let us write own decimal numbers)
Solution: 84.67 > 72.67

Question 10. Let’s express the following in decimal numbers:

1. Six tenths
Solution: \(\frac{6}{10}\) = 0.6

2. Nine hundredths
Solution: \(\frac{9}{100}\) = 0.09

3. Two thousandths
Solution: \(\frac{2}{1000}\) = 0.02

4. Two hundred three point four-five
Solution: 203.45

5. Four thousand two units five thousandths
Solution: 4002.005

6. Six hundred twenty-nine point zero five
Solution: 629.005

7. 2 + \(\frac{3}{10}\)
Solution: 2 +0.3 = 2.3

8. 10+7+\(\frac{8}{1000}\)
Solution: 17.008

Question 11. I had Rs. 5. I bought a pen for Rs. 3.50. Let’s find out how much money is left.
Solution: Remaining money = Rs. 5 – Rs. 3.50 = Rs. 1.50.

Question 12. What must be added to 2.75 to get 3?
Solution: Required number = 3 – 2.75 = 0.25.

Question 13. From a string of length 12.5 cm, Mira cuts off 8.5 cm. Let’s find, what portion of the string is left?
Solution: Remaining portion = 12.5 cm – 8.5 cm = 4 cm.

Question 14. The length of my exercise book is □ cm and its breadth is □ cm. Let me find the perimeter of my exercise book.
Solution: Let the length of the exercise book = 18 cm and the breadth of the exercise book = 12 cm.

∴ Perimeter = 2 x (length + breadth)

= 2 x (18 cm + 12 cm) = 2 x 30 cm = 60 cm.

Question 15. For an occasion at our house, father bought rice for Rs. 200, pulses for Rs. 125.50 and vegetables for Rs. 242.50. Let’s calculate the total amount of money spent by father.
Solution: Total amount of money spent = Rs. (200 + 125.50 + 242.50) = Rs. 568.

Question 16. In a long-jump competition, Sahil jumped 182.88 cm and Munna jumped 179.25 cm of length. Let’s find how much more did Sahil jump.
Solution: Sahil jumped more than Munna

= 182.88 cm – 179.25 cm = 3.63 cm.

Question 17. What must be added to 2.172 to get 5?
Solution: Required number = 5-2.172 = 2.828.

Question 18. 2.647 is subtracted from 4.15. How much is to be added to the result to get 10?
Solution: Subtraction of 2.647 from 4.15 = 4.150-2.647=1.503.

∴ Required number = 10 -1.503 = 8.497.

Question 19. Let’s find the values of the following

1. 0.07 + 0.09
Solution: 0.07 + 0.09 = 0.16

⇒ \(\begin{aligned}
& 0.07 \\
& +0.09 \\
& \hline 0.16 \\
& \hline
\end{aligned}\)

2. 4.11 + 1.6
Solution: 4.11 +1.6 = 5.71

⇒ \(\begin{array}{r}
4.11 \\
+1.6 \\
\hline 5.71 \\
\hline
\end{array}\)

3. 312.61 + 276.72
Solution: 589.33

⇒ \(\begin{aligned}
& 312.61 \\
& \underline{276.72} \\
& \underline{589.33} \\
& \hline
\end{aligned}\)

4. 5 – 0.555
Solution: 4.445

5. 27.56 + 14.69
Solution: 42.25

⇒ \(\begin{aligned}
& 27.56 \\
& 14.69 \\
& \hline 42.25 \\
& \hline
\end{aligned}\)

6. 4.3 + 3 – 6.4
Solution: 7.3 – 6.4 = 0.9

= 11.3-6.4 = 0.0

7. 3.36 – 4.62 + 2.18
Solution: 3.36 + 2.18- 4.62

= 5.54 – 4.62 = 0.92

8. 2.67 – 3.727 + 4.2.
Solution: 6.870 – 3.727 = 3.143

Fraction Exercise 1.6

Question 1. Let us try to place the following angles under right heading: 12°, 22.5°, 180°, 179°, 100°,39°,90°,69°,910
Solution:

Actute angles: 12°, 22.5°, 39°, 69°

Obtuse angles: 179°, 100°, 91°

Right angles: 90°

Straight angles: 180°

Question 2. Straight angle = 2 x □, hence straight angle is twice a right angle.
Solution:

Straight angle = 180° = 2 x 90° = 2 x right angle.

Question 3. Let’s find with which set of the lengths of line segments, triangles can be drawn:

1. 2 cm, 3 cm, 4 cm,
Solution:

Sum of 2 smaller sides = 2 cm + 3 cm = 5 cm is greater than the 3rd side (4 cm).

∴ Triangle can be drawn.

2. 4 cm, 3 cm, 7 cm
Solution:

Here sum of 2 smaller sides = 4 cm + 3cm = 7 cm is equal to the 3rd side (7 cm).

∴ Triangle can not be drawn.

3. 1 cm, 3 cm, 2 cm
Solution:

Here sum of 2 smaller sides = 1 cm + 2 cm= 3 cm is equal to the 3rd side (3 cm).

∴ Triangle can not be drawn.

4. □ cm, □ cm, □ cm.
Solution:

6 cm, 8 cm, 10 cm (let)

Here sum of 2 smaller sides = 6 cm + 8 cm = 14 cm is greater than the 3rd side (10 cm).

∴ Triangle can be drawn.

Question 4. Let us define actute angled and obtuse angled triangles.
Solution:

Acute angled triangle: A triangle whose three angles are acute, i.e., less than 90° is called an acute angled triangle.

Obtuse angled triangle: A triangle whose one angle is obtuse, i.e., greater than 90° but less than 180° is called obtuse angled triangle.

Question 5. Let’s measure the perimeter of the square in the figure.
Solution:

Side = 4 cm

Perimeter = 4 x side

= 4 x 4 cm

= 16 cm

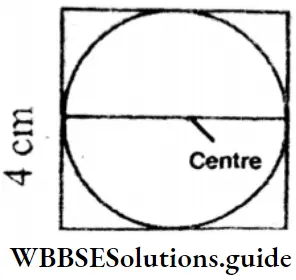
Question 6. The diameter of circle in the figure = □ cm. Radius = □□□ cm = □ cm.
Solution:

Diameter = 4 cm

Radius = 4 ÷ 2 cm = 2 cm.

Fraction Exercise 1.7

Question 1. To construct an embankment on a certain part of river Ichhamati, 40 labourers are required to work for 35 days. If the work has to be finished in 28 days, let us find how many labourers will be needed.
Solution:

Given

To construct an embankment on a certain part of river Ichhamati, 40 labourers are required to work for 35 days. If the work has to be finished in 28 days

To finish the work:

⇒ \(\begin{array}{ll}
\text { No. of days } & \text { No. of Labourers } \\
35 & 40 \\
28 & x \text { (let) }
\end{array}\)

Number of days is inversely proportional to number of labourers.

∴ \(\frac{40}{x}\) = \(\frac{28}{35}\)

∴ 28 x = 40 x 35

X = \(\frac{40 \times 35}{28}\) = 50

∴ Number of labourers = 50

OR

To construct an embarkment.

To construct an embarkment in 35 days, number of labourers required 40

To construct an embarkment in 1 day number of labourers required is 40×35

To construct an embarkment in 28 days number of labourers required \(\frac{40 \times 35}{28}\) = 50

∴ Number of labourers required = 50

Question 2. Rajeeb, Debangana, Masum and Tajmira can do 150 sums in 6 days. Let us find in how many days Rajeeb and Tajmira will finish 250 sums if each of them does equal number of sums everyday.
Solution:

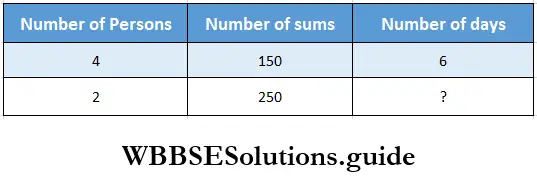
4 persons can do 150 sums in 6 days.

1 person can do 150 sums in 6 x 4 days

1 persons can do 1 sum in \(\frac{6 \times 4}{150}\) days

2 persons can do 1 sum in \(\frac{6 \times 4}{150 \times 2}\) days.

2 persons can do 250 sums in \(\frac{6 \times 4 \times 250}{150 \times 2}\) = 20 days.

∴ Number of days required = 20.

Question 3. Two labourers can polish 1/3 of a door in 1 day. Let us find how many labourers are needed to polish 2/3 of a door in 2 days.
Solution:

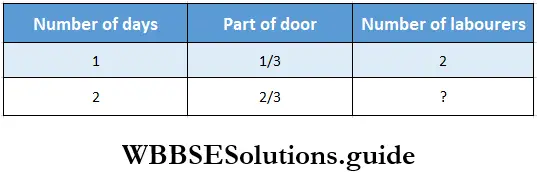
In 1 day \(\frac{1}{3}\) part of the door is polished by 2 labourers.

In 1 day full part of the door is polished by 2 x 3 labourers.

In 2 days full part of the door is polished by \(\frac{2 \times 3}{2}\) labourers.

In 2 days \(\frac{2}{3}\) part of the door is polished by \(\frac{2 \times 3}{2} \times \frac{2}{3}\) labourers = 2 labourers.

∴ Number of labourers required = 2

Question 4. For a mid-day meal of 500 students, 175 kg of rice is required for a week. After 75 kg of rice has been used, let us find how long remaining rice will last for 400 students.
Solution:

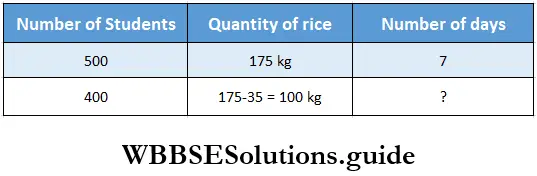
500 students can eat 175 kg rice in 7 days.

1 student can eat 175 kg rice in 7 x 500 days.

1 student can eat 1 kg rice in \(\frac{7 \times 500}{175}\) days.

400 student can eat 1 kg rice \(\frac{7 \times 500}{400 \times 175}\) days

400 student can eat 100 kg rice in \(\frac{7 \times 500 \times 400}{400 \times 175}\) =5 days.

∴ Number of days required = 5.

Question 5. 4 tractors are required to cultivate 360 bighas of land in 20 days. Let us find how many tractors are needed to cultivate 1800 bighas of land in 10 days.
Solution:

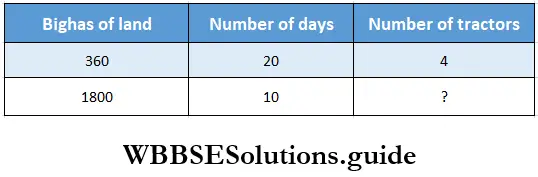
360 bighas of land are cultivated in 20 days by 4 tractors.

1 bigha of land are cultivated in 1 days by \(\frac{4}{360}\) tractors.

1 bigha of land are cultivated in 1 day by \(\frac{4 \times 20}{360}\) tractors.

180 bighas of land are cultivated in 1 day by \(\frac{4 \times 20 \times 1800}{360}\)

1800 bighas of land are cultivated in 10 days by \(\frac{4 \times 20 \times 1800}{360 \times 10}\)=40 tractors.

∴ Number of tractors required is 40.

Question 6. In a fair, if 12 generators work for 6 hours a day, then 7 litres of deposited oil is required. If generators run for 4 hours a day for 9 days, then let us find how many generators can run with that remaining amount of oil.
Solution:

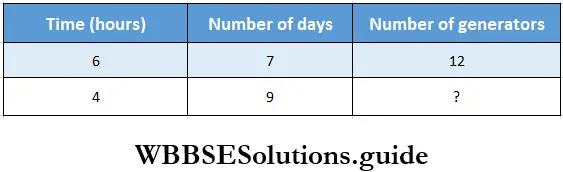
For 6 hours a day in 7 days oil deposited for 12 generators.

For 6 hours a day in 1 day oil deposited for 12×7 generators.

For 1 hour a day in 1 day oil deposited for 12 x 7 x 6 generators.

For 4 hours a days in 1-day oil deposited for \(\frac{12 \times 7 \times 6}{4}\) generators.

For 4 hours a day in 9 days oil deposited for \(\frac{12 \times 7 \times 6}{9 \times 4}\) =14 generators.

∴ 14 generators can run with that remaining amount of oil.

Question 7. 15 Vans can carry 75 quintals of vegetables in 40 mins. Let us find out how long will 20 vans take to carry 100 quintals of vegetables.
Solution:

15 vans can carry 75 quintals of vegetables in 40 minutes.

1 van can carry 75 quintals of vegetables in 40 x 15 minutes.

1 van can carry 100 quintals of vegetables in \(\frac{40 \times 15}{75}\)minutes.

20 vans can carry 100 quintals of vegetables in \(\frac{40 \times 15 \times 100}{20 \times 75}\) minutes

∴ Time required = 20 minutes.

Question 8. 150 kg of wheat is stored for 20 hostel boys. But 30 kg wheat was wasted and 5 boys went home. With the remaining amount of wheat, let us find for how long the remaining boys be fed.
Solution:

150 kg of wheat is stored for 20 boys for 30 days.

150 kg of wheat is stored for 1 boy for 30 x 20 days.

1 kg of wheat is stored for 1 boy for \(\frac{30 \times 20}{150}\) days.

120 kg of wheat is stored for 1 boy for \(\frac{30 \times 20 \times 120}{150}\) days.

120 kg of wheat is stored for 15 boys for \(\frac{30 \times 20 \times 120}{15 \times 150}\) =32 days.