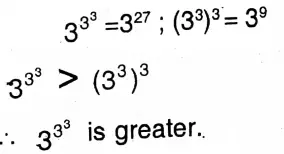WBBSE Solutions For Class 9 Maths Chapter 2 Laws Of Indices

Ganit Prabha Class 9 Solutions Chapter 2 Laws Of Indices

Important Formula:

1. x^m.xⁿ = x^m+n

2. \(x^m \div x^n=\frac{x^m}{x^n}=x^{m-n}\)

3. (x^m)ⁿ = x^mn

4. (xy)^m = x^my^m

5. \(\left(\frac{x}{y}\right)^m=\frac{x^m}{y^m}\)

6. \(\frac{1}{x^{-m}}=x^m\)

7. x⁰=1

8. \(x^{-1}=\frac{1}{x}\)

9. \(x^{-n}=\frac{1}{x^n}\)

10. x^m=Yⁿ ,n ≠ 0 ⇒ x= y when x and y positive

11. x^m=Yⁿ  ⇒ m = n when x>n and x ≠1

12. x^m = y ∴ \(x=y^{\frac{1}{m}}\)

Read and Learn More WBBSE Solutions For Class 9 Maths

Class 9 Math Solution West Bengal Board Chapter 2 Laws Of Indices: Exercise 2.1

 

Question 1. Let us find out the values of the following:

1. \((\sqrt[5]{8})^{\frac{5}{2}} \times\left(16^{\frac{-3}{2}}\right)\)

Solution:

Given

 

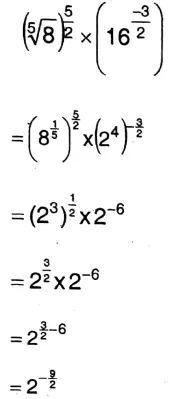
2. \(\left\{(125)^{-2} \times(16)^{\frac{-3}{2}}\right\}^{\frac{-1}{6}}\)

Solution:

Given

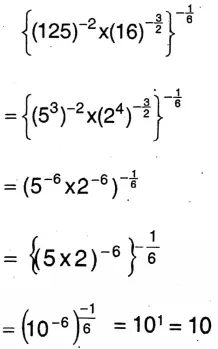
WBBSE Class 9 Math Book Solution

3. \(4^{\frac{1}{3}} \times\left[2^{\frac{1}{3}} \times 3^{\frac{1}{2}}\right] \div 9^{\frac{1}{4}}\)

Solution: \(4^{\frac{1}{3}} \times\left[2^{\frac{1}{3}} \times 3^{\frac{1}{2}}\right] \div 9^{\frac{1}{4}}\)

 

 

 

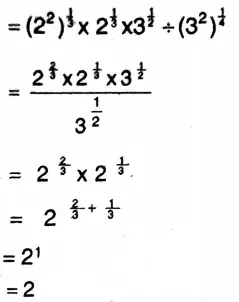
Question 2. Let us simplify:

1. \(\left(8 a^3 \div 27 x^{-3}\right)^{\frac{2}{3}} \times\left(64 a^3 \div 27 x^{-3}\right)^{-2}\)

Solution:

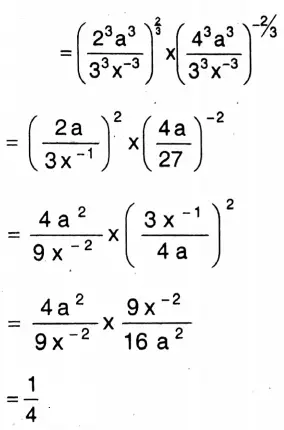
WBBSE Class 9 Math Book Solution

2. \(\left\{\left(x^{-5}\right)^{\frac{2}{3}}\right\}^{\frac{-3}{10}}\)

Solution:

 

 

3. \(\left[\left\{\left(2^{-1}\right)^{-1}\right\}^{-1}\right]^{-1}\)

Solution:

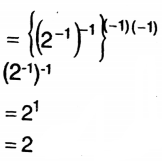
WB Board Class 9 Math Solution

4. \(\sqrt[3]{a^{-2}} \cdot b \times \sqrt[3]{b^{-2}} \cdot c \times \sqrt[3]{c^{-2}} \cdot a\)

Solution:

 

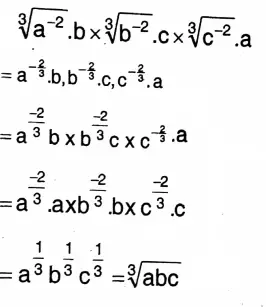
5. \(\left(\frac{4^{m+\frac{1}{4}} \times \sqrt{2.2^m}}{2 \cdot \sqrt{2^{-m}}}\right)^{\frac{1}{m}}\)

Solution:

 

West Bengal Class 9 Maths Solutions

\(\left(2^{\frac{5 m+2-2+m}{2}}\right)^{\frac{1}{m}}\)

\(=\left(2^{\frac{6 m}{2}}\right)^{\frac{1}{m}}=2^3=8\)

 

6. \(9^{-3} \times \frac{16^{\frac{1}{4}}}{6^{-2}} \times\left(\frac{1}{27}\right)^{-\frac{4}{3}}\)

Solution:

West Bengal Class 9 Maths Solutions

7. \(\left(\frac{x^a}{x^b}\right)^{a^2+a b+b^2} \times\left(\frac{x^b}{x^c}\right)^{b^2+b c+c^2} \times\left(\frac{x^c}{x^a}\right)^{c^2+c a+a^2}\)

Solution:

 

West Bengal Class 9 Maths Solutions

Question 3. Let us arrange in ascending order:

1. \(5^{\frac{1}{2}}, 10^{\frac{1}{4}}, 6^{\frac{1}{3}}\)

Solution:

 

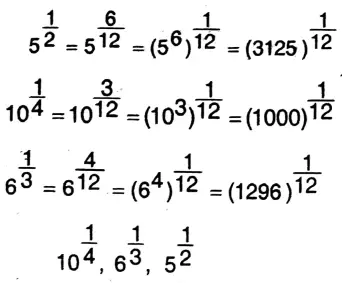
Class 9 Math Solution West Bengal Board

2. \(3^{\frac{1}{3}}, 2^{\frac{1}{2}}, 8^{\frac{1}{4}}\)

Solution:

 

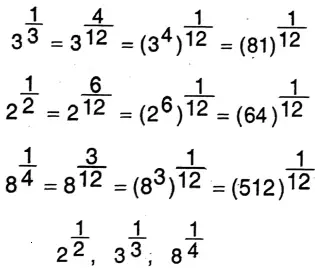
3. \(2^{60}, 3^{48}, 4^{36}, 5^{24}\)

Solution:

Class 9 Math Solution West Bengal Board

Question 4. Let us prove:

1. \(\left(\frac{a^q}{a^r}\right)^p \times\left(\frac{a^r}{a^p}\right)^q \times\left(\frac{a^p}{a^q}\right)^r=1\)

Solution: L.H.S\(\left(\frac{a^q}{a^r}\right)^p \times\left(\frac{a^r}{a^p}\right)^q \times\left(\frac{a^p}{a^q}\right)^r\)

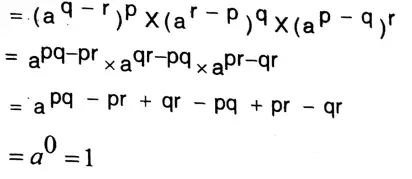
Ganit Prabha Class 9 Solutions

= R.H.S Proved.

2. \(\left(\frac{x^m}{x^n}\right)^{m+n} \times\left(\frac{x^n}{x^1}\right)^{n+1} \times\left(\frac{x^1}{x^m}\right)^{1+m}\)

Solution:

L.H.S = \(=\left(\frac{x^m}{x^n}\right)^{m+n} \times\left(\frac{x^n}{x^{\prime}}\right)^{n+1} \times\left(\frac{x^{\prime}}{x^m}\right)^{l+m}\)

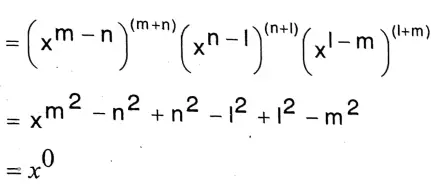
= 1 = R.H.S Proved.

3. \(\left(\frac{\mathbf{x}^m}{\mathbf{x}^n}\right)^{m+n-1} \times\left(\frac{\mathbf{x}^n}{\mathbf{x}^1}\right)^{n+1-m} \times\left(\frac{\mathbf{x}^{\prime}}{\mathbf{x}^m}\right)^{1+m-n}=1\)

Solution: L.H.S \(=\left(\frac{x^m}{x^n}\right)^{m+n-1} \times\left(\frac{x^n}{x^{\prime}}\right)^{n+1-m} \times\left(\frac{x^{\prime}}{x^m}\right)^{1+m-n}\)

 

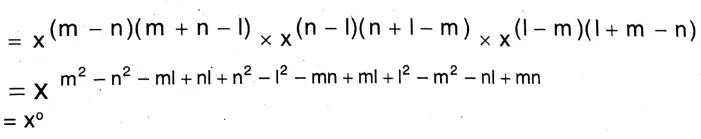
=1= R.H.S Proved.

4. \(\left(a^{\frac{1}{x-y}}\right)^{\frac{1}{x-z}} \times\left(a^{\frac{1}{y-z}}\right)^{\frac{1}{y-x}} \times\left(a^{\frac{1}{z-x}}\right)^{\frac{1}{z-y}}=1\)

Solution: L.H.S \(=\left(a^{\frac{1}{x-y}}\right)^{\frac{1}{x-z}} \times\left(a^{\frac{1}{y-x}}\right)^{\frac{1}{y-x}} \times\left(a^{\frac{1}{z-x}}\right)^{\frac{1}{z-y}}\)

\(=a \frac{1}{(x-y)(x-z)}+\frac{1}{(y-z)(y-x)}+\frac{1}{(z-x)(z-y)}\)

 

Ganit Prabha Class 9 Solutions

 

Question 5. If x+z=2y and b²=ac, then let us show that a ^y-z b ^z-x c^x-y = 1.

Solution:

 

 

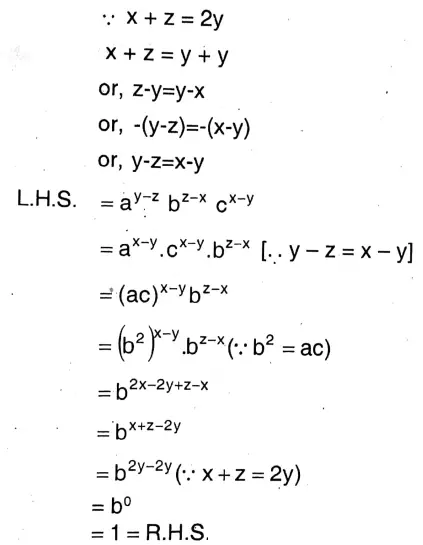
Question 6. If a = xy^p-1, b = xy^q-1 ,and c = xy^r-1, then let us show that a ^q-r b ^r-p  c^p-q=1.

Solution: L.H.S. a ^q-r b ^r-p  c^p-q

=1 R.H.S Proved.

Question 7. If \(x^{\frac{1}{a}}=y^{\frac{1}{b}}=z^{\frac{1}{c}}\) and =xyz=1, then let us show that a+b+c =0.

Solution: \(x^{\frac{1}{a}}=y^{\frac{1}{b}}=z^{\frac{1}{c}}\)=k(let)

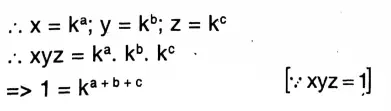
or, a ^q-r b ^r-p  c^p-q=1 k^a+b+c= k^o [ k⁰=1]
or, a+b+c=0 Proved.

Question 8. If a^x = b^y = c^z and abc = 1 then let us show that xy+yz+zx=0.

Solution:

Question 9. Let us solve:

1. 49^x=7³

Solution: 49^x=7³

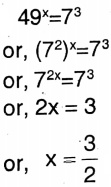
2. 2^x+2+2^x-1=9

Solution: 2^x+2+2^x-1=9

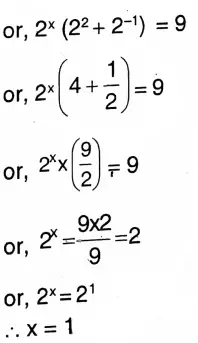
Ganit Prabha Class 9 Solutions

3. 2^x+1+ 2^x+2= 48

Solution: 2^x+1+ 2^x+2= 48

\(or, 2^x=\frac{48}{6}
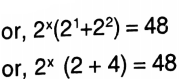
or, 2^x=8=2^3\)

∴x = 3

4. \(2^{4 x} \cdot 4^{3 x-1}=\frac{4^{2 x}}{2^{3 x}}\)

Solution:

 

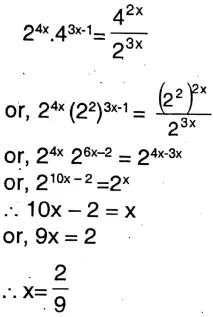
5. 9Χ81^x = 27^2-x

Solution:

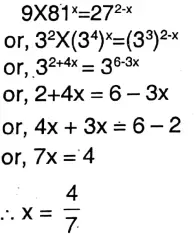
Ganit Prabha Class 9 Solutions

6. 2^5x+4 + 2⁹ = 2¹⁰

Solution: 2^5x+4 + 2⁹ = 2¹⁰

Class 9 Math Solution WBBSE

7. 6^2x+4=3^3x 2^x+8

Solution:

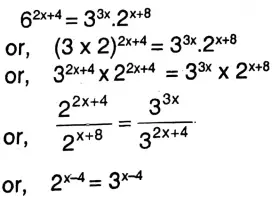
\(\text { or, }\left(\frac{2}{3}\right)^{x-4}=\left(\frac{2}{3}\right)^0\)

or, x-4=0
∴ x = 4 

Question 10. 

1. The value of (0.243)^0.2 x (10)^0.6 is

(1)0.3
(2)3
(3)0.9
(4)9

Solution: (0.243)^0.2 x (10)^0.6

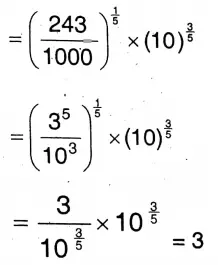
∴ (2)3

2. The value of \(2^{\frac{1}{2}} \times 2^{-\frac{1}{2}} \times(16)^{\frac{1}{2}}\) is

(1) 1
(2) 2
(3) 4
(4)1/2

Solution:\(2^{\frac{1}{2}} \times 2^{-\frac{1}{2}} \times(16)^{\frac{1}{2}}\)

Class 9 Math Solution WBBSE

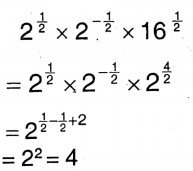
∴(3) 4

3. If 4^x=8³, then the value of x is

(1)3/2
(2)9/2
(3)3
(4)9

Solution: 4^x=8³
or, 2^2x=2⁹
∴ x=9/2

∴ (2)9/2

4. If 20^-x= 1/7 then the value of (20)^2x is
(1) 1/49
(2)7
(3)49
(4)1

Solution: 20^-x= 1/7

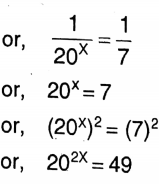
∴ (3)49

5. If 4 x 5^x = 500 then the value of x^x is
(1) 8
(2)1
(3)64
(4)27

Solution: 4 x 5^x = 500

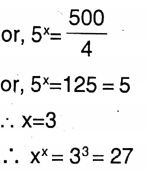
∴ (4)27

Question 11. Short answer type questions:

1. If (27)^x = (81)^y, then let us write the ratio x: y.

Solution: (27)^x = (81)^y
or, 33^x=34^y
or, 3x=4y

or, \(\frac{x}{y}=\frac{4}{3}\)

= x:y 4:3

2. If (5⁵+0.01)²– (55-0.01)²=5^x, then let us calculate the value of x and write it.

Solution: (5⁵+0.01)²– (55-0.01)²=5^x
or, 4 x 5⁵x 0.01= 5^x

or,\(4 \cdot 5^5 \cdot \frac{1}{100}=5^x\)

or, \(5^5 \times \frac{1}{25}=5^x\)

Class 9 Math Solution WBBSE

or, 5³ =5^x
∴ x = 3 .

3. If 3 x 27^x=9^x+4, then let us calculate the value of x and write it.

Solution: 3 x 27^x=9^x+4
or, 3 x (3³)=(3²)^x+4
or, 3 x 3^3x=3^2x+8
or, 3^{1+ 3x} = 3^2x+8
or,3x-2x=8-1
∴ x = 7

4. Let us find out the value of \(\sqrt[3]{\left(\frac{1}{64}\right)^{\frac{1}{2}}}\)and write it.

Solution:

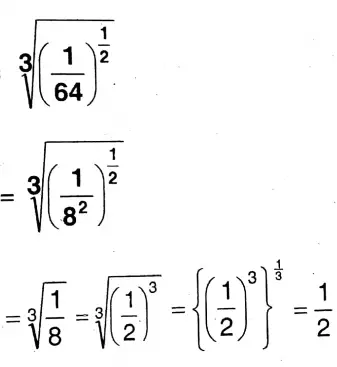
5. Let us write explaining the greater value between \(3^{3^3} \text { and }\left(3^3\right)^3\)with reason.

Solution: