WBBSE Solutions For Class 9 Maths Chapter 4 Co-ordinate Geometry Distance Formula

Class IX Maths Solutions WBBSE Chapter 4 Co-ordinate Geometry: Distance Formula Let Us Work Out

Question 1. I measure the length of the straight line joining the following pairs of points

1. (18, 0); (8, 0)

Solution: Let P and Q be two points whose coordinates are (18,0) and (8,0). Points P and Q are situated in the positive direction of the x-axis.

∴ \(\overline{\mathrm{PQ}}=(\overline{\mathrm{OP}}-\overline{\mathrm{OQ}})\) = (18 – 8) unit = 10 units.

Read and Learn More WBBSE Solutions For Class 9 Maths

 

 

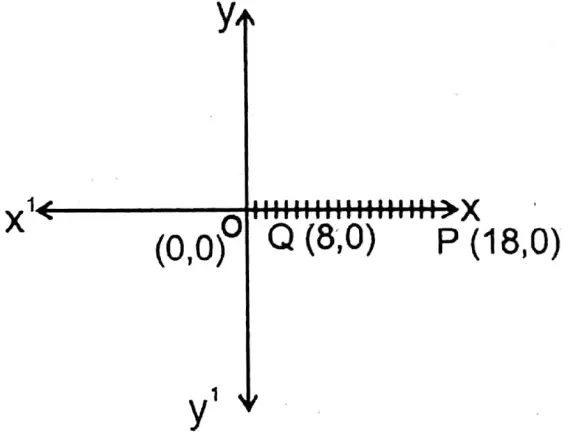
∴ The length of the straight line \(\overline{\mathrm{PQ}}\) = 10 units.

∴ Length of the line segment by joining two points is 10 units.

2. (0, 15); (0, 4)

Soltion: Let P and Q are two points whose coordinates are (0, 15) and (0, 4). P and Q are situated in the positive direction of the y-axis.

∴ \(\overline{\mathrm{OP}}\) =15 unit

∴ \(\overline{\mathrm{OQ}}\)= 4 unit

 

 

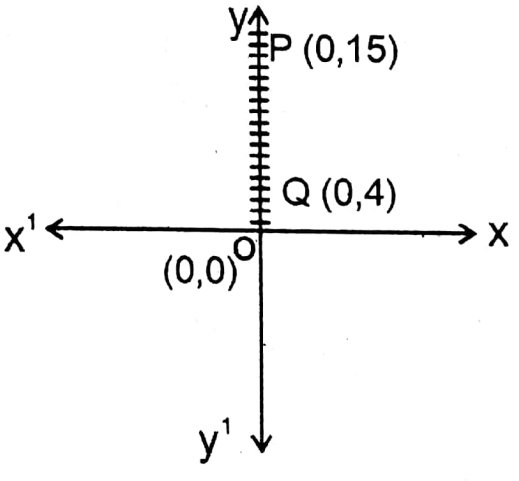
∴ \(\overline{\mathrm{PQ}}=(\overline{\mathrm{OP}}-\overline{\mathrm{OQ}}))\) = (15 – 4) units = 11 units.

∴ The length of the straight line PQ is 11 units.
∴ The length of the line segment formed by joining the two points is 11 units.

3. (-7, 0) (-2, 0)

Solution: Let P and Q are two points whose coordinates are (-7, 0) and (-2, 0). Points P and Q are situated in the negative direction of the x-axis.

∴ \(\overline{\mathrm{OP}}\) = 7 units

∴\(\overline{\mathrm{OQ}}\)= 2 units

 

 

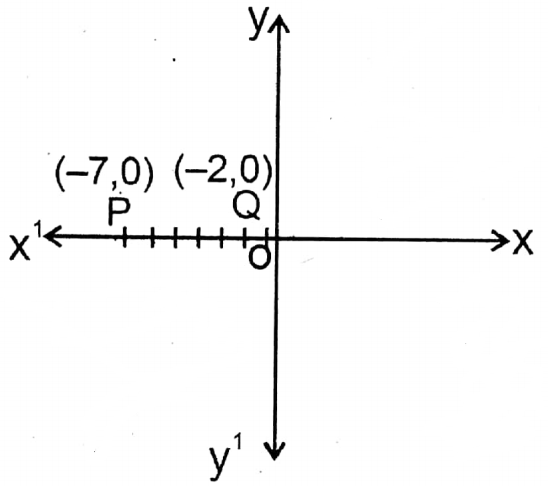
∴ \(\overline{\mathrm{PQ}}=\overline{\mathrm{QP}}=\overline{\mathrm{OP}}-\overline{\mathrm{OQ}}\) = (7-2) units = 5 units

∴ Length of straight line PQ is 5 units.
∴ The length of the straight line formed by joining the two points is 5 units.

Class 9 Mathematics West Bengal Board

4. (0, -10) (0, -3)

Solution: Let P and Q are two points whose coordinates are: (0, -10) and (0, -3).

∴ \(\overline{\mathrm{OP}}\) = 10 units

∴ \(\overline{\mathrm{OQ}}OQ\) = 3 units

 

 

 

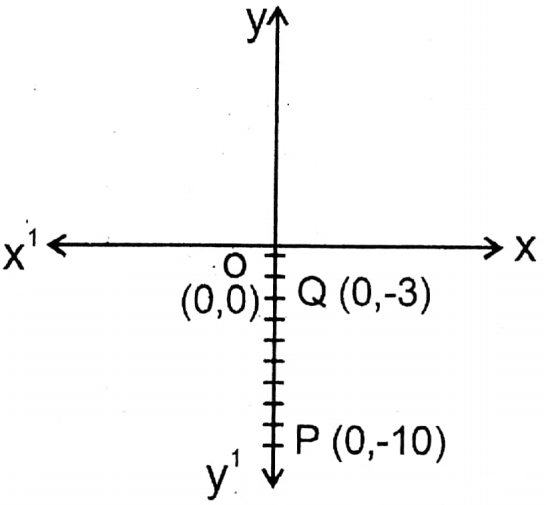
\(\overline{\dot{\mathrm{PQ}}}=\overline{\mathrm{QP}}=\overline{\mathrm{OP}}-\overline{\mathrm{OQ}}\) = (10 – 3) units = 7 units.
∴ Length of straight line PQ is 7 units.
∴ The length of the line segment formed by joining the two points is 7 units.

5. (6, 0) (-2, 0)

Solution: Let P and Q are two points whose coordinates are: (6, 0) and (-2, 0). Point P is situated in the positive direction and point Q is situated in the negative direction of x-axis.

∴ \(\overline{\mathrm{OP}}\) = 6 units

∴ \(\overline{\mathrm{OQ}}\) = 2 units

 

 

\(\overline{\mathrm{PQ}}=\overline{\mathrm{QP}}=(\overline{\mathrm{OP}}+\overline{\mathrm{OQ}})\) = (6 + 2) units = 8 units.

∴ Length of straight line PQ is 8 units.
∴ The length of the line segment formed by joining the two points is 8 units.

Class 9 Mathematics West Bengal Board

6. (0,-5) (0, 9)

Solution: Let P and Q are two points whose co-ordinates are: (0, -5) and (0, 9). Point P is situated in the negative direction and point Q is situated in the positive direction of y-axis.

∴ \(\overline{\mathrm{OP}}\) = 5 units

∴ \(\overline{\mathrm{OQ}}OQ\)=9 units

 

 

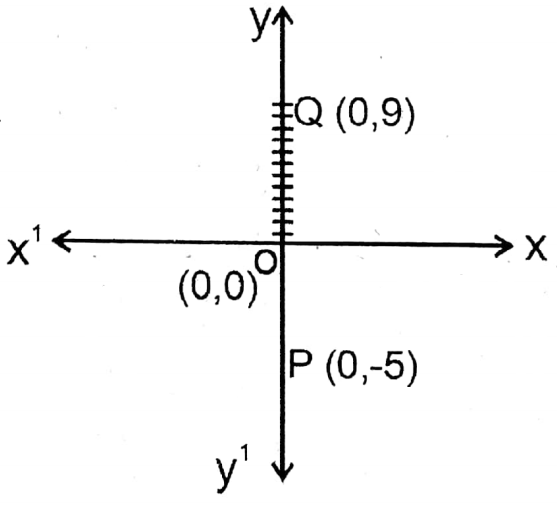
∴ \(\overline{\mathrm{PQ}}=\overline{\mathrm{QP}}=(\overline{\mathrm{OP}}+\overline{\mathrm{OQ}})\) = (5+9) units = 14 units.

∴ Length of the straight line PQ is 14 units.
∴ The length of the line segment formed by joining the two points is 14 units.

7. (5, 0): (0, 10)

Solution: Let P and Q are two points whose coordinates are (5, 0) and (0, 10)
∴OP = 5 units
OQ = 10 units

 

 

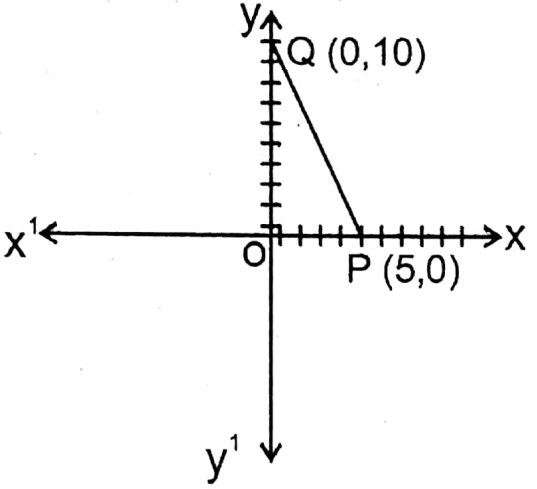
In right angled ΔPOQ from Pythagoras’ theorem,

PQ² = OP² + OQ²
or, PQ² = (5)² + (10)² sq.units
or, PQ² = 25+ 100 sq.units
or, PQ² = 125 sq.units

∴ PQ= √125 units = 5√5 units
∴ Distance between points P and Q is 5√5 units.
∴ The length of the line segment formed by joining the two points is 5√5 units.

Class 9 Mathematics West Bengal Board

8. (3,0): (0, 4)

Solution: Let P and Q be two points whose co-ordinates are (3, 0) and (0, 4).

∴ OP = 3 units
OQ = 4 units

 

 

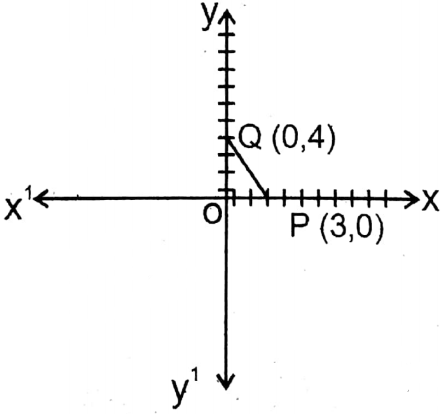
In right-angled APOQ we get by Pythagoras’ theorem,

PQ² = OP²+OQ²
or, PQ² = (3)² + (4)² sq.units
or, PQ² = 9 + 16 sq.units
or, PQ² = 25 sq.units

∴ PQ= √25 units = 5 units
∴ Distance between points P and Q is 5 units.
Length of the straight line formed by joining the two points is 5 units.

Class 9 Maths WB Board

9. (4,3); (2, 1)

Solution: Let A and B are two points whose co-ordinates are (2, 1) and (4, 3).
In Image, OM = 2 units and AM = 1 units.
ON 4 units and BN = 3 units.

∴ AP = MN = ON-OM = (4-2) units = 2 units
Again, BP= BN-PN = (3-1) units = 2 units

 

 

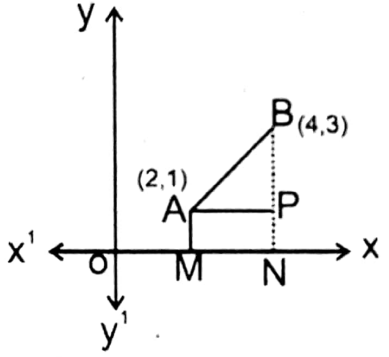
In right angled Δ APB we get by Pythagoras’ theorem,

AB² = AP² + BP²
or, AB² = (2)² + (2)² sq.units
or, AB² = 4+4 sq.units
or, AB² = 8 sq.units

∴ AB = √8 units = 2√2 units
∴ Distance between the points A and B, i.e., length of the straight line formed by lining the two points is 2√2 units.

Class 9 Maths WB Board

10. (-2,-2) (2, 2)

Solution: Let A and B are two points whose co-ordinates are (-2, -2) and (2, 2).
∴ A point lies in third quadrant. B lies in first quadrant. From points A and B on axis respectively AP and BQ perpendiculars are drawn which cut x-axis at points p and Q respectively.
∴ OP 2 units and OQ = 2 units.
BQ 2 units and AP 2 units

 

 

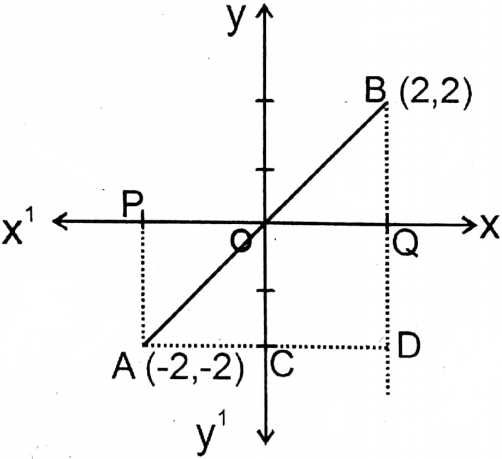
From point A on extended-BQ a perpendicular is drawn which cuts extended BQ at point D.
∴ AD = PQ = OP + OQ
(2+2) units = 4 units
BD = BQ + QD = BQ + PA (2+2) units = 4 units

In right angled AADB we get by Pythagoras’ theorem, AB2

AD²+ BD²
or, AB² = (4)²+(4)² sq.units
or, AB² = 16+ 16 sq.units
or, AB² = 32 sq.units

∴ AB = √32 units = 4√√2 units
∴ Length of the straight line AB is 4√2 units, i.e., the length of the straight line formed by joining the two points is 4√2 units.

 

Class 9 Mathematics West Bengal Board Chapter 4 Co-ordinate Geometry: Distance Formula Exercise 4.1

 

Question 1. Let us calculate the distances of the following points from the origin

Here, distance formula = \(\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2}\)unit.

1. (7, -24)

Solution: The distance of the point (7,-24) from the origin is

\(\begin{aligned}
D & =\sqrt{(7-0)^2+(-24-0)^2} \text { unit } \\
& =\sqrt{49+576} \text { unit } \\
& =\sqrt{625} \text { unit } \\
& =25 \text { unit }
\end{aligned}\)

 

2. (3,-4)

Solution:The distance of the point (3, 4) from the origin (0,0) is

\(\begin{aligned}
D & =\sqrt{(3-0)^2+(-4-0)^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

3. (a+b, a-b)

Solution: The distance of the point (a+b, a-b) from the origin (0,0) is

\(\begin{aligned}
& D=\sqrt{(a+b-0)^2+(a-b-0)^2} \text { unit } \\
& =\sqrt{(a+b)^2+(a-b)^2} \text { unit } \\
& =\sqrt{2\left(a^2+b^2\right)} \text { unit }
\end{aligned}\)

 

Question 2. Let us calculate the distances between the pairs of points given below:

1. (5, 7) and (8, 3)

Solution: The distance between (5, 7) and (8, 3) is

\(\begin{aligned}
& =\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2} \text { unit } \\
& D=\sqrt{(5-8)^2+(7-3)^2} \text { unit } \\
& =\sqrt{-3^2+4^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

2. (7,0) and (2,-12)

Solution: Distance between (7, 0) and (2,-12) is

\(\begin{aligned}
& =\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2} \text { unit } \\
& D=\sqrt{(5-8)^2+(7-3)^2} \text { unit } \\
& =\sqrt{-3^2+4^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

 

3. \(\left(-\frac{3}{2}, 0\right)\) and (0, -2)

Solution: Distance between \(\left(-\frac{3}{2}, 0\right)\) and (0, -2)

 

\(=\sqrt{\left(\frac{-3}{2}-0\right)^2+(0+2)^2} \text { unit }\)

 

\(\begin{aligned}
& =\sqrt{\frac{9}{4}+4} \text { unit } \\
& =\sqrt{\frac{9+16}{4}} \text { unit } \\
& =\sqrt{\frac{25}{4}} \text { unit } \\
& =\frac{5}{2} \text { unit } \\
& =2.5 \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

4. (3, 6) and (-2, -6)

Solution: Distance between (3, 6) and (-2, -6) is

\(\begin{aligned}
& D=\sqrt{(3+2)^2+(6+6)^2} \text { unit } \\
& =\sqrt{25+144} \text { unit } \\
& =\sqrt{169} \text { unit } \\
& =13 \text { unit }
\end{aligned}\)

 

5. (1,-3) and (8,3)

Solution: Distance between (1,-3) and (8,3) is

\(\begin{aligned}
& =\sqrt{(1-8)^2+(-3-3)^2} \text { unit } \\
& =\sqrt{7^2+6^2} \text { unit } \\
& =\sqrt{49+36} \text { unit } \\
& =\sqrt{85} \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

6. (5,7) and (8,3)

Solution: The distance between the points (5, 7) and (8, 3) is

\(\begin{aligned}
D & =\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2} \text { unit } \\
& =\sqrt{(5-8)^2+(7-3)^2} \text { unit } \\
& =\sqrt{3^2+4^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

 

Question 3. Let us prove that the point (-2, -11) is equidistant from the two points (3,7) and (4,6).

Solution: The distance (D₁) between the points (-2, -11) and (-3, 7) is

\(\begin{aligned}
& =\sqrt{(-2+3)^2+(-11-7)^2} \text { unit } \\
& =\sqrt{1^2+(-18)^2} \text { unit } \\
& =\sqrt{1+324} \text { unit } \\
& =\sqrt{325} \text { unit }
\end{aligned}\)

Maths WBBSE Class 10 Solutions

And the distance (D) between the points (-2, -11) and (4, 6) is

\(\begin{aligned}
& =\sqrt{(-2-4)^2+(-11-6)^2} \text { unit } \\
& =\sqrt{(-6)^2+(-17)^2} \text { unit } \\
& =\sqrt{36+289} \text { unit } \\
& =\sqrt{325} \text { unit }
\end{aligned}\)

 

Here, D₁ = D₂
∴ The point (-2, -11) is equidistant to the two points (-3, 7) and (4, 6). Proved

Question 4. By calculation let us show that the points (7, 9), (3, -7), and C (-3, 3) are the vertices of a right-angled triangle.

Solution: Let the points be A = (7, 9), B = (3, -7), and C = (-3, 3).

 

 

\(\begin{aligned}
\text { Distance } A B & =\sqrt{(7-3)^2+(9+7)^2} \text { unit } \\
& =\sqrt{16+256} \text { unit } \\
& =\sqrt{272} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

\(\begin{aligned}
\text { Distance } B C & =\sqrt{(3+3)^2+(-7-3)^2} \text { unit } \\
& =\sqrt{36+100} \text { unit } \\
& =\sqrt{136} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance } A C & =\sqrt{(7+3)^2+(9-3)^2} \text { unit } \\
& =\sqrt{100+36} \text { unit } \\
& =\sqrt{136} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

Here, BC²+ CA² = 136+ 136 = 272 & AB² = 272
∴ BC²+ CA²= AB²
Δ ABC is a right angled triangle, right angle being at ∠C.

Question 5. Let us prove that in both of the following cases, the three points are the vertices of an isosceles triangle:

1. (1, 4), (4, 1), and (8, 8)
   
   Solution: Let A = (1,4); B = (4,1) & C = (8,8)

\(\begin{aligned}
\text { Distance } A B & =\sqrt{(1-4)^2+(4-1)^2} \text { unit } \\
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance AC } & =\sqrt{(1-8)^2+(4-8)^2} \text { unit } \\
& =\sqrt{49+16} \text { unit } \\
& =\sqrt{65} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

\(\begin{aligned}
\text { Distance } B C & =\sqrt{(4-8)^2+(1-8)^2} \text { unit } \\
& =\sqrt{16+49} \text { unit }
\end{aligned}\)

∴ In ΔABC AC= BC
∴ ABC is an isosceles triangle. Proved

2. (-2,-2), (2, 2) and (4,-4)

Solution:Let P= (-2,-2), Q=2, 2) and R = (4,-4)

\(\begin{aligned}
\text { Distance } P Q & =\sqrt{(-2-2)^2+(-2-2)^2} \text { unit } \\
& =\sqrt{4^2+4^2} \text { unit } \\
& =\sqrt{16+16} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance } Q R & =\sqrt{(2-4)^2+(2+4)^2} \text { unit } \\
& =\sqrt{(2)^2+(6)^2} \text { unit } \\
& =\sqrt{4+36} \text { unit } \\
& =\sqrt{40} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance PR } & =\sqrt{(-2-4)^2+(-2+4)^2} \text { unit } \\
& =\sqrt{(-6)^2+(2)^2} \text { unit } \\
& =\sqrt{36+4} \text { unit } \\
& =\sqrt{40} \text { unit }
\end{aligned}\)

WBBSE Solution Class 10 Maths

∴ In triangle PQR, QR = PR= √40
∴ ΔPQR is an isosceles triangle. Proved

Question 6. Let us prove that the three points A (3, 3), B (8,-2), and C (-2,-2) are the vertices of a right-angled triangle. Let us calculate the length of the hypotenuse of ΔABC.

Solution:

Given, A (3,3); B = (8,-2); C = (-2,-2)

AB² = (38)² + (3 + 2)² = 5² +5² = 25+ 25 = 50

AC² = (3 + 2)² + (3 + 2)² = 5² +5² = 25+ 25 =50

BC² = (8+ 2)² + (-2 + 2)² = 10² +0 =100+ 0 = 100

∴ AB²+ AC² = 50+50 = 100∴ Δ ABC is right angled triangle and Hypotenuse BC= √100 = 10 units.

Question 7. Let us show by calculation that the points (2, 1), (0, 0), (-1, 2), and (1, 3) are the angular points of a square.

Solution: A = (2, 1), B = (0, 0), C (-1, 2), and D = (1, 3).

 

 

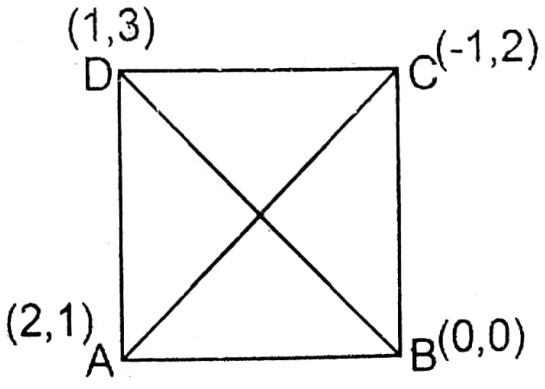
\(Distance A B=\sqrt{(2-0)^2+(1-0)^2}  units =\sqrt{4+1} units =\sqrt{5} units\)

 

\(Distance B C=\sqrt{(0+1)^2+(0-2)^2}  units =\sqrt{1+4} units =\sqrt{5} units\)

Class 10 Math Solution WBBSE Chapter 4

\(\begin{aligned}
\text { Distance CD } & =\sqrt{(-1-1)^2+(2-3)^2} \text { units } \\
& =\sqrt{4+1} \text { units } \\
& =\sqrt{5} \text { units }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance DA } & =\sqrt{(1-2)^2+(3-1)^2} \text { units } \\
& =\sqrt{1+4} \text { units } \\
& =\sqrt{5} \text { units }
\end{aligned}\)

 

\(Again, Diagonal A C=\sqrt{(2+1)^2+(1-2)^2} units =\sqrt{9+1} units =\sqrt{10} units\)

Class 10 Math Solution WBBSE Chapter 4

\(\begin{aligned}
\text { and Diagonal BD } & =\sqrt{(0-1)^2+(0-3)^2} \text { units } \\
& =\sqrt{1+9} \text { units } \\
& =\sqrt{10} \text { units }
\end{aligned}\)

 

∴ In the quadrilateral ABCD all the sides are equal and diagonals AC = BD.
∴ ABCD is a square. Proved

Question 8. Let us calculate and see that for what value of y, the distance between the two points (2,y) and (10, -9) will be 10 units.

Solution:

Given

Distance of the points (2, y) from the point (10, -9)= 10 units.

∴ \(\begin{aligned}
& \sqrt{(2-10)^2+(y+9)^2}=10 \text { (given) } \\
& \sqrt{(-8)^2+(y+9)^2}=10
\end{aligned}\)

Class 10 Math Solution WBBSE Chapter 4

or, 64 +(y+9)²= 100 (Squaring both sides)
or, (y+9) = 100 -64 =36
∴ y+9 = ±6

(1) y+9=6
y=6-9=-3 and

(2) y+9=-6
∴ y-6-9=-15

Question 9. Let us find a point on x-axis which is equidistant from the two points (3,5) and (1,3).

Hints the required point on x-axis is (x,0) = (x-3)² + (0-5)² = (x-1)² + (0-3)²

Solution:

Given

Two points (3,5) and (1,3)

Let the point on the x-axis is (x, 0).

∴ Distance of the point (3, 5) from the point (x,0) is

\(\begin{aligned}
& =\sqrt{(x-3)^2+(0-5)^2} \text { unit } \\
& =\sqrt{(x-3)^2+5^2} \text { unit }
\end{aligned}\)

Class 10 Math Solution WBBSE Chapter 4

and the distance of the point (1,3) from the point (x, 0) is

\(\begin{aligned}
& =\sqrt{(x-1)^2+(0-3)^2} \text { unit } \\
& =\sqrt{(x-1)^2+3^2} \text { unit }
\end{aligned}\)

According to the condition, \(\sqrt{(x-3)^2+25}=\sqrt{(x-1)^2+9}\)

or,(x -3)² + 25 = (x -1 )² +9
or, (3 – x)² – (1 – x) ² = 9 – 25
or, (3-x+1-x) (3-x-1+x)=-16
or, (4-2x) x 2 = -16
or, 2(2 – x) x2 = -16
or, 2 – x = -4
or, 2 + 4 = x
∴ X = 6
∴ The required point is (6,0).

Class 10 Math Solution WBBSE Chapter 4

Question 10. Let us write by calculation whether the three points O(0, 0), A(4, 3), and B (8, 6) are collinear.

Hints: If OA + AB = OB, then they are collinear.

Solution:

Given

O(0, 0), A(4, 3), and B (8, 6)

 

 

\(\begin{aligned}
\text { Diștance } & O A=\sqrt{(0-4)^2+(0-3)^2} \text { unit } \\
= & \sqrt{16+9} \text { unit } \\
= & \sqrt{25} \text { unit } \\
= & 5 \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Distance } O B & =\sqrt{(0-8)^2+(0-6)^2} \text { unit } \\
& =\sqrt{64+36} \text { unit } \\
& =\sqrt{100} \text { unit } \\
& =10 \text { unit }
\end{aligned}\)

Class 9 Maths WB Board

\(\begin{aligned}
\text { Distance } A B & =\sqrt{(4-8)^2+(3-6)^2} \cdot \text { unit } \\
& =\sqrt{16+9} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Here OA+ AB OB, hence they are collinear.

Question 11. Let us show that the three points (2,2), (-2,-2), and (-2√3,2√3) vertices of an equilateral triangle.

Solution: Let A (2,2); B (-2,-2) and C (-2√3, 2√3).

\(\begin{aligned}
Distance A B & =\sqrt{(2+2)^2+(2+2)^2} \text { unit } \\
& =\sqrt{16+16} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

 

\(\text { Distance } B C=\sqrt{(-2+2 \sqrt{3})^2+(-2-2 \sqrt{3})^2} \text { unit }\)

 

\(\begin{aligned}
& =\sqrt{(2 \sqrt{3}-2)^2+(2+2 \sqrt{3})^2} \text { unit } \\
& =\sqrt{2\left\{(2)^2+(2 \sqrt{3})^2\right\}} \text { unit } \\
& =\sqrt{2(4+12)} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

 

\(\text { Distance } B C=\sqrt{(-2+2 \sqrt{3})^2+(-2-2 \sqrt{3})^2} \text { unit }\) \(\begin{aligned}
& =\sqrt{(2 \sqrt{3}-2)^2+(2+2 \sqrt{3})^2 \text { unit }} \\
& =\sqrt{2\left\{(2)^2+(2 \sqrt{3})^2\right\}} \text { unit } \\
& =\sqrt{2(4+12)} \text { unit } \\
& =\sqrt{32} \text { unit }
\end{aligned}\)

∴ AB = BC = CA
∴ A, B, & C are the vertices of an equilateral triangle.

Question 12. Let us show that the points (-7, 2), (19, 8), (15, -6), and (-11, -12) form a parallelogram when they are joined orderly.

Solution: Let A = (-7,2); B = (19,8); C = (15,-6); D = (-11, -12).

 

 

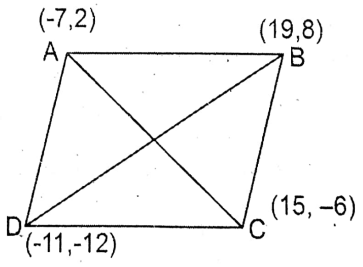
\(The length of A B=\sqrt{(-7-19)^2+(2-8)^2} unit
\begin{aligned}
& =\sqrt{(-26)^2+(-6)^2} \text { unit } \\
& =\sqrt{676+36} \text { unit } \\
& =\sqrt{712} \text { unit }
\end{aligned}
\)

 

\(\begin{aligned}
\text { Length of } B C & =\sqrt{(19-15)^2+(8+6)^2} \text { unit } \\
& =\sqrt{(4)^2+(14)^2} \text { unit } \\
& =\sqrt{16+196} \text { unit } \\
& =\sqrt{212} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Length of } C D & =\sqrt{(15+11)^2+(-6+12)^2} \text { unit } \\
& =\sqrt{(26)^2+(6)^2} \text { unit } \\
& =\sqrt{26^2+6^2} \text { unit } \\
& =\sqrt{676+36} \text { unit }
& = \sqrt{712} \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
\text { Length of } D A & =\sqrt{(-11+7)^2+(-12-2)^2} \text { unit } \\
& =\sqrt{(-4)^2+(14)^2} \text { unit } \\
& =\sqrt{4^2+14^2} \text { unit } \\
& =\sqrt{16+196} \text { unit } \\
& =\sqrt{212} \text { unit }
\end{aligned}\)

 

\(Length of Diagonal A C=\sqrt{(-7-15)^2+(2+6)^2} unit
\begin{aligned}
& =\sqrt{(-22)^2+8^2} \text { unit } \\
& =\sqrt{22^2+8^2} \text { unit } \\
& =\sqrt{484+64} \text { unit }
\end{aligned}\)

Class 9 Maths WB Board

\(\begin{aligned}
\text { Length of Diagonal } B D & =\sqrt{(19+11)^2+(8+12)^2}=\sqrt{(30)^2+(20)^2} \\
& =\sqrt{900+400} \text { unit } \\
& =\sqrt{1300} \text { unit }
\end{aligned}\)

 

In the quadrilateral ABCD, ABCD and BC = DA [opposite sides are equations]
Diagonal AC ≠ Diagonal BD
∴ ABCD is a parallelogram.

Question 13. Let us show that the points (2,-2), (8, 4), (5, 7), and (-1, 1) are the vertices of a rectangle.

Solution: Let P = (2,-2), Q = (8,4); R = (5,7) and S = (-1,1)
To prove the PQRS is a rectangle.

 

 

 

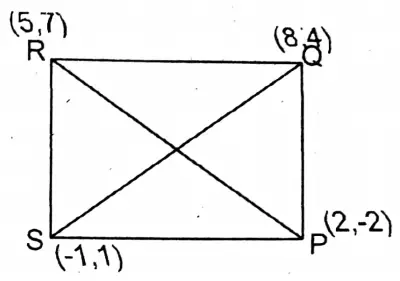
Here, Diagonal PR = Diagonal QS.
∴ In quadrilateral PQRS, opposite sides are equal, and diagonal PR diagonal
∴ PQRS is a rectangle.

Question 14. Let us show that the points (2,5), (5,9), (9,12), and (6,8) form a rhombus when they are joined orderly.

Solution: Let the points A (2, 5); B (5, 9), C (9, 12), and D (6, 8).

 

 

 

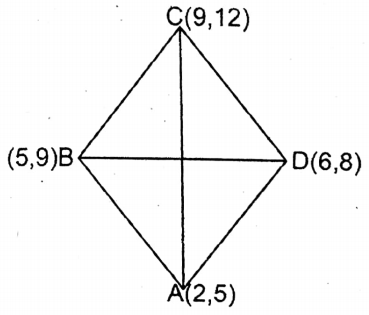
\(\text { Here, } \begin{aligned}
A B= & \sqrt{(2-5)^2+(5-9)^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit }
\end{aligned}\)

Class 9 Maths WB Board

\(\begin{aligned}
& B C=\sqrt{(5-9)^2+(9-12)^2} \text { unit } \\
&=\sqrt{16+9} \text { unit } \\
&=\sqrt{25} \text { unit } \\
&=5 \text { unit }
\end{aligned}\)

 

\(\begin{aligned}
& C D=\sqrt{(9-6)^2+(12-8)^2} \text { unit } \\
& =\sqrt{9+16} \text { unit } \\
& =\sqrt{25} \text { unit } \\
& =5 \text { unit } \\
&
\end{aligned}\)

 

\(\begin{aligned}
& D A=\sqrt{(6-2)^2+(8-5)^2} \\
&=\sqrt{16+9} \text { unit } \\
&=\sqrt{25} \text { unit } \\
&=5 \text { unit }
\end{aligned}\)

 

∴ AB BC CD = DA

\(Again, Diagonal A C=\sqrt{(2-9)^2+(5-12)^2} unit
\begin{aligned}
& =\sqrt{49+49} \text { unit } \\
& =\sqrt{98} \text { unit }
\end{aligned}
\)

 

\(\begin{aligned}
\text { Diagonal BD } & =\sqrt{(5-6)^2+(9-8)^2} \text { unit } \\
& =\sqrt{1+1} \text { unit } \\
& =\sqrt{2} \text { unit }
\end{aligned}\)

∴ Diagonals are not equal.
∴ In quadrilateral ABCD, all the sides are equal but the diagonals are not equal.
∴  ABCD is a rhombus.

Chapter 4 Co-ordinate Geometry Distance Formula  Multiple Choice Questions

1. The distance between the two points (a + b, c-d) and (a-b, c + d) is

(1)\(2 \sqrt{a^2+c^2}\)
(2)\(2 \sqrt{b^2+d^2}\)
(3)\(\sqrt{a^2+c^2}\)
(4)\(\sqrt{b^2+d^2}\)

Solution: The distance between (a + b, c-d) and (a-b, c + d)

\(\begin{aligned}
& =\sqrt{(a+b-a+b)^2+(c-d-c-d)^2} \text { unit } \\
& =\sqrt{(2 b)^2+(-2 d)^2} \text { unit } \\
& =\sqrt{4 b^2+4 d^2} \text { unit } \\
& =2 \sqrt{b^2+d^2} \text { unit }
\end{aligned}\)

∴(2)\(2 \sqrt{b^2+d^2}\)

2. If the distance between the two points (x, -7) and (3, -3) is 5 units, then the values of x are
(1)0 or 6
(2)2 or 3
(3)5 or 1
(4)-6 or 0

Solution: The distance between (x, -7) and (3,-3)

\(=\sqrt{(x-3)^2+(-7+3)^2} \text { unit }\) \(=\sqrt{(x-3)^2+16} \text { unit }\)

 

By the problem, \(\sqrt{(x-3)^2+16}=5\)

or, (x-3)²+16= (5)² (Squaring both sides)
or, (x-3)²=25-16
or, (x-3)² = 9
or, x-3 = ± 3
or, x = 3+3 or x = 3-3
or, x = 6 or x = 0

∴ (1)0 or 6

3. If the distance of the point (x, 4) from the origin is 5 units, then the values of x are
(1)±4
(2)±5
(3)±3
(4)None of these

Solution: The distance of the point (x, 4) from (0,0)

\(\begin{aligned}
& =\sqrt{\mathrm{x}^2+4^2} \text { unit } \\
& =\sqrt{\mathrm{x}^2+16} \text { unit }
\end{aligned}\)

 

By the problem, \(\sqrt{x^2+16}=5\)
or, x²+16= (5)² (Squaring both sides)
or, x²+16=25
or, x² = 25-16
or, x²= 9
or, x = +3

∴ (3)±3

4. The triangle formed by the points (3, 0), (-3, 0), and (0,3) is
(1)Equilateral
(2)Isosceles
(3)Scalene
(4)Isosceles right-angled.

Solution:  Let the co-ordinates of A, B & C be (3,0) (3,0); & (0,3) respectively.

\(\text { Here, } A B=\sqrt{(2-5)^2+(5-9)^2} \text { unit }\) \(=\sqrt{9+16} \text { unit }\) \(=\sqrt{25} \text { unit }\) \(=5 \text { unit }\)

 

\(\begin{aligned}
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit } \\
& =3 \sqrt{2} \text { unit }
\end{aligned}\)

 

\(\text { Length of } B C=\sqrt{(-3-0)^2+(0-3)^2} \text { unit }
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit } \\
& =3 \sqrt{2}\text { unit }
\end{aligned}\)

 

\(\begin{aligned}
A C & =\sqrt{(3-0)^2+(0-3)^2} \text { unit } \\
& =\sqrt{9+9} \text { unit } \\
& =\sqrt{18} \text { unit } \\
& =3 \sqrt{2} \text { unit }
\end{aligned}\)

 

∴ \(B C^2+A C^2=(\sqrt{18})^2+(\sqrt{18})^2\) = 18+ 18 = 36 = 6² = AB²

∴AC = BC and AB² = BC² + AC²
∴ The ΔABC formed by the points (3,0), (-3,0) & (0,3) is an isosceles right-angled triangle.

∴(4)Isosceles right-angled.

5. The co-ordinates of the centre of a circle are (0,0) and the co-ordinates of a point on the circumference are (3,4); the length of the radius of the circle is
(1)5 units
(2)4 units
(3)3 units
(4)None of these.

Solution: Distance between the points (0, 0) and (3, 4) is

\(\begin{aligned}
& =\sqrt{(0-3)^2+(0-4)^2} \text { units } \\
& =\sqrt{9+16} \text { units } \\
& =\sqrt{25} \text { units } \\
& =5 \text { units }
\end{aligned}\)

∴ (1)5 units

Question 16. Short answer type questions:

1. Let us write the value of y if the distance of the point (-4, y) from the origin is 5 units.

Solution:

\(Distance =\sqrt{(-4-0)^2+(0-y)^2} units
\sqrt{16+\mathrm{y}^2}=5 \text { (given) }
\)

 

 or, 16 + y² = 25
∴ y² = 9
∴ y =±3

2. Let us write the co-ordinates of a point on y-axis which is equidistant from the two points (2,3) and (-1,2).

Solution: Let the point on y-axis is (0,b).

\(\begin{aligned}
& =\sqrt{(2-0)^2+(3-b)^2} \\
& =\sqrt{(-1-0)^2+(2-b)^2} \\
& =4+(3-6)^2 \\
& =1+(2-b)^2
\end{aligned}\)

 

∴ b = 4
∴ The point is (0,4).