NEET Physics Gravitation Notes

Gravitation

Kepler’s First Law

All planets revolve around the sun in elliptical orbit, with sun at one of the foci

A → perihelion; B → aphelion

Kepler’s Second Law

The imaginary line which connects the planet and the sun sweeps equal areas in equal intervals of time.

⇒i.e., area of velocity = \(\frac{\Delta \mathrm{A}}{\Delta \mathrm{t}}=\frac{\mathrm{L}}{2 \mathrm{~m}}=\text { constant }\)

Read And Learn More: NEET Physics Notes

Note:

In the case of central force L is constant. (Gravitational force is a central force).

Kepler’s Third Law (law of Periods)

Time period of revolution of a planet around the sun is directly proportional to cube of its semimajor axis.

⇒ \(\mathrm{T}^2 \propto \mathrm{a}^3\)

Note:

If average radius is given, then

⇒ \(\mathrm{T}^2 \propto \mathrm{r}^3\)

Universal Law of Gravitation

The force of attraction between two masses mx and m2 seperated by a distance ‘r’ is given by

⇒ \(\mathrm{F}=\mathrm{G} \frac{\mathrm{m}_1 \mathrm{~m}_2}{\mathrm{r}^2}\)

Where G is known as universal gravitational constant. G= 6.67×10^-11Nm²kg^-2

The law of gravitation is strictly valid for point masses.

Shell Theorems

1. A uniformly dense spherical shell exerts force on a body situated outside the shell, as if entire mass of the shell is concentrated at its centre.
2. The force of attraction due to a uniformly dense spherical shell of uniform density, on a point mass situated inside it is zero.

Acceleration due to gravity/Gravitational field intensity/Gravitational field

Gravitational force at a point is the force experienced by a unit mass when placed at that point.

⇒ \(g=\frac{F}{m}\)

Acceleration due to gravity on the surface of earth:

⇒ \(\mathrm{g}=\frac{\mathrm{GM}_{\mathrm{E}}}{\mathrm{R}_{\mathrm{E}}^2}\)

Acceleration due to gravity due to a uniform solid sphere:

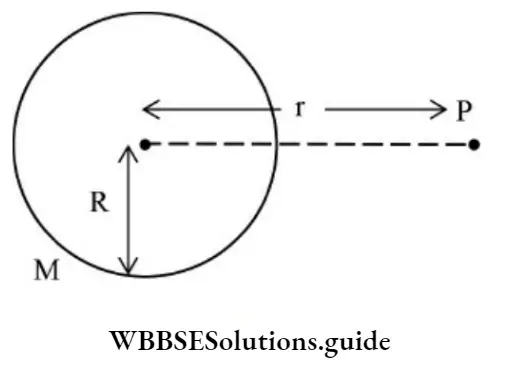
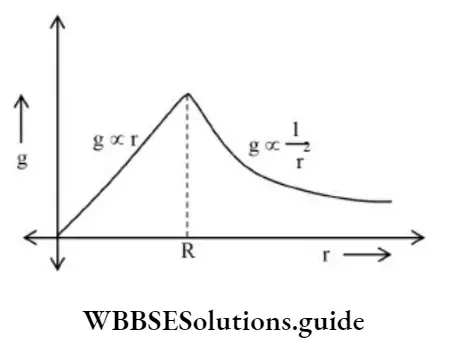
⇒ \(\mathrm{g}_{\mathrm{p}}=\frac{\mathrm{GM}}{\mathrm{r}^2} ; \text { if } \mathrm{r} \geq \mathrm{R}\)

⇒ \(\mathrm{g}_{\mathrm{p}}=\frac{\mathrm{GM}}{\mathrm{R}^3} \mathrm{r} ; \text { if } \mathrm{r}<\mathrm{R}\)

Variation of ‘g’ with distance from the center of the sphere:

Variation of acceleration due to gravity with height:

⇒ \(g(h)=\frac{G M}{(R+h)^2}=\frac{g^2}{(R+h)^2}\)

When h<<R,then

⇒ \(g(h)=g\left(1-\frac{2 h}{R}\right)\)

Where ‘g’ is acceleration due to gravity on the surface of earth.

Variation of acceleration due to gravity with depth:

⇒ \(g(d)=g\left(1-\frac{d}{R}\right)\)

Variation of ‘g’ with latitude due to rotation of earth:

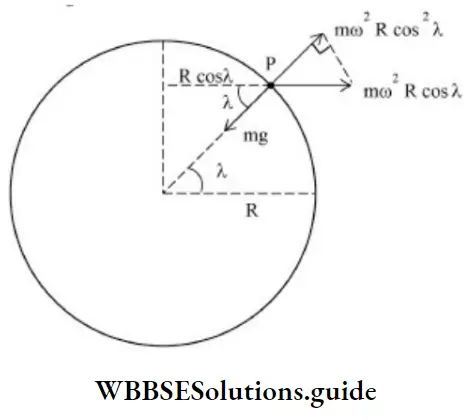
Consider a body of mass ‘m’ at point P.

w.k.t.,

⇒ \(g=\frac{F_{\text {bet }}}{m}\)

⇒ \(g_\lambda=\frac{m g-m \omega^2 R \cos ^2 \lambda}{m}\)

⇒ \(g_\lambda=g-\omega^2 R \cos ^2 \lambda\)

Case 1:

At equator, λ = 0

⇒ \(g_\lambda=g-\omega^2 R\)

If ‘ ω’ of earth increases there is a possibility of ‘g’ at equator becoming zero.

i.e., 0 = g – ω²R ⇒ g=ω²R

⇒ \(\omega=\sqrt{\frac{g}{R}}\)

,i.e., when ω=\(\sqrt{\frac{g}{R}}\) g at equator will become zero.

Case 2:

At pole,  λ = 90°,

∴ g_λ = 0

i.e., ‘g’ at poles is independent of rotation of earth.

Gravitational potential energy at a point is the work done in bringing a mass from infinity to that point against the gravitational force of the field.

⇒ \(\mathrm{U}=-\frac{\mathrm{Gm}_1 \mathrm{~m}_2}{\mathrm{r}}\)

Where ‘r’ is the distance from the centre of earth. For system of ‘3’ particles,

⇒ \(\mathrm{U}=-\mathrm{G}\left(\frac{\mathrm{m}_1 \mathrm{~m}_2}{\mathrm{r}_{12}}+\frac{\mathrm{m}_2 \mathrm{~m}_3}{\mathrm{r}_{23}}+\frac{\mathrm{m}_3 \mathrm{~m}_1}{\mathrm{r}_{31}}\right)\)

For ‘n’ particles,

⇒ \(\mathrm{U}=-\mathrm{G}\left(\frac{\mathrm{m}_1 \mathrm{~m}_2}{\mathrm{r}_{12}}+\frac{\mathrm{m}_1 \mathrm{~m}_3}{\mathrm{r}_{13}}+\ldots . .+\frac{\mathrm{m}_1 \mathrm{~m}_{\mathrm{n}}}{\mathrm{r}_{\mathrm{ln}}}+\frac{\mathrm{m}_2 \mathrm{~m}_3}{\mathrm{r}_{23}}+\ldots . .\right)\)

Note:

For‘n’particle system, there are \({ }^{\mathrm{n}} \mathrm{C}_2=\frac{\mathrm{n}(\mathrm{n}-1)}{2}\) pairs.

Potential energy is calculated for each pair and then added to obtain the potential energy for the system.

⇒ \(F=-\frac{d U}{d x}\)

∴ \(U=-\int_{\infty}^r \vec{F} \cdot d \vec{x}\)

Gravitational potential at a point is the work done in bringing a unit mass from infinity to the given point against the gravitational force of the field.

⇒ \(\mathrm{V}=-\frac{\mathrm{GM}}{\mathrm{r}}\)

Relation between gravitational field and gravitational potential

⇒ \(\mathrm{g}=-\frac{\mathrm{dV}}{\mathrm{dr}}\)

⇒ \(\mathrm{V}=-\int_{\infty}^{\mathrm{r}} \overrightarrow{\mathrm{g}} \cdot \mathrm{d} \overrightarrow{\mathrm{r}}\)

Potential due to solid sphere (if r > R )

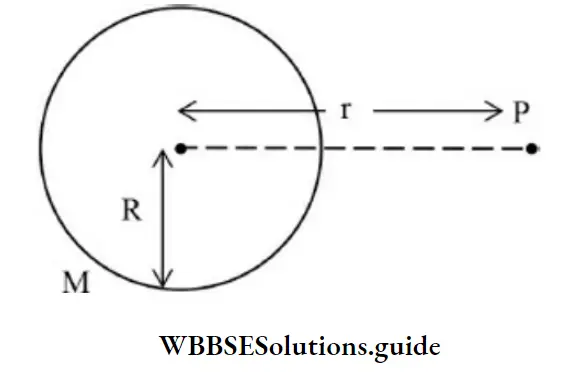
⇒ \(V_p=-\int_{\infty}^r-\frac{G M}{r^2} \cdot d r\)

⇒ \(\mathrm{V}_{\mathrm{p}}=+\mathrm{GM}\left[\frac{\mathrm{r}^{-2+1}}{-2+1}\right]_{\infty}^{\mathrm{r}}=-\frac{\mathrm{GM}}{\mathrm{r}}\)

Potential due to solid sphere (if r < R)

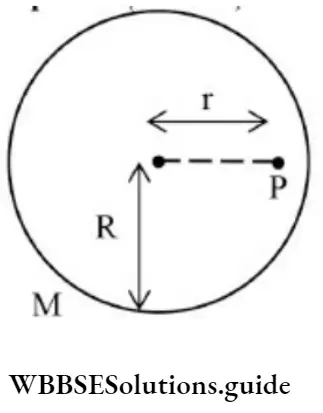
⇒ \(V_P=-\int_{\infty}^R-\frac{G M}{r^2} \cdot d r-\int_R^r-\frac{G M}{R^3} r d r\)

⇒ \(\mathrm{V}_{\mathrm{P}}=\mathrm{GM}\left[\frac{\mathrm{r}^{-1}}{-1}\right]_{\infty}^{\mathbb{R}}+\frac{\mathrm{GM}}{\mathrm{R}^3}\left[\frac{\mathrm{r}^{+2}}{+2}\right]_{\mathbb{R}}^{\mathrm{r}}\)

⇒ \(V_p=-\frac{G M}{R}+\frac{G M}{2 R^3}\left(r^2-R^2\right)\)

⇒ \(V_p=\frac{G M}{2 R^3}\left(r^2-R^2\right)-\frac{G M}{R}\)

⇒ \(V_P=\frac{G M}{2 R^3}\left[\left(r^2-R^2\right)-2 R^2\right]\)

⇒ \(V_P=-\frac{G M}{2 R^3}\left[3 R^2-r^2\right]\)

Orbital Speed of a Satellite

When gravitational force and centrifugal force on the satellite are same, then

⇒ \(\frac{\mathrm{GMm}}{\mathrm{r}^2}=\frac{\mathrm{mv}_0^2}{\mathrm{r}}\)

⇒ \(\mathrm{v}_0^2=\frac{\mathrm{GM}}{\mathrm{r}}\)

⇒ \(v_0=\sqrt{\frac{G M}{r}}=\sqrt{\frac{G M}{R+h}}\)

Where ‘h’ is the height of the satellite from earth’s surface. If h < < R, then,

⇒ \(\mathrm{v}_0=\sqrt{\frac{\mathrm{gR}^2}{\mathrm{R}}} \Rightarrow \mathrm{v}_0=\sqrt{\mathrm{gR}}\)

Expression for escape speed

⇒ \(v_e=\sqrt{\frac{2 G M}{r}}=\sqrt{\frac{2 G M}{R+h}}\)

If h < < R, then

⇒ \(\mathrm{v}_{\mathrm{e}}=\sqrt{\frac{2 \mathrm{gR}^2}{\mathrm{R}}} \Rightarrow \mathrm{V}_{\mathrm{e}}=\sqrt{2 \mathrm{gR}}\)

Note:

If a satellite is very close to earth’s surface, then,

⇒ \(\mathrm{v}_{\mathrm{e}}=\sqrt{2} \mathrm{v}_0\)

Total Energy of a Satellite

Kinetic energy of a satellite is given by,

⇒ \(\mathrm{K}=\frac{1}{2} \mathrm{mv}_0^2=\frac{1}{2} \mathrm{~m} \frac{\mathrm{GM}}{\mathrm{r}}=\frac{\mathrm{GMm}}{2 \mathrm{r}}\)

Potential energy of a satellite is given by,

⇒ \(\mathrm{U}=-\frac{\mathrm{GMm}}{\mathrm{r}}\)

∴ Total energy,

⇒ \(\mathrm{E}=\mathrm{K}+\mathrm{U}=\frac{\mathrm{GMm}}{2 \mathrm{r}}-\frac{\mathrm{GMm}}{\mathrm{r}}\)

⇒ \(\mathrm{E}=-\frac{\mathrm{GMm}}{2 \mathrm{r}}\)