WBBSE Solutions For Class 8 Maths Chapter 5 Cubes

Cubes

Today Sundy and Firoz are making many colourful boxes.

Measuring we see that the length of the box is 1 cm, breadth is 1 cm and height is 1 cm, i.e., each of these boxes is cubical.

Length of each side of this cubical box is 1 cm.

But to make a cubical box of side 3 cm in length,27 small boxes are required.

What shall we call such numbers like 1, 8, 27,?

1,8, 27 are called perfect cube numbers because 1 = (1)3, 8

= (2)³, 27 = 3³, 8 = 2³, 64 = 4³, 125 = 5³, .

i.e., cubes of 1,2, 3, 4, 5, are respectively 1, 8, 27, 64, 125,…

I try to make a cube with 32 cubes of same size:

32 = 2×2×2×2×2

= (2 × 2) x (2 × 2) × 2

= 4×4×4, i.e., 32 ≠ (any integer)3

Read and Learn More WBBSE Solutions For Class 8 Maths

We see, it not possible to make a bic, cube with 32 small cubes. But it is possible to make a big cube with 32×2=64 small cubes. So, 64 is a perfect cube number, i.e., 32 is not a perfect cube number but we get a perfect cube number by multiplying 32 with the smallest positive integer 2 because 64 = 4³

Let’s see whether Tatai can make a big cube with 54 small cubes of the same size as above.

54 = 2×3×3×3

We see 54 is a not-perfect cube number (Perfect cube no. / not perfect cube number.)

∴  Is 54? If we divide by the smallest positive integer then we will get perfect cube number. So, 54÷2 = 27 a not perfect cube number Because 27 = 3³.

Cubes Exercise

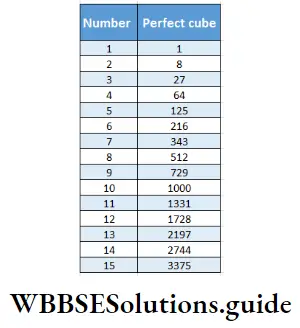
Question 1. Let’s find perfect cube from the following numbers: 125, 500, 64, 73, 729, 968.

Solution:

Given

125, 500, 64, 73, 729, 968.

125 = 5³, 64 = 4⁴, 73, 729 = 9³

Let’s write perfect cubes in the chart below, making cube from 1 to 20.

See the term from the above chart 9 = 1³ + 2³, 280= 4³+ 6³, 1729 = 10³+ 10³(Let’s make another).

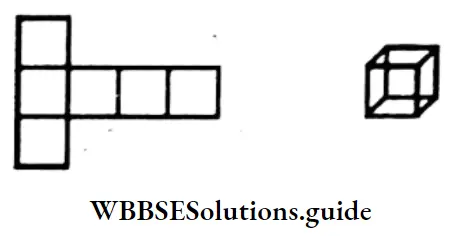
Firoz made many big cubes attaching these small cubes. Suhana and I are measuring the length of each side of cube.

There are 27 small cubes in this big cube.

See, the length of each side of big cube beside the picture is 3 cm. I got differently 27 = 3³

What can be termed 3 of 27?

Cubing 3, we get 27.

So the cube root of 27 is 3.

125= 5×5×5=5³

∴ \(\sqrt[3]{125}\) = 5

729 = 3×3×3×3×3×3

= 3³+3³

∴ \(\sqrt[3]{729}\) = 3×3=9

Cubes Exercise

Question 1. Let’s form two cubes with sides of length 5 cm and 1 cm. Let us calculate the number of small cubes to form the big cube.

Solution:

Given

Sides of length 5 cm and 1 cm.

Number of small cubes required to make this big cube = (5)³ = 5 × 5 × 5 = 125

Question 2. Sumanta has made many cubes of 1 cm length. Manami is trying to make big cubes attaching these small cubes. Let’s see in which of the cube given below Manami will be able to make big cubes.

1. 100

Solution:

Given

100 = 2 × 2 × 5 × 5 = 2² × 5²

2. 1000

Solution:

Given

1000 = 10 × 10 × 10 = (10)³

∴ \(\sqrt[3]{1000}\) = 10

3. 1331

Solution:

Given

1331 = 11×11×11= (11)³

∴ \(\sqrt[3]{1331}\)=11

4. 324

Solution:

Given

324 = 2×2×3×3×3×3 = (2)² × (3)² × (3)²

5. 3375.

Solution:

Given

3375 = 15×15×15 = (15)³

∴ \(\sqrt[3]{3375}\) = 15

6. 1372

Solution:

Given

1372 = 2×2×7×7×7 = 2^2×7³

Question 2. Manami can make a big cube by attaching the following number of small cubes :

Solution:

• 1000,
• 1331
• 3375

Question 3. Let us write number which is not a perfect cube in the numbers given below :

1. 216

Solution:

Given

216 = 2 x 2 x 2 x 3 x 3 x 3

= 2³ x 3³

= 6³

216 = 6³

2. 343

Solution:

Given

343 = 7 x 7 x 7

= 7³

343 = 7³

3. 1024

Solution:

Given

1024 =2×2×2×2×2×2×2×2×2×2

= 2³×2³×2³×2

= 8³×2

1024 = 8³×2

4. 324

Solution:

Given

324 = 2×2×3×3×3×3

= 2³ × 3³ × 3

324 = 2³ × 3³ × 3

5. 1744

Solution:

Given

1744 = 2 × 2 × 2 × 2 × 109

= 2³×2 ×109

1744 = 2³×2 ×109

6. 1372

Solution:

Given

1372 = 2 × 2 × 7 × 7 × 7

= 2² × 7³

1372 = 2² × 7³

The following aren’t perfect cube numbers :

• 1024,
• 324,
• 1744 and
• 1372

Question 4. Dabnath has made a cuboid whose length, breadth and height are respectively 4 cm, 3 cm, and 3 cm. Let’s see how many cuboids of this type will form a cube.

Solution:

Given

Dabnath has made a cuboid whose length, breadth and height are respectively 4 cm, 3 cm, and 3 cm.

Length of the cuboid = 4 cm

Breadth = 3 cm.

Height = 3 cm

∴  Number of cuboids required to make the cube = 4×3×3 = 36

Question 5. Let us calculate with which positive smallest number should be multiplied with the numbers below so that the product will be a perfect cube.

1. 675

Solution:

Given

675 = 3 ×3 × 3 × 5 × 5 = 3³ × 5²

∴  675 is not a perfect cube number.

But, 3³ ×5² × 5 = 3³ × 5³ = (15)³

= 3375

Multiplying 675 by the smallest positive number 5, the product 3375 will be a perfect cube number.

2. 200

Solution:

Given

200 = 2×2×2×5×5 = 2³×5²

∴ 200 is not a perfect cube number.

But, 2³×5²×5 = 23×5³=(10)³ = 1000

Multiplying 200 by the smallest positive number 5, the product 1000, will be a perfect cube number.

3.108

Solution:

Given

108 = 3 × 3 × 3 × 2 × 2 = 3³ × 2²

∴ 108 is not a perfect cube number.

But, 3³ × 2²×2

= 3³ × 2³

= (6)³

= 216

∴  Multiplying 108 by the smallest positive number 2, the product 216 will be a perfect cube number.

4. 121.

Solution:

Given

121 = 11 ×11 = (11)²

∴ 121 is not a perfect cube number

But, (11)² × 11 = (11)³ = 1331

∴ Multiplying 121 by the smallest positive number 11, the product 1331 will be & perfect cube number.

5. 1225

Solution:

Given

1225 = 5×5×7×7 = 5²×7²

∴  1225 is not a perfect cube number

But, 5² × 7² × 5 × 7

= 5³ × 7³

= (35)³

= 42875

∴ Multiplying 1225 by the smallest positive number 35, the product 42875 will be a perfect cube number.

Question 6. Let us calculate with which positive smallest number should the number be divided so that the quotient will be a perfect cube.

1. 7000

Solution:

Given

7000 = 7 × 10 × 10 × 10 = 7 × (10)³

∴ Dividing 7000 by the smallest positive number 7, the quotient will be a perfect cube number.

∴ Required number = 7

2. 2662

Solution:

Given

2662 = 2×11 × 11 × 11 = 2 × (11)³

∴ Dividing 2662 by the smallest positive number 2, the quotient will be a perfect cube number.

∴ Required number = 2

3. 4394

Solution:

Given

4394 = 2 × 13 × 13 × 13 = 2 × (13)³

Dividing 4394 by the smallest positive number 2, the quotient will be a perfect cube number.

Required number = 2

4. 6750

Solution:

Given

6750 = 2 × 3 × 3 × 3 × 5 × 5 × 5 = 2 × 3³ × 5³

Dividing 6750 by the smallest positive number 2, the quotient will be a perfect cube number.

Required number = 2

5. 675

Solution:

Given

675 = 3×3×3×5×5 = 3³×5²

Dividing 675 by the smallest positive number 25, the quotient will be a perfect cube number.

Required number = 25

Question 7. Let us write the number below as product of prime factors and write the cube roots of the numbers.

1. 512

Solution:

Given

512 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2

The cube root of 512

= 2³ × 2³ × 2³ = 8³

∴ \(\sqrt[3]{512}\) = 8

The cube root of 512 = 8

2. 1728

Solution:

Given

1728 = 2×2×2×2×2×2×3×3×3

= 2³×2³×3³

=(12)³

∴ \(\sqrt[3]{1728}\)= 12

The cube root of 1728 = 12

3. 5832

Solution:

Given

5832 = 2×2×2×3×3×3×3×3×3

= 2³× 3³×3³=(18)³

∴ \(\sqrt[3]{5832}\)= 18

The cube root of 5832 = 18

4. 15625

Solution:

Given

15625 = 5×5×5×5×5×5

= 5³×5³ = (25)³

∴ \(\sqrt[3]{15625}\) = 25

The cube root of 15625 = 25

5. 10648

Solution:

Given

10648 = 2×2×2×11×11×11

= 2³× 11³= (22)³

∴ \(\sqrt[3]{10648}\) = 2

After measuring we see that the length of a side of this cubical box is 12 cm. ‘

∴ Volume = 12³ cc. = 1728 cc.

Question 8. If the length of a side of the cubical box was x cm, then the volume was = (x cm)³ = x³ cc. If the length of a side of the cubical box was (x + 2) cm, then the volume of the box was = (x + 2)³ cm.

Solution:

Given

If the length of a side of the cubical box was x cm, then the volume was = (x cm)³ = x³ cc. If the length of a side of the cubical box was (x + 2) cm, then the volume of the box was = (x + 2)³ cm

Let us see what we get after expanding (x + 2)³.

(x + 2)³ = (x + 2) x (x + 2)²

= (x + 2) {x² + 4x + 4} [with the help of identity (a + b)² = a² + 2ab + b²]

= (x + 2) x² + (x + 2) 4x + (x + 2) 4 [with the help of Distributive Law]

= x³+ 2x² + 4×2+ 8x + 4x + 8 [with the help of associative] law]

= x³+ 6x² + 12x + 8

Titli wrote the length of a side of this cube as (a + b) cm.

∴ The volume of this cube is (a + b)³ cc.

= (a + b)³ = (a + b) (a + b)²

= (a + b) × a² + 2ab + b2

= (a + b) x a² + (a + b) 2ab + (a + b) b² [Distributive Law]

= a³ + b²a + 2a²b + 2ab² + ab² + b³ [Distributive Law]

= a³ + ab² + 2a²b + 2ab² + ab² + b³ [b²a = ab², by commutative Law of Multiplication.]

= a³ + 3a²b + 3ab² + b3

We get (a + b)³ = a³ + 3a²b + 3ab² + b³

(a + b)³ = a³ + 3a²b + 3ab² + b³

= a³ + 3ab (a + b) + b³

We got with the help of commutative & distributive laws (a + b)³ = a³+ b³ + 3ab (a + b)

1. (2y + 3)³ (Let’s expand).

Solution:

Given (2y + 3)³

= (2y)³ + 3 (2y)³ 3 + 3.2y. (3)³ + (3)³ there a =2y b = 3

(2y + 3)³ = (2y)³ + 3 (2y)³ 3 + 3.2y. (3)³ + (3)³

2. (15)³

Solution:

Given (15)³

= (10+5)³

= 3375

(15)³ = 3375

3. (101)³

Solution:

Given (101)³

= (100+1)

= (100+1)

= 1030301

(101)³ = 1030301

4. (210)

Solution:

Given (210)

= (200+10)³

= 9261000

(210) = 9261000

5. If the length of one side of the cube is = (a – b) cm, we get,

Solution:

The volume of the cube = (a – b)³ cc

(a – b)³ Let’s see by expanding

(a – b)³ = {a + (- b)}³ = a³ + 3 (a²) (-b) + 3a (-b)² + (-b)³

(a – b)³ = a³ – 3a³b + 3ab² – b³

With the help of identity no.3, I expand

6. (99)³ and find the value of it.

Solution:

Given (99)³

= (100 – 1)³

= (100)³ – 3 (100)2 x 1 + 3 x 100 x (1 )2 – (1 )³

= 970299

(99)³ = 970299

Question 9. If x – \(\frac{1}{9 x}\)= 1 then find the value of 27×3 – \(\frac{1}{27 x^3}\)

Solution:

= \(x-\frac{1}{9 x}=1\)

or, \(3\left(x-\frac{1}{9 x}\right)\) = 1×3(Multiplying both sides by 3)

or,\(3 x-\frac{1}{3 x}\) = 3

or,\(\left(3 x-\frac{1}{3 x}\right)^3\) = 3 (Cubing both sides)

or,\(27 x^3-\frac{1}{27 x^3}-3 \times 3 x \times \frac{1}{3 x}\left(3 x-\frac{1}{3 x}\right)\) = 27

or,\(27 x^3-\frac{1}{27 y^3}-3 \times 3\) = 27

∴ \(27 x^3-\frac{1}{27 x^3}\) = 36

Question 10. Let us solve the questions below with the help of identities from I to IV.

1.  If x – y = 2 then find the value of x³ – y³ – 6xy.

Solution:

Given x – y = 2

x³ – y³ – 6xy = x³ – y³ – 3xy.2 = x³ – y³ – 3xy (x – y)

= (x – y)³.

= (2)³

= 8

The value of x³ – y³ – 6xy = 8

2. If a + b = – -then prove that a³+ b³ – ab = \((a+b)^3-3 a b(a+b)-a b\)

Solution:

Given a³+ b³ – ab = \((a+b)^3-3 a b(a+b)-a b\)

L.H.S. = a³ + b³ – ab .

= (a + b)³ – 3ab (a + b) – ab

a³+ b³ – ab = (a + b)³ – 3ab (a + b) – ab

= R.H.S. Proved

3. If x + y = 2 and \(\frac{1}{x}+\frac{1}{y}=2\) then find the value of x³ + y³.

Solution:

Given

x + y = 2 and \(\frac{1}{x}+\frac{1}{y}=2\)

= \(\frac{1}{x}+\frac{1}{y}=2\)

= \(\frac{y+x}{x y}\) =2

= \(\frac{x+y}{x y}\) = 2

or, \(\frac{2}{x y}\)= 2

or, xy = 1

= x³ + y³

= (x + y)³ – 3xy (x + y)

= (2)³ – 3×1 x 2

= 8-6

= 2

The value of x³ + y³ = 2

4. If \(\frac{x^2-1}{x}\) = 2 then find the value of \(\frac{x^6-1}{x^3}\)

Solution:

Given

\(\frac{x^2-1}{x}\) = 2

= \(\frac{x^2-1}{x}=2\)

or, \(\frac{x^2}{x}-\frac{1}{x}=2\)

or,\(x-\frac{1}{x}=2\)

∴ \(\frac{x^6-1}{x^3}\)

= \(\frac{x^6}{x^3}-\frac{1}{x^3}\)

= \(x^3-\frac{1}{x^3}\)

= \((x)^3-\left(\frac{1}{x}\right)^3\)

= \(\left(x-\frac{1}{x}\right)^3+3 \cdot x \cdot \frac{1}{x}\left(x+\frac{1}{x}\right)\)

= (2)³ + 3×2

= 8 + 6

= 14

The value of \(\frac{x^6-1}{x^3}\) = 14

5.  If x + \(\frac{1}{x}\) = 5 then find the value of x³ + \(\frac{1}{x^3}\)

Solution:

Given

x + \(\frac{1}{x}\) = 5

= \(x+\frac{1}{x}=5\)

= \(x^3+\frac{1}{x^3}\)

= \((x)^3+\left(\frac{1}{x}\right)^3\)

= \(\left(x+\frac{1}{x}\right)^3-3 \cdot x \cdot \frac{1}{x}\left(x-\frac{1}{x}\right)\)

= (5)³-3×5

= 125-15

=110

The value of x³ + \(\frac{1}{x^3}\) =110

6. If x = y + z then find the value of x³– y³– 3xyz.

Solution:

Given

x = y + z

= x – y = z

= (x – y)³ = (z)³ (Cubing both sides)

= x³– y³– 3xy (x – y) = z³

= x³– y³ – 3xy.z = z³ (∴x – y = z)

= x³– y³ – 3xy.z=0

The value of x³– y³– 3xyz =0

7. If xy ( x + y) = m then prove that x³+ y³+ 3m =\(\frac{m^3}{x^3 y^3}\)

Solution:

Given xy ( x + y) = m

xy ( x + y) = m

= x + y=\(\frac{m}{x y}[latex]

= [latex](x+y)^3=\left(\frac{m}{x y}\right)^3\)

= \(x^3+y^3+3 x y(x+y)=\frac{m^3}{x^3 y^3}\)

= \(x^3+y^3+3 x y \cdot \frac{m}{x y}=\frac{m^3}{x^3 y^3}\)

= \(x^3+y^3+3 m=\frac{m^3}{x^3 y^3}\)

Proved.

8. If 2x + \(\frac{1}{3 x}\) = 4 then prove that 27x³ + \(\frac{1}{8 x^3}\) = 189.

Solution:

Given

2x + \(\frac{1}{3 x}\) = 4

= \(2 x+\frac{1}{3 x}=4\)

= \(\frac{3}{2}\left(2 x+\frac{1}{3 x}\right)=\frac{3}{2} \times 4\)(Multiplying sides by \(\frac{3}{2}\)

= \(3 x+\frac{1}{2 x}=6\)

= \(\left(3 x+\frac{1}{2 x}\right)^3=(6)^3\)(Cubing both sides)

= \((3 x)^3+\left(\frac{1}{2 x}\right)^3+3.3 x \frac{1}{2 x}\left(3 x+\frac{1}{2 x}\right)=216\)

= \(27 x^3 \frac{1}{8 x^3}+\frac{9}{2} x 6=216\)

= \(27 x^3+\frac{1}{8 x^3}=216-27\)

= \(27 x^3+\frac{1}{8 x^3}=189\)

Proved.

9. If 2a-\(\frac{2}{a}\)+1=0 then find the value of \(a^3-\frac{1}{a^3}+2\)

Solution:

Given

2a-\(\frac{2}{a}\)+1=0

= \(2 a-\frac{2}{a}+1=0\)

or, \(2\left(a-\frac{1}{a}\right)=-1\)

or, \(a-\frac{1}{a}=-\frac{1}{2}\)

∴ \(a^3-\frac{1}{a^3}+2\)

= \((a)^3-\left(\frac{1}{a}\right)^3+2\)

= \(\left(a-\frac{1}{a}\right)^3+3 \cdot a \cdot \frac{1}{a}\left(a-\frac{1}{a}\right)+2\)

= \(\left(-\frac{1}{2}\right)^3+3\left(-\frac{1}{2}\right)+2\)

= \(\left(-\frac{1}{2}\right)^3+3\left(-\frac{1}{2}\right)+2\)

= \(-\frac{1}{8}-\frac{3}{2}+2\)

= \(\frac{-1-12+16}{8}\)

= \(\frac{3}{8}\)

The value of \(a^3-\frac{1}{a^3}+2\) = \(\frac{3}{8}\)

10. a³+b³+c³ = 3abc then find the value of (a+b+c). Given (a≠b≠c).

Solution:

Given a³+b³+c³ = 3abc

or, (a+b)³-3ab(a+b)+c³=3abc

or, (a+b)³+c³-3ab(a+b)-3abc=0

or, (a+b+c){(a+b)²-(a+b)c+(c)²}=0

or, (a+b+c) (a²+2ab+b²-ac-bc+c²-3ab)=0

or, (a+b+c) (a²+b²+c²-ab-ac-ac)=0

∵ The product of both expressions is zero.

∵ At least one of the expressions is zero.

∵ Here a≠b≠c

∵ a+b+c=0

The value of (a+b+c) =0

11. If m+n=5 and mn=6 then find the value of (m²+n²)(m³+n³).

Solution:

Given m+n=5 and mn= 6

(m²+n²) (m³+n³)

= {(m+n)-2mn}{(m+n)³-3mn(m+n)}

= {(5)²-2×6}{(5)³-3×6×5}

= (25-12) (125-90)

= 13×35

= 455

The value of (m²+n²)(m³+n³) = 455

Question 11. Let’s multiply with the help of identity no.5

1. (7 + 3x) (49 – 21 x + 9x²)

Solution:

Given (7 + 3x) (49 – 21 x + 9x²)

= (7 + 3x) (49 – 21 x + 9x²)

= (7 + 3x) {(7)² – 7 x 3x + (3x)²}

= (7)³ + (3x)³

= 343 + 27x³

(7 + 3x) (49 – 21 x + 9x²) = 343 + 27x³

2. (x² + y2) (x4 – x²y² + y⁴)

Solution:

Given (x² + y²) (x⁴ – x²y² + y⁴)

(x²+y²){(x²)²-x²y²+(y²)²}

= (x²)³+(y²)³

= x⁶+y⁶

(x² + y²) (x⁴ – x²y² + y⁴) = x⁶+y⁶

Question 12. Let’s try to factorize the following algebraic expression with the help of identity no. V

1. p³q³ + 1

Solution:

Given p³q³ + 1

= p³q³ + 1

= (pq)³ + (1)³

= pq+1×p²q²-pq+1

p³q³ + 1 = pq+1×p²q²-pq+1

Question 13. Let’s find the product of the following algebraic expression with the help of identity no. VI

1. (p – 2q) (p² + 2pq + 4q²)

Solution:

Given (p – 2q) (p² + 2pq + 4q²)

= (p – 2q) (p² + 2pq + 4q²)

= (P – 2q) {p²+ p x 2q + (2q)²}

= (p)³ – (2q)³ [ By identity no. V ]

= (p)³-8q³

(p – 2q) (p² + 2pq + 4q²) = (p)³-8q³

2. (x² – 1) (x⁴ + x² + 1)

Solution:

Given (x² – 1) (x⁴ + x² + 1)

= (x² – 1) {(x²)² + x² x 1 + 1²}

= ((x²)³-(1)³)

= x⁶-1

(x² – 1) (x⁴ + x² + 1) = x⁶-1

3. 2x {(4x – 3)2 + (4x – 3) (2x – 3) + (2x – 3)2}

Solution:

Given 2x {(4x – 3)2 + (4x – 3) (2x – 3) + (2x – 3)2}

= {(4x – 3) – (2x – 3)} {(4x – 3)² + (4x – 3) + (2x – 3)²}

[Since : (4x – 3) – (2x – 3)} = 4x – 3 – 2x + 3 = 2x]

Let 4x – 3 = a and 2x – 3 = b = (a – b) {a² + ab + b²}

= a³ – b³ [ With the help of identity no. VI]

= (4x – 3)³– (2x -3)³ [By replacing a = 4x – 3 and b = 2x – 3]

= {(4x)³ – 3.(4x)² x 3+3.4x.3³-(3)³}-{(2x)³-3(2x)² 3+3 x 2x x 32.3³}

= 64x³ – 144x² + 108x – 27 – {8x³ – 36x² + 54x – 27}

= 56x³ – 108x² + 54x

2x {(4x – 3)2 + (4x – 3) (2x – 3) + (2x – 3)2} = 56x³ – 108x² + 54x

Question 14. Let’s factorize the following algebraic expressions with the help of identity no. VI

1. 64 I³ – 34³

Solution:

Given 64 I³ – 34³

= (4l)³-(7)³

= (4l-7)×(16l2+28l+49)

64 I³ – 34³ = (4l-7)×(16l2+28l+49)

2. (x+2)³-(x-2)³

Solution:

Given (x+2)³-(x-2)³

= {(x + 2) – (x – 2)} {(x + 2)² + (x + 2) (x – 2) + (x – 2)²}

= (x + 2 – x + 2) {x² + 4x + 4 + x² – 4x + 4}

= 4x3x²+4

(x+2)³-(x-2)³ = 4x3x²+4

Question 15. Let’s factorize the following algebraic expressions with the help of identity no. 4

1. 8m³ + 12m²n + 6mn² + 2n³

Solution:

Given 8m³ + 12m²n + 6mn² + 2n³

= (2m)³ + 3(2m)² n+3.2mn² + n³ + n³

= (2m + n)³ + n³

= 2m + 2n x

8m³ + 12m²n + 6mn² + 2n³  = 2m + 2n x

2. a³ – 9b³ + (a + b)³

Solution:

Given a³ – 9b³ + (a + b)³

= a³ – b³ – 8b³ + (a + b)³

= a³ – b³+ x(a + b)³ – (2b)³

= (a – b) (a² + ab + b²)+(a + b – 2b)x{(a + b)² + 2b(a + b)+4b²}

= (a – b) (a² + ab + b² + a² + 2ab + b² – 2ab – 2b² + 4b²}

= (a – b)×(2a²+ab+4b²)

a³+b³=a+b×a²+ab+b²)

a³-b³=(a-b)×(a²-ab+b²)

a³ – 9b³ + (a + b)³ = (a – b)×(2a²+ab+4b²)

Let’s simplify using formula :

1. (a + b) (a – b) (a² + ab + b²) (a² – ab + b²)

Solution:

Given (a + b) (a – b) (a² + ab + b²) (a² – ab + b²)

= (a + b) (a² – ab + b²) (a – b) (a² + ab + b²)

= (a³+b³) (a³-b³)

= (a³)²-(b³)² = a6 – b6

2. (a – 2b) (a² + 2ab + 4b²) (a3 + 8b3)

Solution:

Given (a – 2b) (a² + 2ab + 4b²) (a3 + 8b3)

= (a – 2b) {(a)² + a. 2b + (2b)2} (a³ + 8b³)

= {(a)3 – (2b)³} (a³ + 8b³) = (a³-8b³) (a³ + 8b³)

= (a³)² – (8b³)²

= a6 – 64b6

3. (4a² – 9) (4a² – 6a + 9) (4a² + 6a + 9)

Solution:

Given (4a² – 9) (4a² – 6a + 9) (4a² + 6a + 9)

= {(2a)² – (3)²} (4a² – 6a + 9) (4a² + 6a + 9)

= (2a + 3) (2a – 3) (4a² – 6a + 9) (4a² + 6a + 9)

= (2a + 3) {(2a)² – 2a.3 + (3)²} (2a – 3) {(2a)2 + 2a.3 + (3)²}

= {(2a)³ + (3)³} {(2a)³ – (3)³} .

= (8a³ + 27) (8a³ – 27)

= (8a³)² – (27)²

= 64a⁶-729

(4a² – 9) (4a² – 6a + 9) (4a² + 6a + 9) = 64a⁶-729

4. (x-y) (x²+xy+y²) + (y-z) (y²+yz+z²) + (z-x) (z²+zx+x²)

Solution:

Given (x-y) (x²+xy+y²) + (y-z) (y²+yz+z²) + (z-x) (z²+zx+x²)

= x³ – y³ + y³ – z³ + z³ – x³ = 0

(x-y) (x²+xy+y²) + (y-z) (y²+yz+z²) + (z-x) (z²+zx+x²) = 0

5.  (x+1) (x²-x+1) + (2x-1) (4x²+2x+1 )-(x-1) (x²+x+1)

Solution:

Given (x+1) (x²-x+1) + (2x-1) (4x²+2x+1 )-(x-1) (x²+x+1)

= (x)³ + (1 )³ + (2x)³ – (1 )³ (x)³ – (1 )³}

= x³ + 1 + 8x³ – 1- (x³ – 1)

= 9x³ – x³ + 1

= 8x³+ 1

(x+1) (x²-x+1) + (2x-1) (4x²+2x+1 )-(x-1) (x²+x+1) = 8x³+ 1

6. If x + \(\frac{1}{x}\)= -1 then find the value of (x³ – 1).

Solution:

Given x + \(\frac{1}{x}\)= -1

\(\left(x+\frac{1}{x}\right) x=-1(x)\)

x²+x+1=0[by transposition]

x³-1

or, (x-)x ×  x²+x+1

=(x-1)×0=0

The value of (x³ – 1)=0

7.  If a + \(\frac{9}{a}\)= 3 then find the value of (a3 + 27).

Solution:

Given a + \(\frac{9}{a}\)= 3

or, \(\frac{a^2+9}{a}\) = 3

or, a²+9=3a

or, a² – 3a + 9 = 0

= a³+ 27 = (a)³+ (3)³

= (a + 3) {(a)² – a.3 + (3)²}

= (a + 3) (a² – 3a + 9)

= (a + 3) x 0 = 0

The value of (a³ + 27) = 0

8. If \(\frac{a}{b}+\frac{b}{a}\)= 1 then find the value of (a3 + b3).

Solution:

Given \(\frac{a}{b}+\frac{b}{a}\)= 1

= \(\frac{a}{b}+\frac{b}{a}\)= 1

= \(\frac{a^2+b^2}{a b}=1\)

a² +b²=ab

or, a²-ab+b²= 0

∴ a³ + b³

= (a + b) (a² – ab + b²)

= (a + b) x 0 = 0

The value of (a³ + b³) = 0

9. Let’s factorize the following algebraic expressions :

1. 1000a³+ 27b6

Solution:

Given 1000a³+ 27b6

= (10a)³ + (3b2)³

= (10a + 3b²) {(10a)² – 10a.3b² + (3b2)²}

= (10a + 3b²) (100a² – 30ab² + 9b4)

1000a³+ 27b6 = (10a + 3b²) (100a² – 30ab² + 9b4)

2. 1-216z³.

Solution:

Given 1-216z³

=(1)3-(6z)³

= (1- 6z) {(1)² + 1.6z + (6z)²}

= (1-6z) (1 + 6z + 36z²)

1-216z³ = (1-6z) (1 + 6z + 36z²)

3. m⁴-m

Solution:

Given m⁴-m

= m (m³ – 1)

= m{(m)³-(1)³}

= m (m-1) (m² + m + 1)

m⁴-m = m (m-1) (m² + m + 1)

4. 192a³ + 3

Solution:

Given 192a³ + 3

= 3 (64a³ + 1)

= 3 {(4a)³ + (1)³}

= 3 (4a + 1) {(4a)² – 4a. 1 + (1)²}

= 3 (4a+ 1) (16a²-4a + 1)

192a³ + 3 = 3 (4a+ 1) (16a²-4a + 1)

5. 16a4x3³ + 54ay³

Solution:

Given 16a4x3³ + 54ay³

= 2a (8a³x³ + 27y³)

= 2a {(2ax)³ + (3y)³}

= 2a (2ax + 3y) {(2ax)² – 2ax.3y + (3y)²}

= 2a (2ax + 3y) (4a²x² – 6axy + 9y²)

16a4x3³ + 54ay³ = 2a (2ax + 3y) (4a²x² – 6axy + 9y²)

6. 729a³b3c³ – 125

Solution:

Given 729a³b3c³ – 125

= (9abc)³ – (5)³

= (9abc – 5) {(9abc)² + 9abc.5 + (5)2}

= (9abc – 5) (81a²b2c² + 45abc + 25)

729a³b3c³ – 125 = (9abc – 5) (81a²b2c² + 45abc + 25)

7. \(\frac{27}{a^3}-\frac{1}{27 b^3}\)

Solution:

Given \(\frac{27}{a^3}-\frac{1}{27 b^3}\)

⇒ \(\left(\frac{3}{a}\right)^3-\left(\frac{1}{3 b}\right)^3 \)

⇒ \(\left(\frac{3}{a}-\frac{1}{3 b}\right)\left\{\left(\frac{3}{a}\right)^2+\frac{3}{a} \cdot \frac{1}{3 b}+\left(\frac{1}{3 b}\right)^2\right\} \)

⇒ \(\left(\frac{3}{a}-\frac{1}{3 b}\right)\left(\frac{9}{a^2}+\frac{1}{a b}+\frac{1}{9 b^2}\right)\)

\(\frac{27}{a^3}-\frac{1}{27 b^3}\)= \(\left(\frac{3}{a}-\frac{1}{3 b}\right)\left(\frac{9}{a^2}+\frac{1}{a b}+\frac{1}{9 b^2}\right)\)

8. \(\frac{x^3}{64}-\frac{64}{x^3}\)

Solution:

Given \(\frac{x^3}{64}-\frac{64}{x^3}\)

⇒ \(\left(\frac{x}{4}\right)^3-\left(\frac{4}{x}\right)^3\)

⇒ \( \left(\frac{x}{4}-\frac{4}{x}\right)\left\{\left(\frac{x}{4}\right)^2+\frac{x}{4} \cdot \frac{4}{x}+\left(\frac{4}{x}\right)^2\right\} \)

⇒\( \left(\frac{x}{4}-\frac{4}{x}\right)\left\{\left(\frac{x}{4}\right)^2+2 \cdot \frac{x}{4} \cdot \frac{4}{x}+\left(\frac{4}{x}\right)^2-\frac{x}{4} \cdot \frac{4}{x}\right\} \)

⇒ \(\left(\frac{x}{4}-\frac{4}{x}\right)\left\{\left(\frac{x}{4}+\frac{4}{x}\right)^2-1\right\} \)

⇒ \( \left(\frac{x}{4}-\frac{4}{x}\right)\left\{\left(\frac{x}{4}+\frac{4}{x}\right)^2-(1)^2\right\} \)

⇒ \(\left(\frac{x}{4}-\frac{4}{x}\right)\left(\frac{x}{4}+\frac{4}{x}+1\right)\left(\frac{x}{4}+\frac{4}{x}-1\right)\)

\(\frac{x^3}{64}-\frac{64}{x^3}\)=  \(\left(\frac{x}{4}-\frac{4}{x}\right)\left(\frac{x}{4}+\frac{4}{x}+1\right)\left(\frac{x}{4}+\frac{4}{x}-1\right)\)

9. x³ + 3x²y + 3xy² + 2y³

Solution:

Given x³ + 3x²y + 3xy² + 2y³

= x³ + 3x²y + 3xy² + y³ + y³

= (x + y)³ + y³

= (x + y + y) {(x + y)² – (x + y) y + (y)²}

= (x + 2y) (x² + 2xy + y²– xy – y² + y²)

= (x + 2y) (x² + xy + y²)

x³ + 3x²y + 3xy² + 2y³ = (x + 2y) (x² + xy + y²)

10. 1 + 9x + 27x² + 28x³

Solution:

Given 1 + 9x + 27x² + 28x³

= 1 + 9x + 27x² + 27x³ + x³ .

= (1)³ + 3.(1 )² .3x + 3.1. (3x)² + (3x)³ + x³

= (1 + 3x)³ + (x)³

= (1 + 3x + x) {(1 + 3x)² – (1 + 3x) x + (x)²}

= (1 + 4x) (1 + 6x + 9x² – x – 3x² + x²)

= (1 + 4x) (1 + 5x + 7x²)

1 + 9x + 27x² + 28x³  = (1 + 4x) (1 + 5x + 7x²)

11.  x³ – 9y³ – 3xy (x – y)

Solution:

Given x³ – 9y³ – 3xy (x – y)

= x³ – 9y³ – 3x²y + 3xy²

= x2 – 3x²y + 3xy² – y³ – 8y³

= (x – y)³– (2y)³

= (x – y – 2y) {(x-y)² + (x-y) 2y + (2y)²}

= (x-3y) (x² – 2xy + y² + 2xy – 2y² + 4y²)

= (x – 3y) (x² + 3y²)

x³ – 9y³ – 3xy (x – y) = (x – 3y) (x² + 3y²)

12. 8-a³ + 3a²b – 3ab² + b³

Solution:

Given 8-a³ + 3a²b – 3ab² + b³

= 8- (a³ – 3a²b + 3ab² – b³)

= (2)³ – (a – b)³

= {2 – (a -b)}{(2)² + 2(a – b) + (a-b)²}

= (2 – a + b) (4 + 2a – 2b + a² – 2ab + b²)

8-a³ + 3a²b – 3ab² + b³ = (2 – a + b) (4 + 2a – 2b + a² – 2ab + b²)

13. x⁶ + 3x⁴b² + 3x²b⁴ + b⁶ + a³b³

Solution:

Given x⁶ + 3x⁴b² + 3x²b⁴ + b⁶ + a³b³

= (x²)³+ 3.(x²)².b² + 3.x².(b²)² + (b²)³ + a³b³

= (x² + b²)³ + (ab)³

= (x² + b² + ab) {(x² + b²)² – (x² + b²) ab + (ab)²}

= (x² + b² + ab) (x⁴ + 2x²b² + b⁴ – x²ab – ab³ + a²b²)

= (x² + ab + b²) (x⁴ + 2x²b² – abx² + a²b² – ab³ + b⁴)

x⁶ + 3x⁴b² + 3x²b⁴ + b⁶ + a³b³ = (x² + ab + b²) (x⁴ + 2x²b² – abx² + a²b² – ab³ + b⁴)

14.  x⁶+27

Solution:

Given x⁶+27

= (x²)³ + (3)³

= (x² + 3) {(x²)² – x².3 + (3)²}

= (x² + 3) (x⁴ – 3x² +9)

x⁶+27 = (x² + 3) (x⁴ – 3x² +9)

15.  x⁶ – y⁶

Solution:

Given x⁶ – y⁶

= (x³)² – (y³)² = (x³ + y³) (x³ – y³)

= (x + y) (x² – xy + y²) (x – y) (x² + xy + y²)

= (x + y) (x – y) (x² – xy + y²) (x² + xy + y²)

x⁶ – y⁶ = (x + y) (x – y) (x² – xy + y²) (x² + xy + y²)

16.  x¹²– y

Solution:

Given x¹²– y

= (x⁶)² – (y⁶)²

= (x⁶ + y⁶) (x⁶ – y⁶)

= {(x²)³+(y²)³} {(x³)² – (y³)²}

= (x² + y²) {(x²)²– x².y² + (y²)²} (x³ + y³) (x³ – y³)

= (x²+ y²) (x⁴– x²y² + y⁴) (x + y).(x²– xy + y²) (x-y) (x² + xy + y²)

= (x + y) (x – y) (x² + y²) (x² – xy + y²) (x² + xy + y²) (x⁴ – x²y² + y⁴)

x¹²– y = (x + y) (x – y) (x² + y²) (x² – xy + y²) (x² + xy + y²) (x⁴ – x²y² + y⁴)

17. m³ – n³ – m (m² – n²) + n(m – n)

Solution:

Given m³ – n³ – m (m² – n²) + n(m – n)

= (m-n) (m²+mn+n²) -m(m+n) (m-n) +n(m-n)(m-n)

= (m-n) (ref + rpfi + rf – ryf – rprfi + mn -rf) –

= (m-n) mn = mn (m-n)

m³ – n³ – m (m² – n²) + n(m – n) = mn (m-n)