NEET Physics Motion In A Plane Notes

Motion In A Plane

Projectile Motion

A body which is in flight through the atmosphere, but if it is moving only under the influence of gravity, then it is a projectile.

Types of Projectile Motion

1. Oblique projectile
2. Horizontal projectile

Oblique Projectile

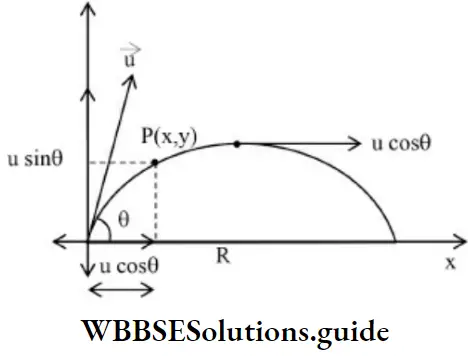
1. The equation of the trajectory of the projectile is given by:

⇒ \(y=(\tan \theta) x-\left(\frac{g}{2 u^2 \cos ^2 \theta}\right) x^2\)

The above equation is similar to the equation of a parabola.

y= ax- bx²

i.e trajectroy of a projectile is a parabola

Read And Learn More: NEET Physics Notes

The equation of oblique projectile can also be written as,

⇒ \(y=x \tan \theta\left[1-\frac{x}{R}\right]\)

Whereas, R= \(\frac{\mathrm{u}^2 \sin 2 \theta}{\mathrm{g}}\)

2. Velocity of the projectile at any point on its trajectory is given by:

⇒ \(\vec{v}=v_x \hat{i}+v_y \hat{j}\)

⇒\(\mathrm{V}=\sqrt{\mathrm{V}_{\mathrm{x}}^2+\mathrm{V}_{\mathrm{y}}^2}\)

⇒ \(\mathrm{v}_{\mathrm{x}}=\mathrm{u} \cos \theta\)

⇒  \(\mathrm{v}_{\mathrm{y}}=\mathrm{u} \sin \theta-\mathrm{gt}\)

v= \(\sqrt{\left(u^2 \cos ^2 \theta\right)+(u \sin \theta-g t)^2}\)

v= \(\sqrt{u^2+g^2 t^2-2 u g t \sin \theta}\)

The direction of instantaneous velocity is given by, \(\tan \alpha=\frac{v_y}{v_x}\)

3. Change in velocity of the projectile between point of projection and highest point is given by:

⇒ \(\Delta \overrightarrow{\mathrm{v}}=\overrightarrow{\mathrm{v}}_{\mathrm{f}}-\overrightarrow{\mathrm{v}}_{\mathrm{i}}=-\mathrm{u} \sin \theta \hat{\mathrm{j}}\)

Change in velocity during complete oblique projectile motion is given by

⇒ \(\Delta \overrightarrow{\mathrm{v}}=-2 \mathrm{u} \sin \theta \hat{\mathrm{i}}\)

4. The change in momentum of the projectile between the point of projection and the highest point is:

⇒ \(\Delta \overrightarrow{\mathrm{P}}=\overrightarrow{\mathrm{P}}_{\mathrm{f}}-\overrightarrow{\mathrm{P}}_{\mathrm{i}}=-\mathrm{mu} \sin \theta \hat{\mathrm{j}}\)

The change in momentum of the projectile during complete oblique projectile motion is given by,

⇒ \(\Delta \overrightarrow{\mathrm{P}}=-2 m u \sin \theta \hat{\mathrm{i}}\)

5. Angular momentum of the projectile at highest point of trajectory about the point of projection is given by:

L = \(m v r\left(r=H=\frac{u^2 \sin ^2 \theta}{2 g}\right)\)

∴  L= \(\mathrm{m}(\mathrm{u} \cos \theta)\left(\frac{\mathrm{u}^2 \sin ^2 \theta}{2 \mathrm{~g}}\right)\)

∴ L= \(=\frac{m u^3 \cos \theta \sin ^2 \theta}{2 \mathrm{~g}}\)

6.  Time of maximum height:

⇒ \(\mathrm{t}_{\max }=\frac{\mathrm{u} \sin \theta}{\mathrm{g}}\)

7. Time of flight:

⇒ \(\mathrm{T}=2 \mathrm{t}_{\max }=\frac{2 \mathrm{u} \sin \theta}{\mathrm{g}}\) Or \(\mathrm{T}=\frac{2 \mathrm{u}_{\mathrm{y}}}{\mathrm{g}}\)

8. Horizontal range:

It is the horizontal distance traveled by the projectile during its flight.

R = (u_x) (T)

R= \((\mathrm{u} \cos \theta) \frac{2 \mathrm{u} \sin \theta}{\mathrm{g}}\)

r = \(\mathrm{R}\frac{\mathrm{u}^2}{\mathrm{~g}} \sin 2 \theta\)

R= \(\frac{2}{\mathrm{~g}}\left(\mathrm{u}_{\mathrm{x}}\right)\left(\mathrm{u}_{\mathrm{y}}\right)\)

Where u_x = u cos θ: u = u_y sin θ

For complementary angles of projection θ and 90 – θ range is the same.
Or
For angles of projection θ₁ =(45 – α) and θ₂ = (45 + α)) range will be the same.

If the angle of projection is 450, the range is maximum.

⇒ \(\mathrm{R}_{\max }=\frac{\mathrm{u}^2}{\mathrm{~g}}\)

9. Maximum height of a projectile is given by:

⇒ \(\mathrm{H}=\frac{\mathrm{u}^2 \sin ^2 \theta}{2 \mathrm{~g}}\)

Or \(\mathrm{H}=\frac{\mathrm{u}_{\mathrm{y}}^2}{2 \mathrm{~g}}\)

⇒ \(\mathrm{H}_{\max }=\frac{\mathrm{u}^2}{2 \mathrm{~g}}\)

Or

⇒  \(\frac{\mathrm{H}}{\mathrm{T}^2}=\frac{\mathrm{g}}{8}\)

Energy of Projectile

When a projectile moves upwards through its trajectory its kinetic energy will decrease and potential energy will increase, but the total energy always remains constant.

Say a body of mass ‘m’ is projected obliquely with angle of projection ‘0’.

 

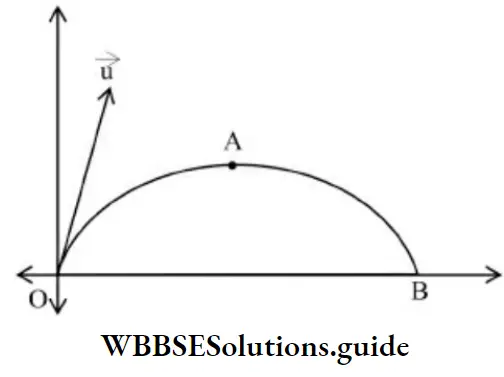
At’O’:

⇒ \(\mathrm{K} \cdot \mathrm{E}=\frac{1}{2} \mathrm{mu}^2\)

P.E = 0

Total energy E = ½ mu²

At’A’:

⇒ \(\mathrm{K} \cdot \mathrm{E} .=\frac{1}{2} \mathrm{mu}^2 \cos ^2 \theta\)

P.E = mgh

P.E = \(m g \frac{u^2 \sin ^2 \theta}{2 g}\)

P.E= \(\frac{1}{2} m u^2 \sin ^2 \theta\)

Total energy

E= K.E + P.E = \(=\frac{1}{2} m u^2 \cos ^2 \theta+\frac{1}{2} m u^2 \sin ^2 \theta\)

⇒ \(\mathrm{E}=\frac{1}{2} \mathrm{mu}^2\)

Similarly, at B,

⇒ \(\mathrm{E}=\frac{1}{2} \mathrm{mu}^2\)

Horizontal Projectile

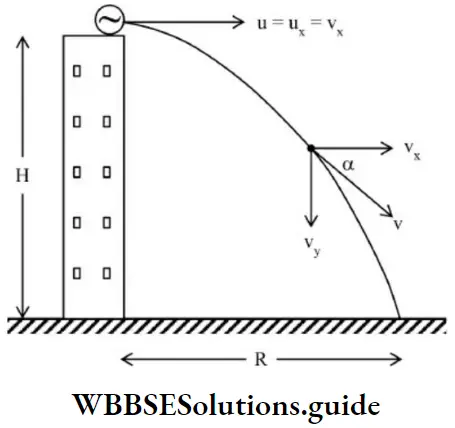
Suppose a body of mass ‘m’ is thrown horizontally with speed ‘u’ from the top of a tower.

The magnitude of velocity at any point on the trajectory is given by,

v= \(\sqrt{v_x^2+v_y^2}\)

Where, v_x = u and v_y = gt, tan \(\frac{v_y}{v_x}=\frac{g t}{u}\)

Time taken by the ball to reach the ground is given by,

⇒ \(t=\sqrt{\frac{2 H}{g}}\)

Range:

u_x × t

⇒ \(\mathrm{R}=\mathrm{u} \sqrt{\frac{2 \mathrm{H}}{\mathrm{g}}}\)

Riverboat problem

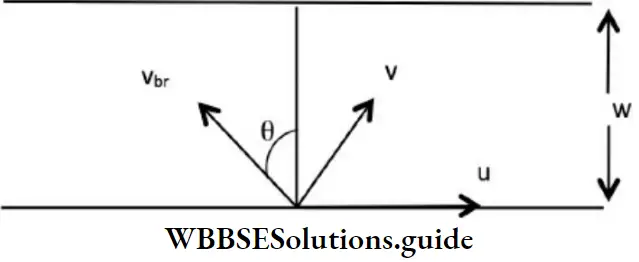
V_br velocity of the boat w.r.to river

V is the velocity of the boat w.r.to ground

u is the velocity of the river

1. To cross the river straight, we should row the boat by making an angle with the upstream where 0 is the angle made by with the shortest distance (which is width of the river)

⇒ \(\sin \theta=\frac{u}{v_{b r}}\)

The time taken to cross the river is given by

2. To cross the river in the shortest time, we should row the boat perpendicular to bank. The time taken in this case is,

⇒ \(t_{\min }=\frac{w}{v_{b r}}\)

3. The horizontal distance travelled by the boat w.r.to starting point is,

⇒  \(xu \times t_{\min }\)

Uniform Circular Motion

If a body is moving in a circular path with constant speed then the body is said to be in uniform circular motion.

Expression for centripetal acceleration is given by,

⇒\(a_c=\frac{v^2}{r}\)

Or, \(a_c=\omega^2 r\) (because ω = rω) where co is the angular velocity.

Expression for centripetal force is

⇒ \(F_c=\frac{m v^2}{r}=m \omega^2 r\)